Inverse Variation // Lesson 11-1 [Glencoe Texas Algebra 1]

When the ratio between two terms remains constant, we call it direct variation. We typically solve direct variation scenarios by setting up proportions and then working with cross-products. But when instead it is the product between two terms that remains constant, we call that inverse variation.

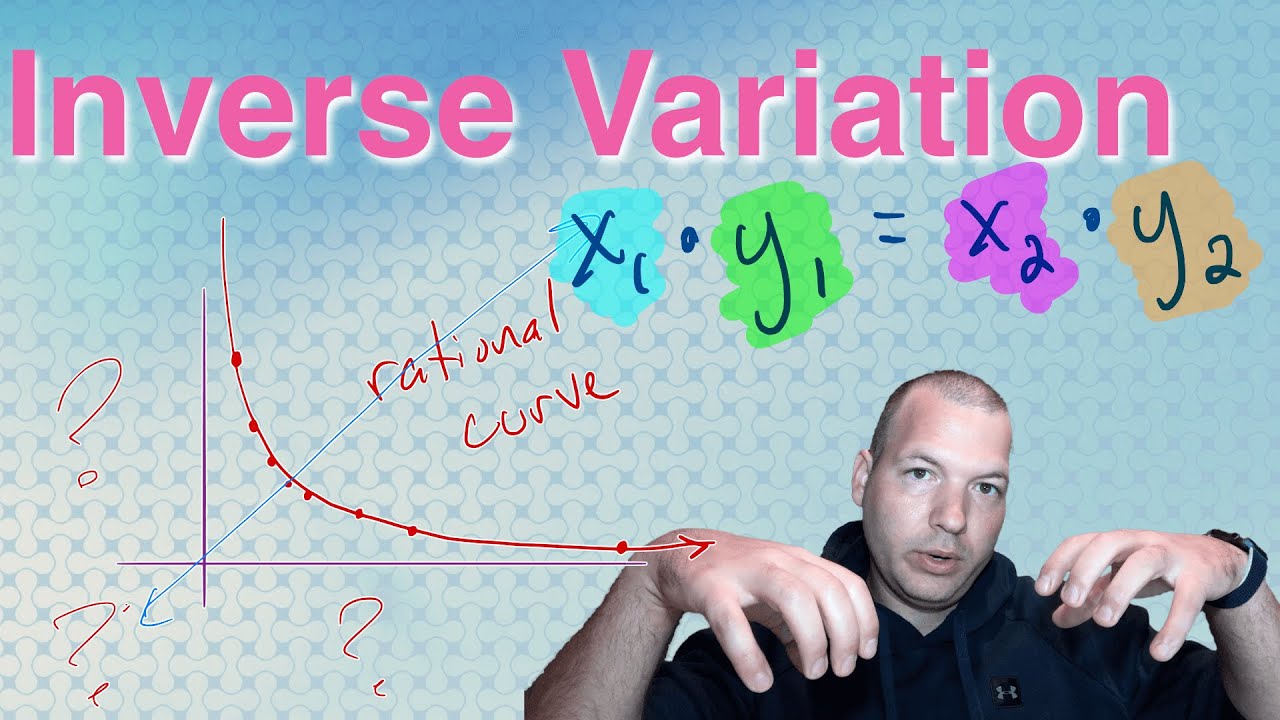
Although it is possible to set up proportions that will solve inverse variation scenarios, it's much better to take advantage of the constant products using the formula x₁×y₁=x₂×y₂. In other words, the product of your first (x,y) coordinate pair must be the same as the product of the second (x,y) coordinate pair (or any other set of coordinate pairs for that matter).

When graphing inverse variation scenarios, you should end up with a distinctive curve that has symmetricality across the line y=x. This is due to the fact that multiplication is commutative, which means order does not matter. For the graph, this means that both the points (4,3) and (3,4), for example, will be on the graph. You can use this symmetricality to simplify the graphing process.

Follow Tim Ricchuiti:

Watch more Math Videos: