filmov
tv
A Nice Factorable Quintic | Problem 215

Показать описание
▶ Greetings, everyone! Welcome to @aplusbi 🧡🤩💗
This channel is dedicated to the fascinating realm of Complex Numbers. I trust you'll find the content I'm about to share quite enjoyable. My initial plan is to kick things off with informative lectures on Complex Numbers, followed by a diverse range of problem-solving videos.
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you. ❤️ ❤️ ❤️
❤️ This is Problem 215 on this channel!!! ❤️
🤩 Don't forget to SUBSCRIBE, hit that NOTIFICATION bell and stay tuned for upcoming videos!!!
▶ The world of Complex Numbers is truly captivating, and I hope you share the same enthusiasm! Come along with me as we embark on this exploration of Complex Numbers. Feel free to share your thoughts on the channel and the videos at any time.
▶ MY CHANNELS
Future channels: TBD
▶ EQUIPMENT and SOFTWARE
Camera: none
Microphone: Blue Yeti USB Microphone
Device: iPad and apple pencil
Apps and Web Tools: Notability, Google Docs, Canva, Desmos
LINKS
#complexnumbers #aplusbi #jeeadvanced #jee #complexanalysis #complex #jeemains
via @YouTube @Apple @Desmos @GoogleDocs @canva @NotabilityApp @geogebra
This channel is dedicated to the fascinating realm of Complex Numbers. I trust you'll find the content I'm about to share quite enjoyable. My initial plan is to kick things off with informative lectures on Complex Numbers, followed by a diverse range of problem-solving videos.
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you. ❤️ ❤️ ❤️
❤️ This is Problem 215 on this channel!!! ❤️
🤩 Don't forget to SUBSCRIBE, hit that NOTIFICATION bell and stay tuned for upcoming videos!!!
▶ The world of Complex Numbers is truly captivating, and I hope you share the same enthusiasm! Come along with me as we embark on this exploration of Complex Numbers. Feel free to share your thoughts on the channel and the videos at any time.
▶ MY CHANNELS
Future channels: TBD
▶ EQUIPMENT and SOFTWARE
Camera: none
Microphone: Blue Yeti USB Microphone
Device: iPad and apple pencil
Apps and Web Tools: Notability, Google Docs, Canva, Desmos
LINKS
#complexnumbers #aplusbi #jeeadvanced #jee #complexanalysis #complex #jeemains
via @YouTube @Apple @Desmos @GoogleDocs @canva @NotabilityApp @geogebra
A Nice Factorable Quintic | Problem 215
Solving A Nice Quintic System
Factoring A Quintic | Polynomials #maths
Factoring A Quintic vol.2 | Polynomials #maths
Solving a Nice Homemade Quintic | #factoring #algebra
A nice quintic equation. | How to solve it? | You should know the method.
EXTREME quintic equation! (very tiring)
Factoring x^5+x+1 | A Quintic
Quintic Equation
Factoring a Quintic | Challenging Algebra
solving a 6-term quintic equation
How to Solve this Quintic Polynomial Related Problem?
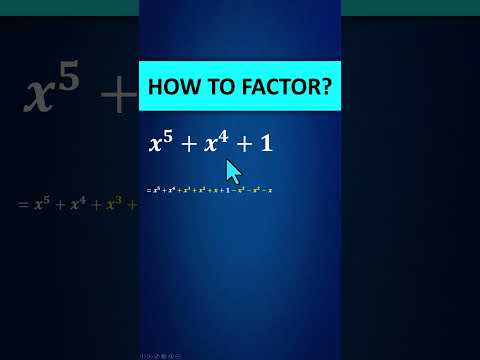
Factor this quintic polynomial : x⁵+x⁴+1
Finding the only real solution to the quintic equation x^5+x^4+1=0
Factoring a Quintic Sum | Algebra
Solving A Quintic | Polynomial Equations #maths
Factoring A Quintic Trinominal #algebra #fast #mathematics #maths #shorts #challenge #equation
x^5+x^4+1 How to Factor Quintic Polynomial?
Can You Factories A Quintic Trinomial in Just 40 Seconds? | #Shorts
Solving A Quintic Without Using The Quintic Formula
How To Solve A Quintic Equation | Algebra | Math Olympiad
Powerful Quintic Equation Here || Learn How to solve for all the values of m ●■|| Math Olympiad
My First Quintic Equation
Can You Solve a Quintic with Complex Roots? No? Then Watch!
Комментарии
 0:09:33
0:09:33
 0:08:12
0:08:12
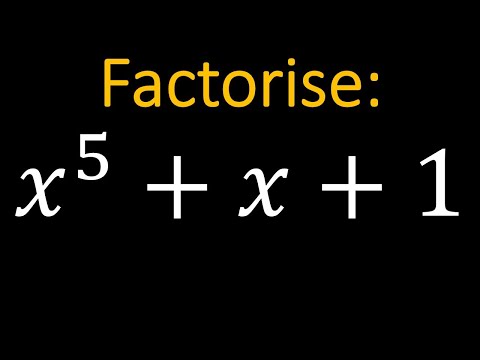 0:01:55
0:01:55
 0:02:08
0:02:08
 0:09:02
0:09:02
 0:06:38
0:06:38
 0:31:27
0:31:27
 0:00:52
0:00:52
 0:00:58
0:00:58
 0:12:52
0:12:52
 0:04:24
0:04:24
 0:04:43
0:04:43
 0:01:00
0:01:00
 0:00:26
0:00:26
 0:10:12
0:10:12
 0:03:58
0:03:58
 0:00:27
0:00:27
 0:00:54
0:00:54
 0:00:55
0:00:55
 0:12:03
0:12:03
 0:10:42
0:10:42
 0:09:56
0:09:56
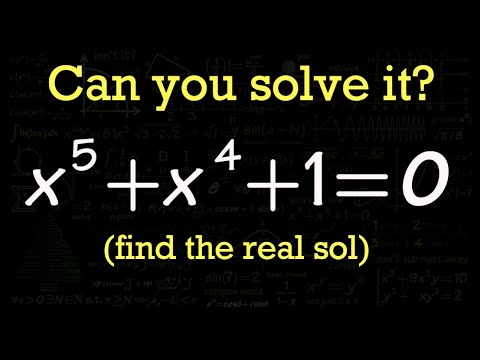 0:10:29
0:10:29
 0:07:19
0:07:19