filmov
tv
Linear Algebra, Krylov subspace Methods, and Multigrid Solvers for the Discovery of New Physics

Показать описание
In this talk, the speakers will give an overview of the numerical methods used in Lattice Quantum Chromodynamics for the study of properties of quarks and gluons inside nuclei. The speakers will focus on the approaches used for solving linear systems of massive dimensions that arise from the discretization of the Dirac operator. These are namely iterative Krylov-subspace solvers preconditioned by algebraic multigrid methods, which are state-of-the-art in the field. After introducing them, the speakers will present the developments that they have carried out under the PRACE project LyNCs in collaboration with Inria and LRZ.
This seminar was given by Jacob Finkenrath and Simone Bacchio..
Jacob Finkenrath received his PhD, Dr. rer. nat., in High Energy Physics in 2015, awarded by the University of Wuppertal. Since 2015 he has worked at CaSToRC as a Computational Scientist. His research is focused on Lattice Quantum Chromodynamics where he works on algorithm development, large-scale simulations and hadron spectroscopy. He is the coordinator of the academic activities of the National Competence Center in High-Performance Computing.
Simone Bacchio is a Computational Scientist at the Cyprus Institute under The National Competence Center in High-Performance Computing. He has received a joint doctorate in Computational Physics and Applied Mathematics, awarded by the University of Cyprus and University of Wuppertal. His major research interests are algorithms, multigrid methods, HPC software development and Lattice Quantum Chromodynamics.
This seminar was given by Jacob Finkenrath and Simone Bacchio..
Jacob Finkenrath received his PhD, Dr. rer. nat., in High Energy Physics in 2015, awarded by the University of Wuppertal. Since 2015 he has worked at CaSToRC as a Computational Scientist. His research is focused on Lattice Quantum Chromodynamics where he works on algorithm development, large-scale simulations and hadron spectroscopy. He is the coordinator of the academic activities of the National Competence Center in High-Performance Computing.
Simone Bacchio is a Computational Scientist at the Cyprus Institute under The National Competence Center in High-Performance Computing. He has received a joint doctorate in Computational Physics and Applied Mathematics, awarded by the University of Cyprus and University of Wuppertal. His major research interests are algorithms, multigrid methods, HPC software development and Lattice Quantum Chromodynamics.
 0:12:46
0:12:46
 0:03:50
0:03:50
 0:58:37
0:58:37
 0:36:19
0:36:19
 0:04:05
0:04:05
 0:27:45
0:27:45
 0:49:11
0:49:11
 0:29:23
0:29:23
 0:07:25
0:07:25
 0:05:41
0:05:41
 2:59:56
2:59:56
 0:02:06
0:02:06
 0:20:01
0:20:01
 0:10:11
0:10:11
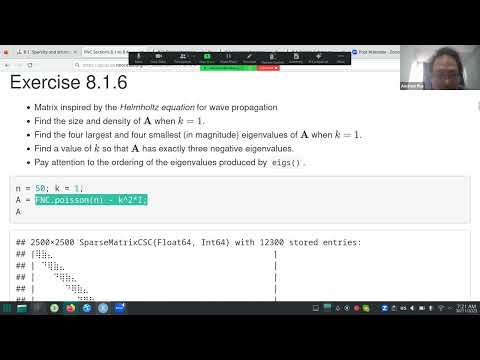 0:58:06
0:58:06
 0:33:40
0:33:40
 0:33:03
0:33:03
 0:12:01
0:12:01
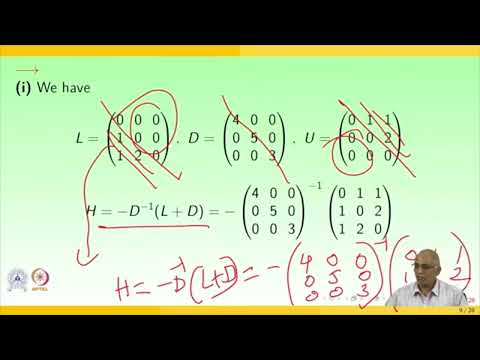 0:24:51
0:24:51
 0:40:46
0:40:46
 0:01:24
0:01:24
 0:08:22
0:08:22
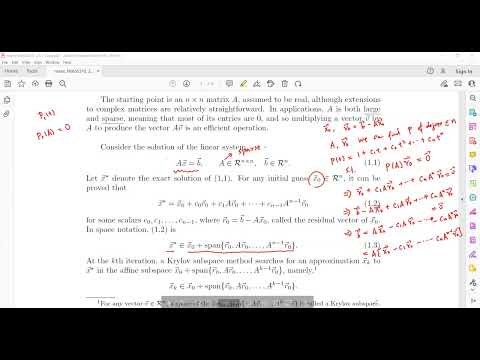 0:47:52
0:47:52
 0:14:53
0:14:53