Linear Algebra 5.2.2 The Characteristic Equation

Показать описание

Kimberly Brehm

Рекомендации по теме

Комментарии

THANK YOU SO MUCH I THOUGHT MY TEACHER WAS JUST LOOKING AT A MATRIX AND MAKING UP NUMBERS!!! NOW I KNOW!!!

crabcakeenthusiast

شكرا شكرا
you make things easy, enjoyable THANK YOU from Egypt

AhMedKhaled-omly

on the practice, did you mean to write lamda^3 for the last term? In the problem is was to the first power.

matt

Hi, can you please make a video for section 5.3 and 5.4? Thank you!

marthabarranco

But the equation isn’t lambda ^ 3, it’s just lambda. was there a typo in the question maybe ?

ozlempolat

You did not write out the last polynomial (in pink) correctly, you left out the ^3 on the 3(lambda).

grapplegamer

you're a life saver thanks so much for linear algebra video

bisrataraya

Ok so we can only find characteristic equation for square matrix only?

debjitkhaskel

5:57 how did you calculate it so quickly

muhammadabdullahriazbhatti

But what about the complicated ones? There are more concepts right??

saminyasar

Can you add the sections in-between 5.2 and 6.1 please 🙏

pringlegodx

Characteristic equation can be calculated without determinant
We can use trace and multiplication
a_{n}=1
a_{k} = -1/(n-k)sum(a_{j+k} tr(A^{j}), j=1..n-k)
This can be proved using fact that tr(A^{m})=sum(λ_{k}^{m}, k=1..n)
after this we will get sum of powers which is symmetric function so Newton-Girard formulas will be helpful
Vieta formula also will be useful to express elementary symmetric polynomials in terms of coefficients
The other method is using Cayley-Hamilton theorem
We pick randomly chosen vector and plug in matrix into characteristic equation
this will lead us to the system of equations for coefficients

holyshit

Linear Algebra 5.2.2 The Characteristic Equation

Linear Algebra 5.2.2 The Characteristic Equation

Linear Algebra Ch 5.2 Characteristic equation # 2

5.2 Characteristic Equation

[Linear Algebra] The Characteristic Equation and Eigenvalues

Eigenvectors and eigenvalues | Chapter 14, Essence of linear algebra

Linear Algebra - Lecture 34 - The Characteristic Equation

Linear Algebra -- Characteristic Polynomials and Eigenvalues of 3x3 Matrix

Intro to Linear Algebra - Characteristic Equation

A quick trick for computing eigenvalues | Chapter 15, Essence of linear algebra

Finding Eigenvalues and Eigenvectors

Find the characteristic equation & roots of the matrix example(PART-3)

Characteristic Polynomial

Find Eigenvalues and Eigenvectors of a 2x2 Matrix

Eigen values and Eigen vectors | 2 x 2 matrix | Problem Solved | Mathspedia |

Linear Algebra 5.2.2 Similar Matrices

Characteristic Equation - Linear Algebra - F3

MATH 2120 Characteristic Polynomial of a 2 by 2 Matrix

Linear Algebra Ch 5.2 Characteristic equation # 1

Linear Algebra 5.2.1 Determinants and the IMT

Linear Algebra - Section 5.2 - The Characteristic Equation

Linear Algebra 5.1.1 Eigenvectors and Eigenvalues

Matrices and Polynomials

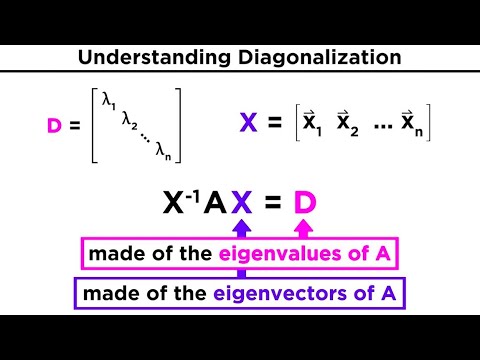
Diagonalization

Linear Algebra 5.2.1 Characteristic Polynomial