filmov
tv
Find the SUM of the interior angles of an OCTAGON. Must Know Geometry Formula!

Показать описание
Help with Middle and High School Math
Test Prep for High School Math, College Math, Teacher Certification Math and More!
Popular Math Courses:
Math Foundations
Math Skills Rebuilder Course:
Pre-Algebra
Algebra
Geometry
Algebra 2
Pre-Calculus
• MIDDLE & HIGH SCHOOL MATH
• HOMESCHOOL MATH
• COLLEGE MATH
• TEST PREP MATH
• TEACHER CERTIFICATION TEST MATH
How to find the sum of 3 numbers
What is the sum of 3/4 and 2/3?
Math tutorial for determining the sum of an arithmetic series
Finding the sum or an arithmetic series using summation notation
Find the sum of the following fractions
How to find the sum of a mixed number and a fraction
Find the Sum of the Series SUM((2^n + 1)/3^n)
Finding The Sum of an Infinite Geometric Series
How to find sum of 1st 'n' natural numbers…!!#shorts
Sum and Difference
Find the sum of & find the difference between
Class 6 - Exercise 6.2 - Q 4 | Find the sum of following
Find the Sum of the First 30 Terms of the Arithmetic Sequence 3, 7, 11, 15, ...
Sum of an Arithmetic Sequence: Find the sum of multiples
Find the Sum || Class -5 || Chapter Fraction || Farha Zakir
Finding the sum of a series arithmetic
Find the Sum of the Series by using Maclaurin Series
Learn how to determine the sum of a geometric finite series
How to Find the Sum of Functions | Algebra, Adding Functions, Sums of Functions
How To Find The Sum of a Geometric Series
Find the sum of first n squares, difference equation approach, (ft. Max!)
Calculus II: Example finding the sum of a convergent series
Find the Sum of the Angles of a Decagon
Finding The Sum of a Finite Geometric Series
Комментарии
 0:01:04
0:01:04
 0:03:05
0:03:05
 0:06:37
0:06:37
 0:03:53
0:03:53
 0:03:20
0:03:20
 0:01:55
0:01:55
 0:03:57
0:03:57
 0:19:50
0:19:50
 0:00:21
0:00:21
 0:01:36
0:01:36
 0:05:44
0:05:44
 0:04:03
0:04:03
 0:03:38
0:03:38
 0:05:48
0:05:48
 0:04:40
0:04:40
 0:04:58
0:04:58
 0:03:11
0:03:11
 0:02:42
0:02:42
 0:04:28
0:04:28
 0:05:14
0:05:14
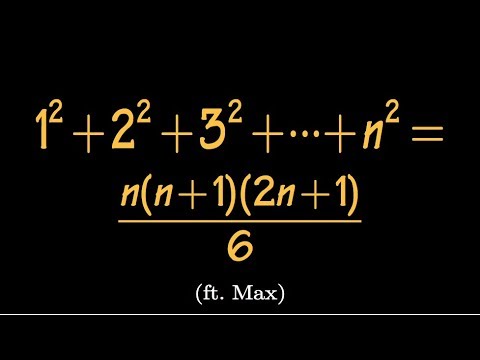 0:11:19
0:11:19
 0:06:41
0:06:41
 0:00:58
0:00:58
 0:12:59
0:12:59