Differential Equations, Lecture 4.9: Variation of parameters for systems

Differential Equations, Lecture 4.9: Variation of parameters for systems.

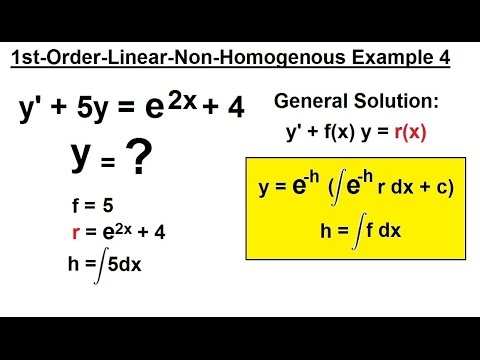
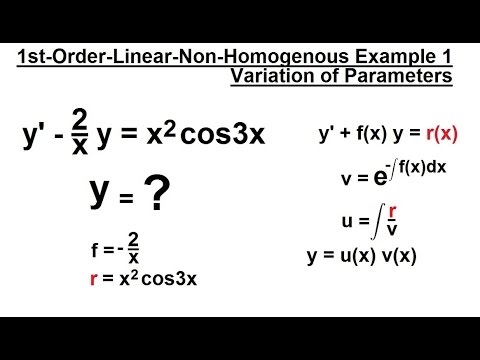
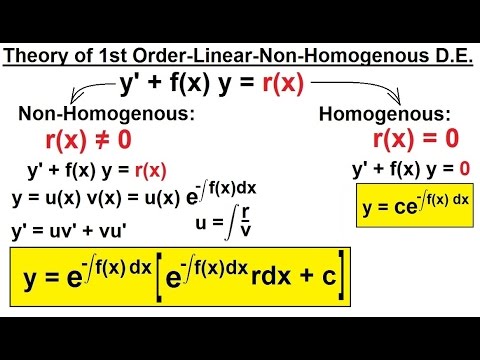
This is the third lecture on the variation of parameters method, which is a "last-resort" but surefire way to find a particular solution. Previous, we've seen it for 1st order systems, where we assume that y_p(t) = v(t)y_1(t), and for 2nd order systems, where we assume that y_p(t) = v_1(t)y_1(t)+v_2(t)y_2(t). In this lecture, we assume that there is a solution of the form x_p(t)=X_h(t)v(t), where X_h(t) is the 2x2 matrix where the columns solve the homogeneous ODE, and v(t) is an unknown 2-dimensional vector. This new method for systems ends up being a generalization of both the previous methods that we've learned, i.e., they end up as a special cases.