filmov
tv
🔵24 - D Operator Method for Solving First Order Linear Differential Equations
Показать описание
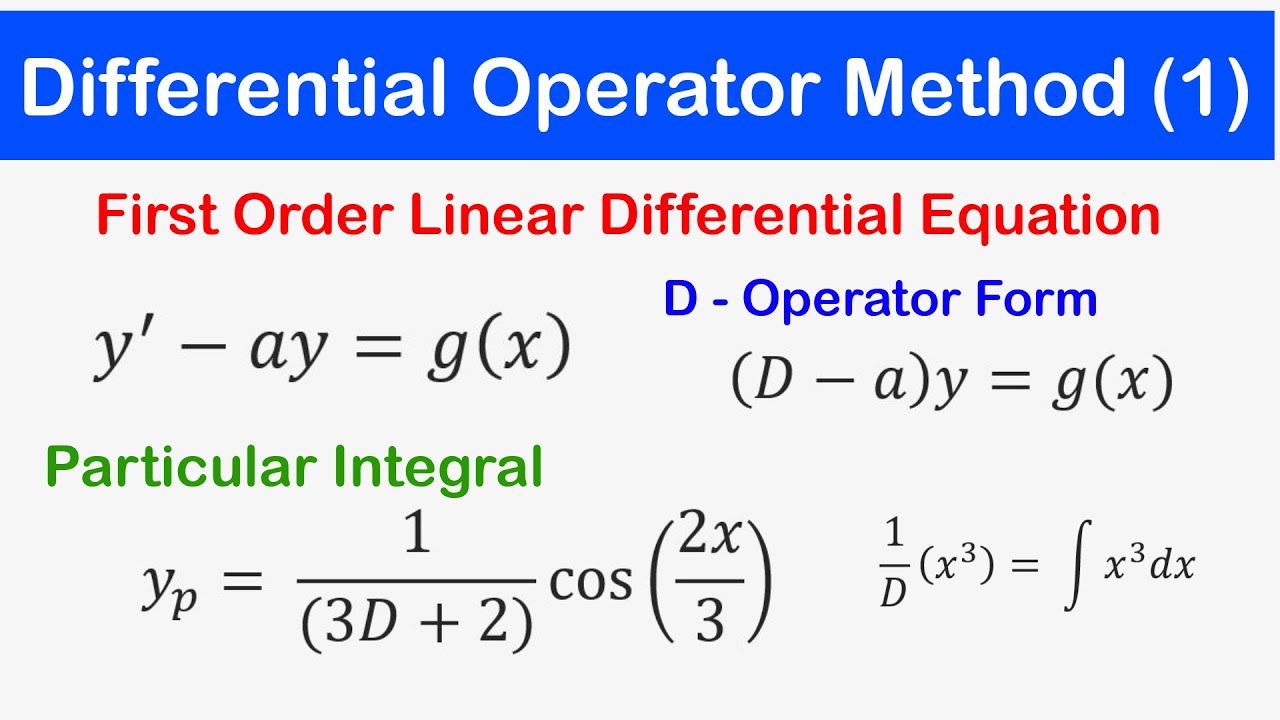
In this lesson we shall learn how to solve the general solution of a linear differential equation using the d operator method. The d operator is an effective way of solving d.e's where the coefficients need to be constants.
For each form of G(x), the solution process is quite different.
In this lesson we shall consider variety of cases and examples i.e
for g(x) to be a:
1. Exponential Function
2. Sine or cosine function
3. Polynomial function
4. sum of functions
5. product of functions
00:00 - Introduction
04:46 - First Order Linear D.E
06:10 - Ex 1: Exponential Function
10:14 - Ex 1: (Alternative Approach)
15:57 - Ex 2: sine or cosine function
22:18 - Ex 3: sine or cosine function
29:53 - Ex 4: sine or cosine function
Playlists on various Course
1. Applied Electricity
2. Linear Algebra / Math 151
3. Basic Mechanics
4. Calculus with Analysis / Calculus 1 / Math 152
5. Differential Equations / Math 251
6. Electric Circuit Theory / Circuit Design
Make sure to watch till the end.
Like, share, and subscribe.
Thank you.
For each form of G(x), the solution process is quite different.
In this lesson we shall consider variety of cases and examples i.e
for g(x) to be a:
1. Exponential Function
2. Sine or cosine function
3. Polynomial function
4. sum of functions
5. product of functions
00:00 - Introduction
04:46 - First Order Linear D.E
06:10 - Ex 1: Exponential Function
10:14 - Ex 1: (Alternative Approach)
15:57 - Ex 2: sine or cosine function
22:18 - Ex 3: sine or cosine function
29:53 - Ex 4: sine or cosine function
Playlists on various Course
1. Applied Electricity
2. Linear Algebra / Math 151
3. Basic Mechanics
4. Calculus with Analysis / Calculus 1 / Math 152
5. Differential Equations / Math 251
6. Electric Circuit Theory / Circuit Design
Make sure to watch till the end.
Like, share, and subscribe.
Thank you.
0:39:06
🔵24 - D Operator Method for Solving First Order Linear Differential Equations
0:18:39
🔵25 - D Operator Method for Solving Second Order Linear Differential Equations
0:07:11
24-D Operator Method solution of Differential Equations(𝑦𝑃𝐼 =𝐶𝑜𝑚𝑏𝑖𝑛𝑎𝑡𝑖𝑜𝑛)...
1:13:26
Solving Differential Equations using operator D method
0:42:00
Finding Particular Integral (D-Operator Method)
0:05:12
D-Notation and Determine a Linear Differential Operator of a Linear Differential Equation
0:31:00
D-operator method
0:50:38
Math 24 Section 8.1: Operator Method
0:15:06
Math 24 Section 4.5: Differential Operators
0:00:55
Write the Differential Equations using the Differential Operator D: y'' - 5y = x^2 - 2x
0:15:45
D-operator method for solving systems of linear differential equation.
0:56:59
D Operator Method for System of Differential Equations.
1:37:57
Lec07 | D-Operator Method in 1 Shot | 2nd Order Linear Differential Equation | Differential Calculus
0:38:43
How to use Inverse D-operators method in solving non-homogeneous linear ODEs of the 2nd order.
0:26:22
Differential Equations: Method of Undetermined Coefficients - Annihilator Approach
0:38:06
Lecture # 30 || D-Operator Method (CASE II) || Non-Homogenous Diff. Eq. ||Particular Solution || ODE
0:10:35
Using Differential Operators
0:01:08
Modulus Operator - CS101 - Udacity
0:08:39
Solving systems of DE - Elimination method with Operator - Made Easy
0:46:00
(DE24) Fractional-Order Differential Operators
0:33:55
Differential Equations | D-OPERATOR METHODS & METHOD OF UNDETERMINED COEFFICIENTS
0:12:43
D-operator method
0:33:16
Lecture #33||D-Operator Method ||Exponential Shift Theorem (Part II)||Non-Homogenous Diff. Eq. ||ODE
0:10:34
How to find the annihilator operator of a function
Комментарии