filmov
tv
Queuing theory and Poisson process
Показать описание
Queuing theory is indispensable, but here is an introduction to the simplest queuing model - an M/M/1 queue. Also included is the discussion on Poisson process, which is the underlying assumption for the M/M/1 queue.
To me, this is mainly a "prequel" which serves as a prerequisite for the next video, even though the next video is not as long.
queuingmodelM/M/1
and follow the instructions on the website. If you can't enter the website, watch the latest video! It always changes when a new video is up.
Sources:
Different queues:
The (transient) solution: Computer Networks and Systems. New York, NY: Springer New York. p. 72 (uses moment-generating function and Laplace transforms); for more details, see Gross, D. and Harris, C.M., Fundamentals of Queueing Theory, Wiley, New York, 1974, 1985. (Section 3.11.2)
Other related sources:
Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:
If you want to know more interesting Mathematics, stay tuned for the next video!
SUBSCRIBE and see you in the next video!
If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I use PowerPoint, GeoGebra, and (sometimes) Mathematica to produce the videos.
Social media:
For my contact email, check my About page on a PC.
See you next time!
To me, this is mainly a "prequel" which serves as a prerequisite for the next video, even though the next video is not as long.
queuingmodelM/M/1
and follow the instructions on the website. If you can't enter the website, watch the latest video! It always changes when a new video is up.
Sources:
Different queues:
The (transient) solution: Computer Networks and Systems. New York, NY: Springer New York. p. 72 (uses moment-generating function and Laplace transforms); for more details, see Gross, D. and Harris, C.M., Fundamentals of Queueing Theory, Wiley, New York, 1974, 1985. (Section 3.11.2)
Other related sources:
Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:
If you want to know more interesting Mathematics, stay tuned for the next video!
SUBSCRIBE and see you in the next video!
If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I use PowerPoint, GeoGebra, and (sometimes) Mathematica to produce the videos.
Social media:
For my contact email, check my About page on a PC.
See you next time!
Комментарии
 0:25:25
0:25:25
 0:19:14
0:19:14
 0:11:30
0:11:30
 0:05:07
0:05:07
 0:15:52
0:15:52
 0:01:42
0:01:42
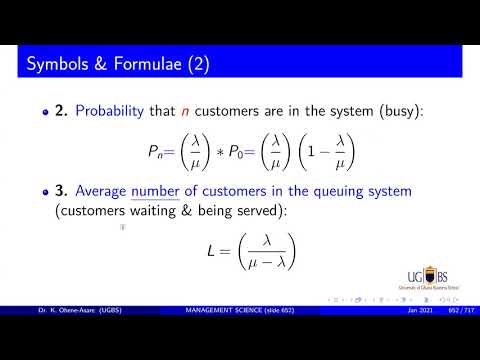 0:20:27
0:20:27
 0:28:12
0:28:12
 0:08:37
0:08:37
 0:06:34
0:06:34
 0:08:56
0:08:56
 0:11:53
0:11:53
 0:17:48
0:17:48
 0:13:24
0:13:24
 0:08:32
0:08:32
 0:16:33
0:16:33
 0:17:59
0:17:59
 0:00:25
0:00:25
 0:10:44
0:10:44
 0:15:19
0:15:19
 0:14:55
0:14:55
 0:33:40
0:33:40
 0:20:50
0:20:50
 0:11:32
0:11:32