Derivative of Lambert W function

In this video, I showed the relevance and behavior of the Lambert W function and how to compute its derivative

[ambikachhikara]

Hi Mr. Ok! I had you as my Algebra 1 teacher back in middle school and remembered you had a YouTube channel, and now I am in AP Calculus BC and your videos come in handy. It’s great to see that your channel has grown so much!

[rhc-weinkontore.k.]

This is fun. Prime Newtons, you are a really great teacher.

[octs]

I do not know anything of calculus, and man I hated math, but for some odd reason, I can not help, but be so intrigued. I blame my educators for me being so bad at math, but also so uninspired and uninterested, after all I was a child, but I commend you for revitalizing my love for math. Your a godsend mate.

[Misteribel]

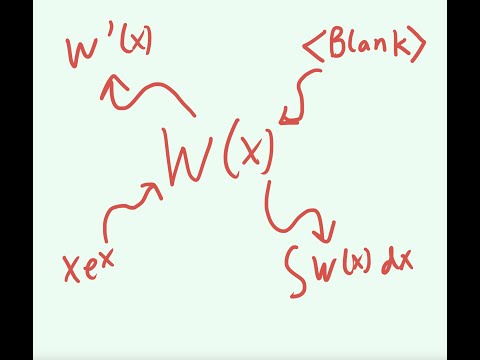
The trick you apply by taking the derivative on both sides (9:10), then using the product rule, and get back a component that's itself containing the derivative (W'(x)) really caught me off guard. So simple and so useful! It allows you to find the derivative of the productlog function by inference, using basic high school differentiation rules and never really differentiating the function itself directly.

[deathracoffee]

I just wanted to say, I really like your voice. Keep on being awesome

[weo]

Next - integration of Lambert w function

[koenth]

Your teaching skills are beyond normal!

[johnsellers]

I've taken many math courses up through graduate school and you are the best teacher I've encountered.

[rivalhunters]

aah, u forgot the bracket at the end MY OCD IS TRIGGERED. A very good video :)

[remopellegrino]

Youtube needs more Math people like you and Michael Penn

[laman]

We love how this dude is lecturing Math. Step-by-step. I have watched a number of Lambert W-function clips and they all start right away. But here, you are introduced to the fundamentals first and then how they apply to the actual problem. So, even if you have never heard of it, you can still follow the explanation. We wonder if he has this all hidden in his hat.

[johannaselbrun]

Gracias por apoyarme y me gusta tu trabajo mucho

[Ron_DeForest]

I have to say that’s an amazingly fast turnaround. Request a video one day, get it the next. Wasn’t quite what I was hoping though. Was really hoping for a deep dive into how it actually works. There’s more to it besides being very convenient. If you use the function on a calculator it comes up with an answer.

[EvilSandwich]

Thank you. So many people covered this before but they tend to just glaze over a lot of the simplification. Which usually would be fine, but for a function like this, it just feels like their skipping steps and I'm grateful you took your time and explained every step.

Any plans to explain how to integrate W(x) in a future video too?

[kusuosaiki]

I have watched few of your videos. As a Math student, I really find these interesting. Keep it up good sir.

[Ferraco]

The "third" version really just gives you back the first version.

On another note, you could write a "fourth" version:

d/dx [ln(W(x))] = 1/[x(1+W(x))]

[lambertWfunction_]

goated teacher man, great explanation

[ferretcatcher]

This is elegant mathematics. ❤ the use of the chalkboard. Reminds me of my salad days at university.

[ikhsanmnoor]

Then I meet this really good explanation

[CalculusIsFun]

Alternatively you could have used the formula for inverse functions derivative based on the regular function.

If y = f^-1(x) then f(y) = x

1 = f’(y) * dy/dx

Dy/dx = 1/f’(y)

y = f^-1(x)

Therefore the derivative of any inverse function can be represented using its none inverse counterpart as dy/dx = 1/f’(f^-1(x))

Let apply this to lambert.

The derivative of xe^x = e^x(1 + x)

so d/dx(w(x)) = 1/f’(w(x)) where f’ is e^x(1 + x)

So derivative of the lambert function is 1/(e^w(x) * (1 + w(x))