Ciprian Manolescu | Four-dimensional topology

In the Spring 2020 semester, the CMSA will be hosting a lecture series on literature in the mathematical sciences, with a focus on significant developments in mathematics that have influenced the discipline, and the lifetime accomplishments of significant scholars. Talks will take place throughout the semester. All talks will take place virtually.

Speaker: Ciprian Manolescu (Stanford)

Title: Four-dimensional topology

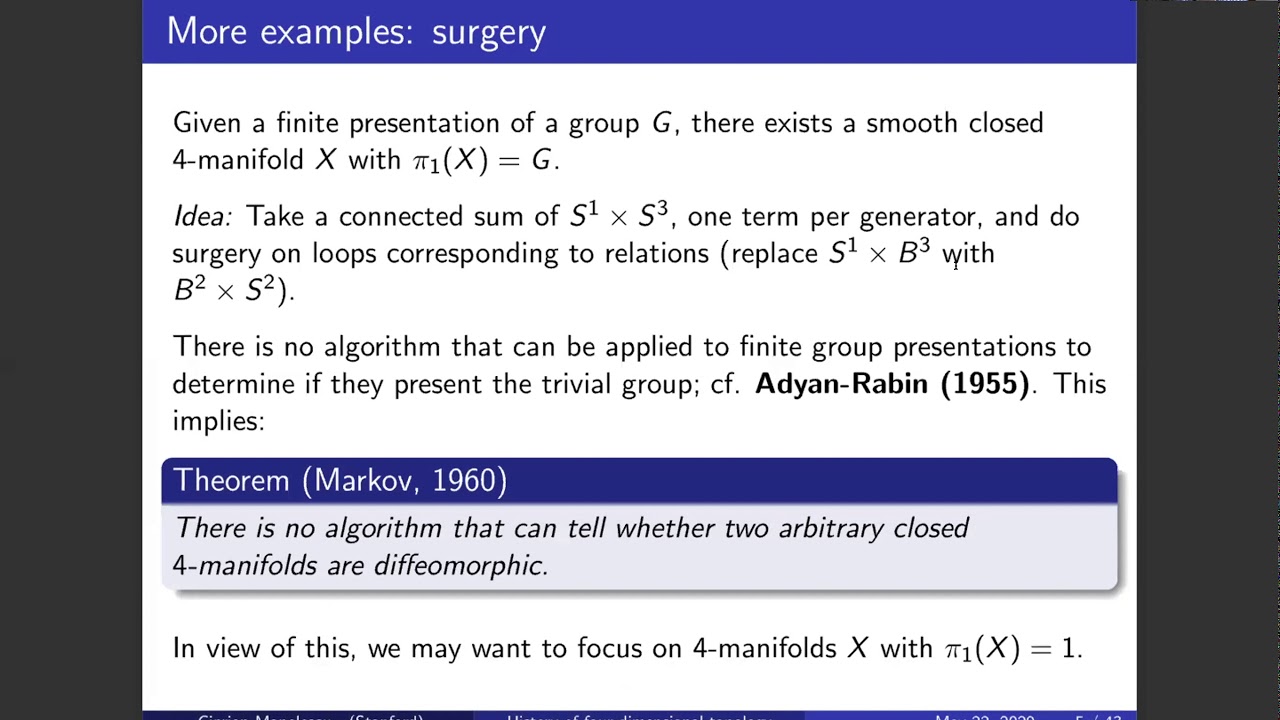
Abstract: I will outline the history of four-dimensional topology. Some major events were the work of Donaldson and Freedman from 1982, and the introduction of the Seiberg-Witten equations in 1994. I will discuss these, and then move on to what has been done in the last 20 years, when the focus shifted to four-manifolds with boundary and cobordisms. Floer homology has led to numerous applications, and recently there have also been a few novel results (and proofs of old results) using Khovanov homology. The talk will be accessible to a general mathematical audience.

[JanPBtest]

Actually the term "gauge" as used by mathematicians is a bit of a misnomer. In particle physics gauge (or, more usefully, _local_ gauge invariance) refers to certain invariances of Lagrangians. There is an aspect of this that can be written as a covariant derivative property (a "connection"). But physicists don't study those connections per se, they are interested in the Lagrangians (which, curiously, never seem to interest mathematicians). In fact, studying manifolds of connections in the physics context would completely fall apart from the get-go because in physics all this is done using the Minkowski (indefinite) metric, so the Yang-Mills equations are not elliptic, so the entire finite-dimensionality program and the Atiyah-Singer theorem are false there. I'm not sure why mathematicians latched onto the word "gauge theory", it's weird.

[johnstfleur]

"I AM NOW ONE OF YOU TO CREATE THE ARTICIAL INTELLIGENCE."

[johnstfleur]

Humbly speaking why can we use differential equations as topological group operators themselves in tensor contraction to prove the point are conjecture for the knot of a proof theory itself?

[johnstfleur]

I AM HE. THE 5 DIMENSIONAL MATHEMATICIAN.

[johnstfleur]

KIRBY MANIFOLDS OF DIMENSION 4 ACTUALLY EXIST.