filmov
tv
How to solve limit of sin(2x)/sin(5x) as x approaches 0

Показать описание
How to algebraically solve the limit as x approaches 0 of the function sin(2x)/sin(5x)?
In this question we deploy the use of basic limit laws and substitution techniques that allow our trig function to be solved algebraically.
Please consider supporting the channel on Patreon:
Music by Adrian von Ziegler
In this question we deploy the use of basic limit laws and substitution techniques that allow our trig function to be solved algebraically.
Please consider supporting the channel on Patreon:
Music by Adrian von Ziegler
3 WAYS TO SOLVE LIMITS
Class 11 Maths | How We Solve Limit? - Limits and Derivatives
L-Hospital Rule | Limits Tricks | MH-CET | Mathematics | How to solve in Minimum Time
How to solve LIMITS BY FACTORING (KristaKingMath)
How to Solve 1^Infinity Indeterminate Form Limits | Calculus 1 Exercises
LIMITS SHORTCUT TRICK: Solve limit at infinity (very fast)
How to solve limit for grade 11-12 | KhmerScience
Super Shortcut Tricks Limits and Derivatives | Solve Limits Questions In 3 Seconds | Harsh Sir
Calculate Limit #anime
How to Solve Limits Using Tabular Methods and Graphical Methods | Dave Jeffrey Camposano
Solve Limits question in 10 sec | Super Trick | IIT JEE Maths | ATP STAR | Harsh Sir
Limits | Solve Any Que. in 5 Seconds | Limits and continuity CBSE NCERT Maths in Hindi | Lecture 1
Neat ways to solve complicated limits.
Limit for jee mains #jeemains #limit #iitjee
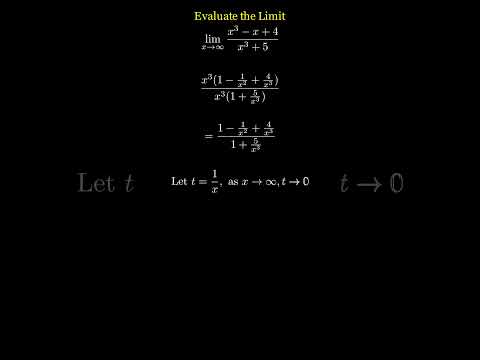
How to Solve The Limit of x^4 - 1 / x^3 - 1, x approaches 1.
Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy
How to Solve Polynomial Limits at Infinity (simple)
Solve Limit of a Function using Numerical Method
Fastest way to solve limit n tends to Infinity sums
how to solve limit equation
LIMITS CALCULATOR TECHNIQUE: Solve fast(in seconds) with casio fx-991 es plus calculator.
Solve LIMITS in just 10 seconds! | Limits Short Trick for JEE Main 2025 | JEEfinity
how to solve a rubix cube
📚 How to solve an upper-limit, exponential decay problem
Комментарии
 0:05:00
0:05:00
 0:09:13
0:09:13
 0:08:48
0:08:48
 0:04:32
0:04:32
 0:10:38
0:10:38
 0:05:31
0:05:31
 0:06:26
0:06:26
 0:27:08
0:27:08
 0:00:29
0:00:29
 0:53:03
0:53:03
 0:07:17
0:07:17
 0:22:44
0:22:44
 0:10:47
0:10:47
 0:00:37
0:00:37
 0:01:08
0:01:08
 0:09:16
0:09:16
 0:02:32
0:02:32
 0:15:21
0:15:21
 0:08:15
0:08:15
 0:14:48
0:14:48
 0:11:31
0:11:31
 0:06:15
0:06:15
 0:00:17
0:00:17
 0:03:33
0:03:33