filmov
tv
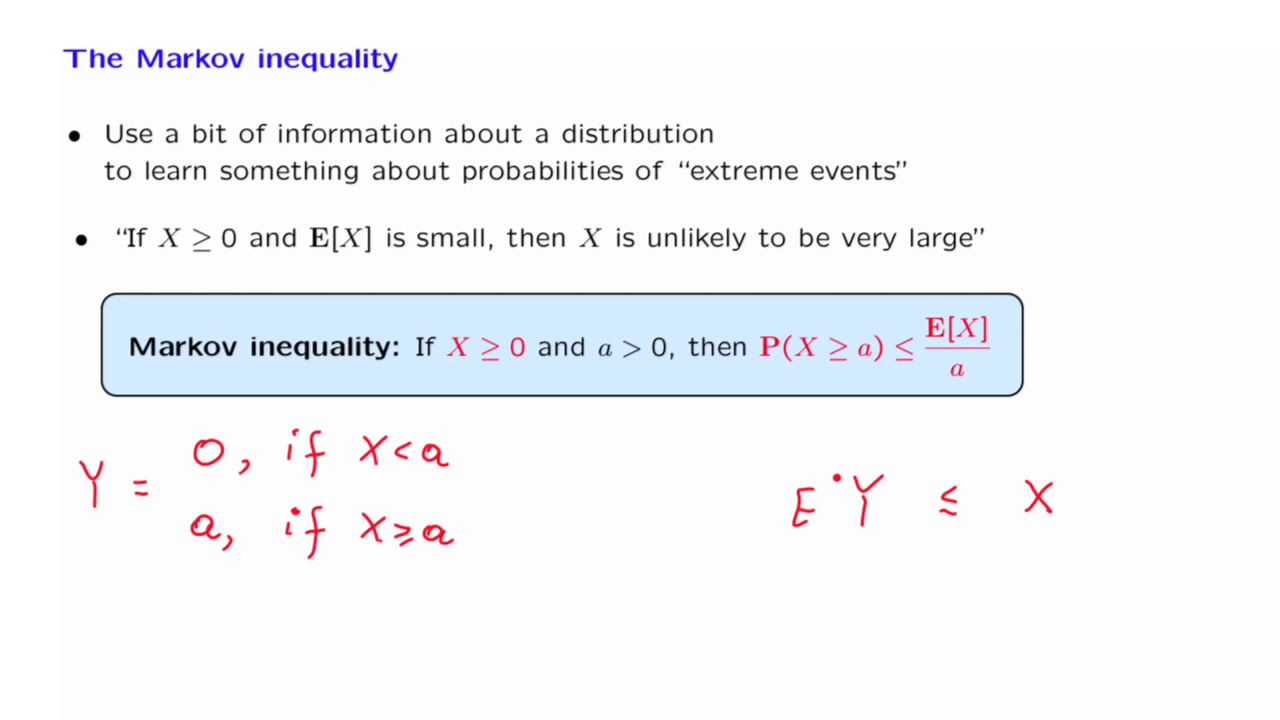
L18.2 The Markov Inequality
Показать описание
MIT RES.6-012 Introduction to Probability, Spring 2018
Instructor: John Tsitsiklis
License: Creative Commons BY-NC-SA
Instructor: John Tsitsiklis
License: Creative Commons BY-NC-SA
L18.2 The Markov Inequality
Markov's Inequality ... Made Easy!
Markov's Inequality
Markov's Inequality
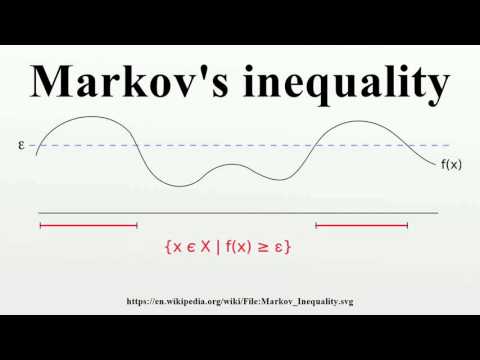
Markov's inequality
Markov and Chebyshev Inequalities
PB39: Markov and Chebyshev Inequalities
Probability Theory 108 Markov's Inequality
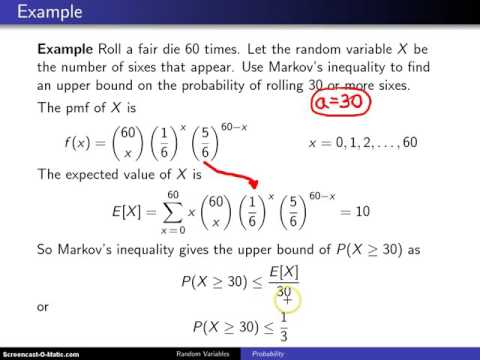
Markov's inequality -- Example 1
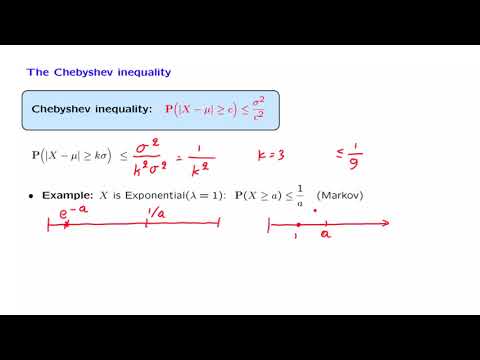
L18.3 The Chebyshev Inequality
Markov's Inequality Intuition
Markov Inequality and its Examples
09-07. Convergence and limit theorems - Markov's inequality and Chebyshev's inequality.
STATISTICS- Chebyshev's InEquality
Markov's Inequality - Intuitively and visually explained
06.02 Markov Inequality: Examples, and Chebyshev Inequality
10-2 Markov's Inequality (First Course in Probability)
Markov inequality example 1
Pillai 'Markov's Inequality and Gaussian Bounds'
Markov's inequality
Markov's inequality
Proof of Markov's Inequality
Chebyshev's Inequality
Episode Negative 1: Markov's Inequality (Proof)
Комментарии
 0:10:21
0:10:21
 0:09:25
0:09:25
 0:05:49
0:05:49
 0:08:02
0:08:02
 0:02:16
0:02:16
 0:05:54
0:05:54
 0:07:45
0:07:45
 0:15:00
0:15:00
 0:02:46
0:02:46
 0:05:57
0:05:57
 0:05:34
0:05:34
 0:15:47
0:15:47
 0:09:53
0:09:53
 0:04:40
0:04:40
 0:19:30
0:19:30
 0:06:56
0:06:56
 0:13:45
0:13:45
 0:02:13
0:02:13
 0:15:24
0:15:24
 0:03:34
0:03:34
 0:15:23
0:15:23
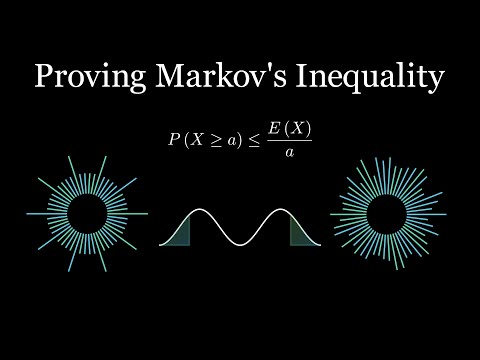 0:11:43
0:11:43
 0:03:50
0:03:50
 0:13:04
0:13:04