filmov
tv
Double Integration Method Example 2 (1/2) - Mechanics of Materials

Показать описание
This is a double integration method example problem for a simply supported beam with linear and uniform distributed loads. This video shows how to calculate slope and displacement functions and then determine the maximum displacement of the beam.
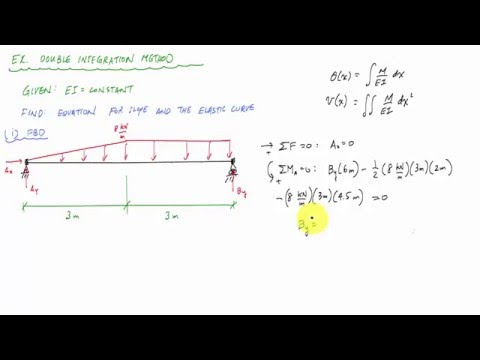
Part 1: Calculating Reactions, Moment Functions, and Antiderivatives
Part 2: Identifying boundary and continuity conditions, solving for constants, determining the maximum deflection of the beam
Part 1: Calculating Reactions, Moment Functions, and Antiderivatives
Part 2: Identifying boundary and continuity conditions, solving for constants, determining the maximum deflection of the beam
Double Integration Method Example 2: Part 1
Double Integration Method Example 2 (1/2) - Mechanics of Materials
Double Integration Method Example 2: Part 2
Double Integration Method Example 2 (2/2) - Mechanics of Materials
Double Integration Method Example 1: Part 2
How to find Slope and Deflection using Double Integration Method | Example 02 | Easy way | 2022
DAC12503 Double Integration Method Example 2
Double Integration Method Example II
Complex: Cauchy's Thm, Integral Thm, Generalized Integral Formula, Morera, Goursat, 10-15-24 pa...
Strength of Materials: Double Integration Method (Beam Deflection) Part 2 of 2
Mechanics of Materials: Lesson 64 - Slope and Deflection Equation Example Problem
Deflection in Beams: Double Integration Method | Example 2 | Strength of Materials (Filipino)
8.6 Double Integration Method (Example 2)
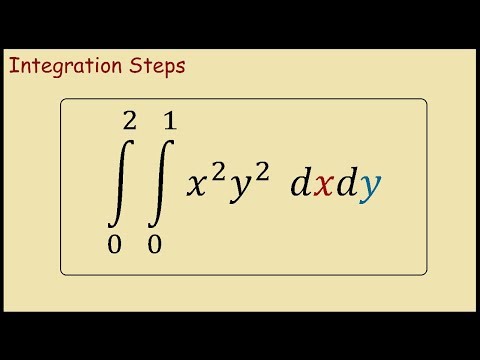
How to solve double integrals (steps)
Lecture 011 - Slope and Deflecion Example Using Double Integration Method
Deflection of Beams Using Double Integration Method, Structural Analysis for Deflection Example 2
Strength of Materials: Double Integration Method (Beam Deflection) Part 1 of 2
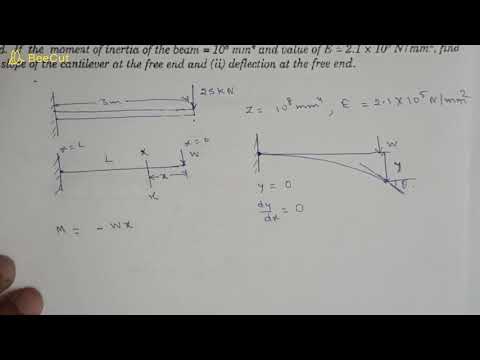
Deflection of beams 02 //double integration method//Cantilever beam with point load
Double Integration Method Example 1: Part 1
Deflection of Beams Using Double Integration Method, Structural Analysis for Deflection Example 5
Double Integration Method
Deflection of Beams || Double Integration Method_Cantilever Beams || Lecture 2
Deflection of Beams Using Double Integration Method, Structural Analysis for Deflection Example 7
8.12 Double Integration Method for Non Prismatic Beams (Example 2)
Комментарии
 0:08:07
0:08:07
 0:08:11
0:08:11
 0:07:12
0:07:12
 0:10:04
0:10:04
 0:09:50
0:09:50
 0:10:25
0:10:25
 0:40:27
0:40:27
 0:19:47
0:19:47
 0:59:51
0:59:51
 0:18:30
0:18:30
 0:27:52
0:27:52
 0:33:50
0:33:50
 0:20:43
0:20:43
 0:02:22
0:02:22
 0:10:49
0:10:49
 0:00:33
0:00:33
 0:39:45
0:39:45
 0:16:11
0:16:11
 0:08:29
0:08:29
 0:00:31
0:00:31
 0:07:22
0:07:22
 0:49:12
0:49:12
 0:00:33
0:00:33
 0:19:14
0:19:14