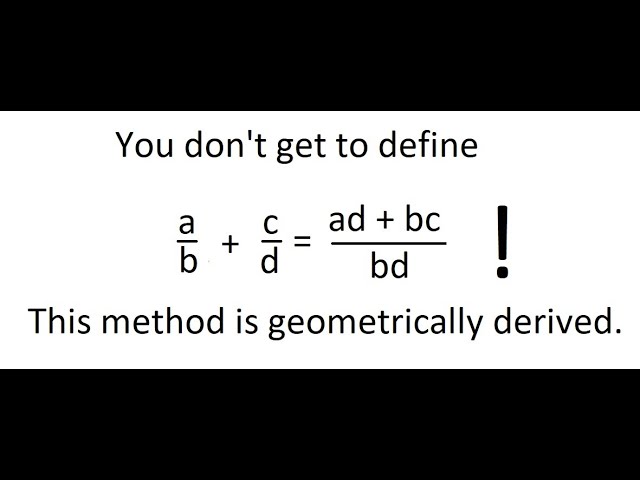
The arithmetic operators are not 'defined', they are derived from sound geometry.

No, you don't get to define p/q + r/s to be any old nonsense you like. p/q + r/s is exactly equal to (ps + qr)/(qs) and is geometrically derived. Thanks to the Ancient Greeks whose minds weren't polluted by set theoretic garbage! Thanks to their clarity of thought, we can perform the basic operations of arithmetic and in the case of mainstream academia, without any understanding thereof whatsoever!

If you asked a fool like Meel to explain to you how the arithmetic operations are the same for all numbers, he will not be able to! His answer will be something to the effect of "It is defined that way." Why is 1/2 x 3/4 = 3/8 which is less than both the operands and why is 3 x 5 = 15 where 15 is greater than both the operands? Meel will not be able to explain to you how these operations can be done exactly the same way, regardless of the type of fraction - whether natural number or otherwise. His syphilis brain has been infected with the rot of Georg Cantor's set theory.

To say as the ignoramus Meel says:

Let ∼ denote the equivalence relation on F given by a/b ∼ c/d if and only if ad = bc. For the remainder of this section, when we use the word fraction, we shall mean an element of F. We define the operations of addition and multiplication on F by a/b + c/d = (ad + bc) / bd, and a/b · c/d = ac/bd.

shows a complete lack of understanding. Firstly, the condition ad = bc is the reason a/b is EQUAL or PROPORTIONAL to c/d and the reason he can claim an "equivalence relation". Multiplication has already been assumed here. The dimwit then goes on to state how fractions are multiplied when he has already assumed the fact that ad=bc implies and is implied by a/b = c/d. There is so much circularity and lack of understanding that trying to correct fools like Meel is a lost cause. They are incorrigible idiots. One might call mainstream math academics math Trumpers. The injection of non-mathematics such as "set theory" which includes fields, sets, elements, etc, facilitates the endless art of hand waving that is so prevalent among mainstream mathematics baboons.

Thank me for enlightening you here: