filmov
tv
Laplace Transform Full Guide

Показать описание
Desmos graphs:
Laplace Transform Full Guide
What does the Laplace Transform really tell us? A visual explanation (plus applications)
Laplace Transform Ultimate Study Guide
How to solve differential equations
Laplace Transform Practice
Laplace Transform in One Video |Laplace Transform Ultimate Reference Guide|Higher Engineering Maths
Ultimate Inverse Laplace Transform Study Guide
Laplace Transform Introduction - Advanced Engineering Mathematics
BSC maths 1st semester✍️ bsc maths Semester 1 #mjpru #bsc #bscmaths #bscnotes @BSCKiPathshala
The Laplace Transform and the Important Role it Plays
Using Laplace Transforms to solve Differential Equations ***full example***
The Laplace transform
Inverse Laplace Transforms - Full Tutorial
Inverse Laplace Transforms Ultimate Study Guide|Complete Reference Guide for Inverse Laplace
Laplace Transform Formula Guide | Perfect Revision for Engineering Students
Laplace transform l Formulae l Inverse Laplace transform
Differential equations, a tourist's guide | DE1
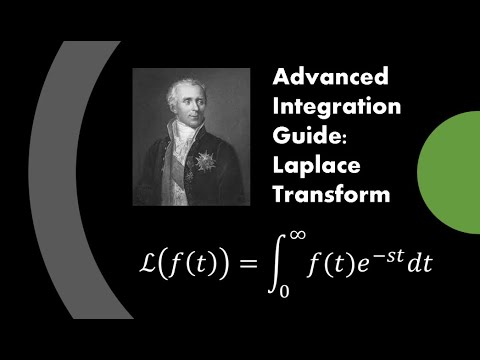
Everything YOU Need to Know about the Laplace Transform: Advanced Integration Guide
solve differential with laplace transform, sect 7.5#3
Laplace Transforms - Introduction (Differential Equations)
Mastering Laplace Transform Formulas: A Comprehensive Guide
Laplace Transform - First Translation Theorem | Math with Janine
Laplace Transforms and Differential Equations
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Комментарии
 0:24:06
0:24:06
 0:20:25
0:20:25
 3:10:51
3:10:51
 0:00:46
0:00:46
 0:10:54
0:10:54
 5:44:18
5:44:18
 2:56:15
2:56:15
 0:25:44
0:25:44
 0:10:07
0:10:07
 0:10:13
0:10:13
 0:09:30
0:09:30
 0:01:01
0:01:01
 1:15:18
1:15:18
 4:53:17
4:53:17
 0:08:17
0:08:17
 0:00:13
0:00:13
 0:27:16
0:27:16
 0:25:21
0:25:21
 0:07:52
0:07:52
 0:26:42
0:26:42
 0:07:05
0:07:05
 0:05:33
0:05:33
 0:18:11
0:18:11
 0:00:38
0:00:38