filmov
tv
Determinant of a matrix of polynomials
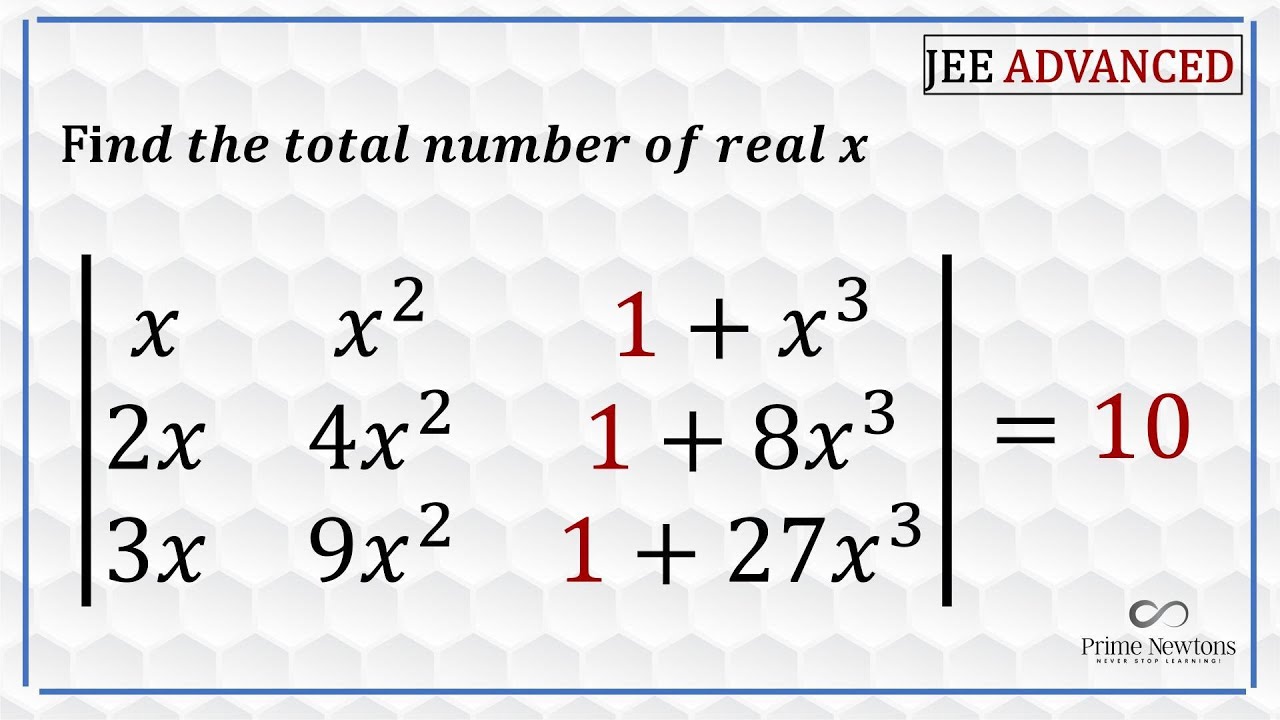
Показать описание
This question requires that the determinant of this matrix with polynomial entries is equal to 10 for real values of x. The solution employs the use of certain properties of determinants that may not be often talked about.
Determinant of 3x3 Matrices, 2x2 Matrix, Precalculus Video Tutorial
The determinant | Chapter 6, Essence of linear algebra
What is a determinant?
Evaluating the Determinant of a Matrix
Determinant of a Matrix Class 9
How To Find The Determinant of a 4x4 Matrix
How To Find The Determinant of a 3x3 Matrix
Trick for finding the the determinant of a Matrix
Determinant of matrix. #maths #mathematics #mathstutor #determinant #matrix #maths ix
Determinant of a 3 by 3 Matrix
Matrices | Determinant of a Matrix (Part 1) | Don't Memorise
n x n determinant | Matrix transformations | Linear Algebra | Khan Academy
Quick determinant trick
Finding the determinant of a 3x3 matrix method 1 | Matrices | Precalculus | Khan Academy
Determinant of a Matrix - How to Find (2x2 & 3x3)
Matrix Determinants (2 of 3: The Determinant's Geometric Meaning)
Finding the determinant of a 3x3 matrix method 2 | Matrices | Precalculus | Khan Academy
Determinant of 3x3 and 4x4 Matrices Shortcut | How to find determinant of matrix
How to Find a 3x3 Determinant
Simpler 4x4 determinant | Matrix transformations | Linear Algebra | Khan Academy
Finding the Determinant of a 3 x 3 matrix
✅▶ DETERMINANT of a MATRIX by applying PROPERTIES
How to Find The Determinant of a 4x4 Matrix (Shortcut Method)
How To Find The Determinant Of 2x2 Matrix.
Комментарии
 0:10:17
0:10:17
 0:10:03
0:10:03
 0:02:51
0:02:51
 0:07:09
0:07:09
 0:00:18
0:00:18
 0:11:29
0:11:29
 0:11:31
0:11:31
 0:05:31
0:05:31
 0:00:49
0:00:49
 0:07:10
0:07:10
 0:01:33
0:01:33
 0:18:40
0:18:40
 0:01:13
0:01:13
 0:02:39
0:02:39
 0:05:46
0:05:46
 0:10:35
0:10:35
 0:03:57
0:03:57
 0:13:32
0:13:32
 0:01:01
0:01:01
 0:09:13
0:09:13
 0:06:56
0:06:56
 0:04:56
0:04:56
 0:05:46
0:05:46
 0:07:27
0:07:27