filmov
tv
Chapter 20. Lagrangian and Hamiltonian mechanics (Part 1)
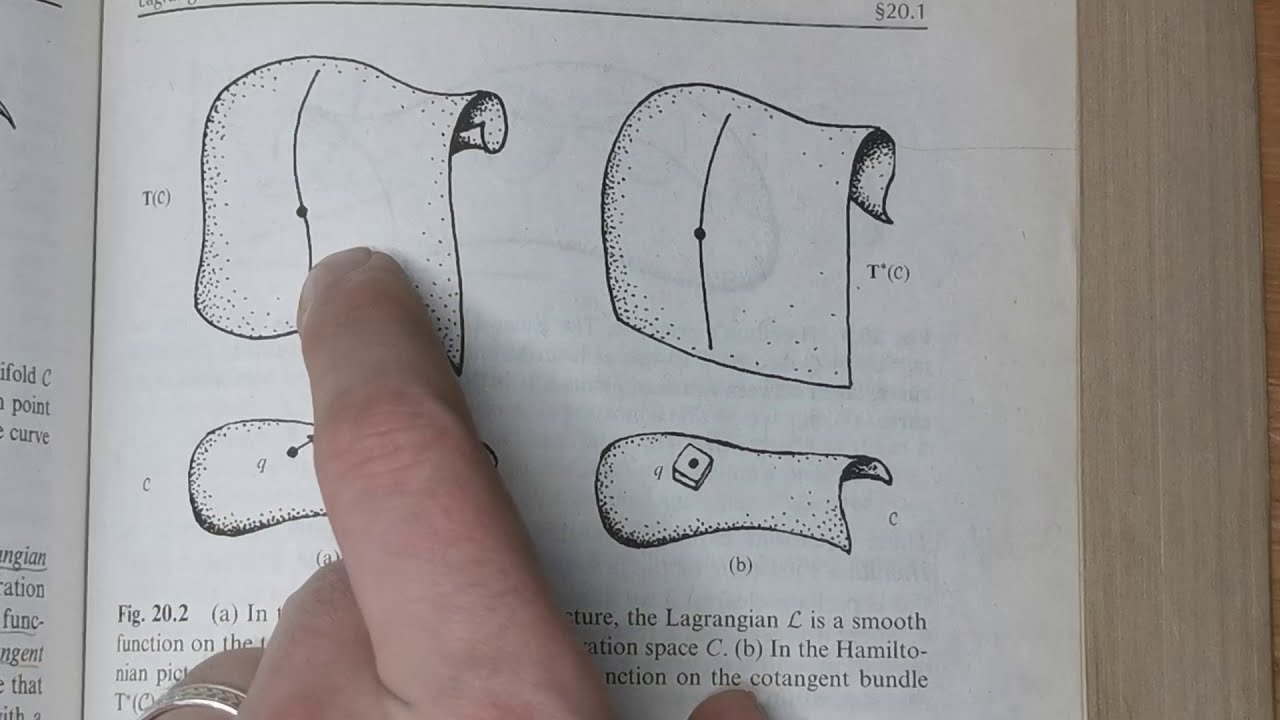
Показать описание
Here we talk about 20.1 - 20.3.
Additional literature:
1. Landau, Lifshitz - Mechanics. Is a pretty straightforward introduction to the matter. I suggest reading the first two chapters. (sometimes you will feel that you need some explanations, authors were probably thinking that you should work on a full-fledged explanation by yourself). Anyway - it contains a great intro about Lagrangians, their independence of scaling and full-time derivatives, and connection of the v^2 dependence to the homogeneity of space and time and isotropy of space.
2. Gelfand, Fomin - Calculus of Variations. Detailed mathematical approach. With physical examples as exercises (solved for you).
3. Susskind, Hrabovsky - Theoretical Minimum. Probably the easiest and the lightest introduction into a subject.
Additional literature:
1. Landau, Lifshitz - Mechanics. Is a pretty straightforward introduction to the matter. I suggest reading the first two chapters. (sometimes you will feel that you need some explanations, authors were probably thinking that you should work on a full-fledged explanation by yourself). Anyway - it contains a great intro about Lagrangians, their independence of scaling and full-time derivatives, and connection of the v^2 dependence to the homogeneity of space and time and isotropy of space.
2. Gelfand, Fomin - Calculus of Variations. Detailed mathematical approach. With physical examples as exercises (solved for you).
3. Susskind, Hrabovsky - Theoretical Minimum. Probably the easiest and the lightest introduction into a subject.
 0:40:47
0:40:47
 0:18:33
0:18:33
 1:14:37
1:14:37
 1:06:22
1:06:22
 0:11:35
0:11:35
 0:12:26
0:12:26
 0:04:40
0:04:40
 0:17:19
0:17:19
 0:23:50
0:23:50
 0:14:24
0:14:24
 0:00:16
0:00:16
 0:06:26
0:06:26
 0:25:35
0:25:35
 0:00:49
0:00:49
 0:04:44
0:04:44
 0:21:57
0:21:57
 0:09:01
0:09:01
 0:00:10
0:00:10
 0:14:17
0:14:17
 0:11:55
0:11:55
 0:09:43
0:09:43
 1:02:12
1:02:12
 0:06:44
0:06:44
 0:09:56
0:09:56