filmov
tv
Boundary Conditions of the Wave Equation - Partial Differential Equations | Lecture 19

Показать описание
Now that we have derived a new PDE, we need to consider appropriate boundary conditions. In this lecture we discuss and derive boundary conditions for the 1-dimensional wave equation describing vibrations of a string. An interesting set of boundary conditions comes from considering one end of the string attached to a vibrating spring-mass system. In this case we find that the boundary condition becomes analogous to Newton's law of cooling for the heat equation.
Follow @jbramburger7 on Twitter for updates.
Follow @jbramburger7 on Twitter for updates.
PHYS 201 | Wave Interface 4 - Boundary Conditions
2D Wave Equation Simulation - Dirichlet Boundary conditions
2D Wave Equation Simulation - Neumann Boundary conditions
Electromagnetic Boundary Conditions Explained
Boundary Conditions for Wavefunctions
2D Wave Equation Simulation - Absorbing Boundary conditions
What Happens When Waves Reflect | Doc Physics
Boundary Conditions — Lesson 7
Boundary conditions of one dimensional wave equation
40-Boundary conditions on the wave equation
Explain Boundary conditions of Standing Wave. | Basic Quantum Chemistry
1D Wave Equation: Open Boundary Conditions
Boundary conditions reflection
Boundary Conditions of the Wave Equation - Partial Differential Equations | Lecture 19
Lecture 25.2 - Boundary Conditions on Standing Waves
Reflection and Transmission of Transverse Waves
MM58: Dirichlet and Von Neumann BC
1D Wave Equation with Different Boundary Conditions and CFL Numbers
Boundary conditions
University Physics Lectures, Waves Under Boundary Conditions
Simulation of inhomogeneous acoustic wave equation with absorbing boundary conditions (free on top)
Haberman 4.3 - Boundary conditions for the wave equation
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (82 of 92) Barrier: Boundary Conditions
PHYS 201 | Traveling Wave 6 - Boundary Conditions
Комментарии
 0:07:05
0:07:05
 0:00:17
0:00:17
 0:00:17
0:00:17
 0:11:26
0:11:26
 0:04:43
0:04:43
 0:00:17
0:00:17
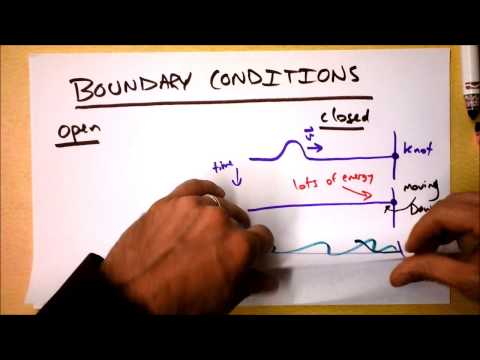 0:04:29
0:04:29
 0:05:59
0:05:59
 0:03:11
0:03:11
 0:22:46
0:22:46
 0:05:08
0:05:08
 0:00:17
0:00:17
 0:01:04
0:01:04
 0:17:14
0:17:14
 0:08:19
0:08:19
 0:31:31
0:31:31
 0:10:48
0:10:48
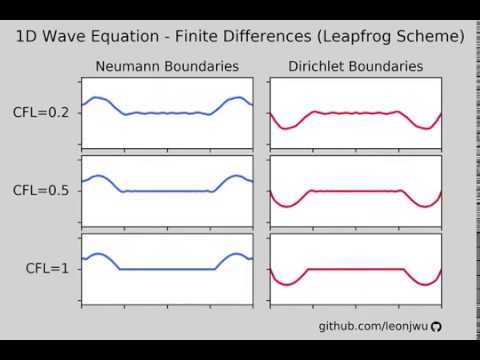 0:00:34
0:00:34
 0:03:26
0:03:26
 0:09:35
0:09:35
 0:00:31
0:00:31
 0:18:24
0:18:24
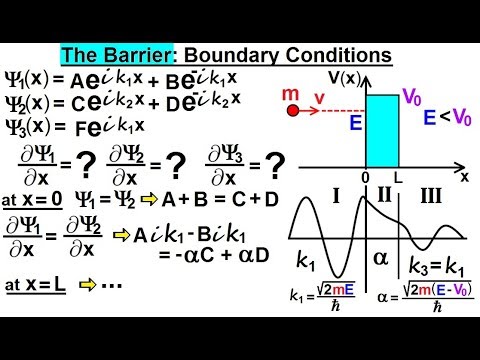 0:04:23
0:04:23
 0:04:59
0:04:59