filmov
tv
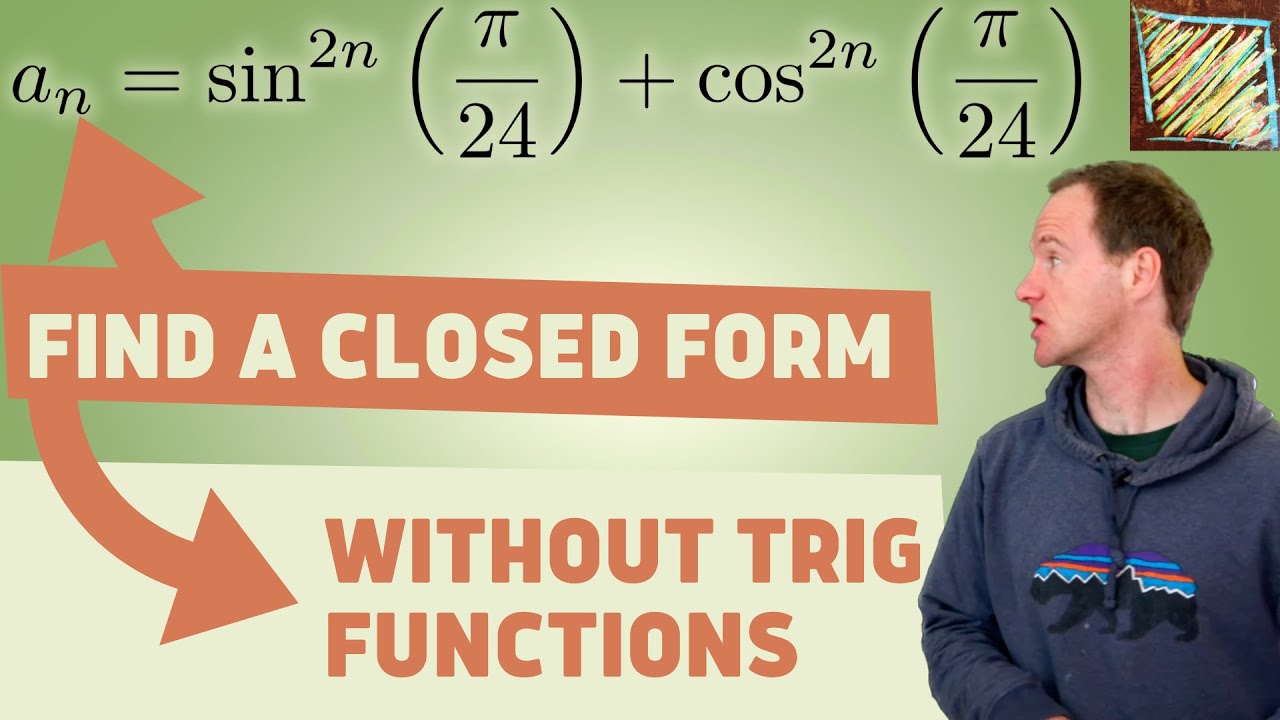
find a closed form for this INTIMIDATING SEQUENCE
Показать описание
FEEEE FIII FOOO FIDDLE! ANSWER ME THIS FAMOUS RIDDLE!
"Voiceless it cries,
Wingless flutters,
Toothless bites,
Mouthless mutters."
Chalkboard and Eraser were nearing the end of their...let's call it the Legally Distinct There and Back Again adventure when they were confronted by the Legally Distinct Gollum, a swamp troll. It screamed again.
FEEEE FIII FOOO FIDDLE! ANSWER ME THIS FAMOUS RIDDLE!
"Voiceless it cries,
Wingless flutters,
Toothless bites,
Mouthless mutters."
If you've read this far and really expect some cogent or reasonable conclusion to this story then perk up buttercup! Because once,
(watch the video get out of here...but press like...subscribe...maybe head to the Patreon that's below all this? I mean you're here already - you seem like the dedicated type.)
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
"Voiceless it cries,
Wingless flutters,
Toothless bites,
Mouthless mutters."
Chalkboard and Eraser were nearing the end of their...let's call it the Legally Distinct There and Back Again adventure when they were confronted by the Legally Distinct Gollum, a swamp troll. It screamed again.
FEEEE FIII FOOO FIDDLE! ANSWER ME THIS FAMOUS RIDDLE!
"Voiceless it cries,
Wingless flutters,
Toothless bites,
Mouthless mutters."
If you've read this far and really expect some cogent or reasonable conclusion to this story then perk up buttercup! Because once,
(watch the video get out of here...but press like...subscribe...maybe head to the Patreon that's below all this? I mean you're here already - you seem like the dedicated type.)
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
Determine a Closed Formula for a Given Sequence (1)
Finding A Closed Form Solution to Sn=S(n-1)+4n+5
48 Introduction to closed forms for sums
Finding a closed form from a recursively defined sequence
General Methods for Finding a Closed Form (Method 5 - Expand and Contract)
Example: Closed Form of a Recurrence Relation
Find the closed form expression of a sequence by changing it to a constant-recursive sequence
Finding a Closed Form Solution for the Towers of Hanoi
I, Reincarnated in the Industrial Revolution, Rose from Obscurity to Hold the World's Secrets
Find Closed-Form Expression for f(x) - part 1
General Methods for Finding a Closed Form (Method 2 - Perturb the Sum)
Lets find a closed form of this gamma series
CLOSED FORM FOR A SUM
General Methods for Finding a Closed Form (Methods 0 - Look it Up and 1 - Guess & Prove)
Finding a closed form for ζ(4)
General Methods for Finding a Closed Form (Method 3 - Repertoire)
Summations 8 Using Formulas to Find Closed Form Expressions 1
Closed form from a recursive definition
Determine a Closed Formula for a Given Sequence (2)
Sequences: closed-form formula vs recursively defined
General Methods for Finding a Closed Form (Method 4 - Integration)
Sec 3.2 How to find a closed form expression
Find the Closed Formula for a Sequence Given a Generating Function
How to Write in Closed Form for Summation in Sigma Notation IB HL SL AP Calculus
Комментарии
 0:08:05
0:08:05
 0:06:25
0:06:25
 0:07:42
0:07:42
 0:03:32
0:03:32
 0:10:40
0:10:40
 0:03:02
0:03:02
 0:09:07
0:09:07
 0:05:39
0:05:39
 9:41:05
9:41:05
 0:00:43
0:00:43
 0:12:09
0:12:09
 0:13:50
0:13:50
 0:03:03
0:03:03
 0:13:43
0:13:43
 0:13:03
0:13:03
 0:05:53
0:05:53
 0:04:09
0:04:09
 0:05:16
0:05:16
 0:07:19
0:07:19
 0:13:13
0:13:13
 0:14:25
0:14:25
 0:06:04
0:06:04
 0:04:00
0:04:00
 0:04:21
0:04:21