filmov
tv
If n is a composite positive integer then n divides (n - 1)! except when n = 4 (1001-180)

Показать описание
Let n be a positive integer. Show that if n is a composite integer then n divides (n - 1)! except when n = 4
#numbertheory #composite #integer #cipher
#numbertheory #composite #integer #cipher
Discrete Math - Proof - Composite integer n has divisor less than sqrt(n) (Request)
If n is a composite positive integer then n divides (n - 1)! except when n = 4 (1001-180)
(NUMBER THEORY) Lecture 14: if n is composite, then the smallest prime divisor of n less than sq (n)
(n-1)! is multiple of n when n≠4 is composite, in contrast to Wilson's Theorem for prime number...
A composite number n has always a prime factor not exceeding root n
Prove that n^4+4 is composite if n is greater than 1.
Show that 111- - -11(n digits, all ones) is composite if n is composite, is the converse true?
Prove n^4+4 Is Composite
NUMBER THEORY: Proof of composite integer n has prime factor not exceeding √n
Suppose n is a composite natural number, Prove 2^n - 1 is also composite
If m is prime, n is a composite number and `m+ n=240` also their LCM is 4199. Find m and n.
Theorem: Every composite integer x contains a prime factor less than equal to its square root
n⁴+4^n // prime // composite // Number Theory8 Chemaths Risers
Sigma Function | Examples | If n is composite number then show sigma (n) is greater than n + n^1/2.
Given any integer n we can find n consecutive composite integers .
Is a Number Prime or Composite? (Math for Teachers)
Is 1 a Composite Number? (Why isn't 1 Composite?)
Proof that one less than a square number is composite (not prime)
Does every arithmetic sequence have an arbitrary long number of consecutive composite members?
Solving using Wilson's theorem and definition of composite numbers (AITTTON-2.1-35)
Establishing n^4 + 4^n is composite without using Sophie Germain algebraic Identity
Euler’s Phi Function | Examples | If n is a composite number then phi (n) = n-n^1/2 | Lecture-8
Recognizing prime and composite numbers | Factors and multiples | Pre-Algebra | Khan Academy
When Can A Composite Function Exist? | A-Level H2 Math, Singapore
Комментарии
 0:02:47
0:02:47
 0:01:53
0:01:53
 0:02:55
0:02:55
 0:03:23
0:03:23
 0:02:20
0:02:20
 0:36:31
0:36:31
 0:01:32
0:01:32
 0:01:00
0:01:00
 0:05:53
0:05:53
 0:05:04
0:05:04
 0:02:31
0:02:31
 0:04:59
0:04:59
 0:04:37
0:04:37
 0:07:22
0:07:22
 0:09:11
0:09:11
 0:05:06
0:05:06
 0:02:36
0:02:36
 0:11:47
0:11:47
 0:03:51
0:03:51
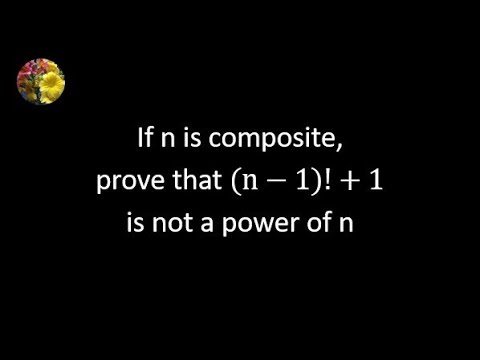 0:02:37
0:02:37
 0:09:44
0:09:44
 0:11:29
0:11:29
 0:04:40
0:04:40
 0:01:00
0:01:00