filmov
tv
Cumulative Distribution Function - Probability

Показать описание
A simple explanation of the Cumulative Distribution Function.
Cumulative Distribution Functions and Probability Density Functions
Calculating a Cumulative Distribution Function (CDF)
Probability Distribution Functions (PMF, PDF, CDF)
L08.7 Cumulative Distribution Functions
What is a Cumulative Distribution Function (CDF) of a Random Variable?
Discrete Random Variable : How to find probability from a CDF.
Cumulative Distribution Function With Example || Lesson 46 || Probability & Statistics ||
How to Find the Cumulative Distribution Function and Compute the Probabilities given the Density
Week11-12 Revision Session (Statistics I)
Continuous Random Variables: Cumulative Distribution Functions
Normal Distribution (PDF, CDF, PPF) in 3 Minutes
Random Variable, Probability Density Function, Cumulative Distribution Function
Cumulative Distribution Function (1 of 3: Definition)
Cumulative Distribution Function - Probability
How to find Cumulative Distribution Function from Probability Density Function PDF to CDF
Lecture 4.2: Cumulative distribution function
Probability : Cumulative Distribution Function F(X) : ExamSolutions
The Cumulative Probability Distribution Function
Cumulative Distribution Function CDF & Probability Density Function PDF in Random Variable
4.2 Cumulative probability distributions | Basic Statistics | Probability Distributions | UvA
Cumulative Distribution Function (2 of 3: Evaluating probabilities)
Probability Density and Cumulative Distribution Functions
Probability Mass Function and Cumulative Distribution Function - Example 1
Understanding Cumulative Distribution Function (CDF) - Part 1
Комментарии
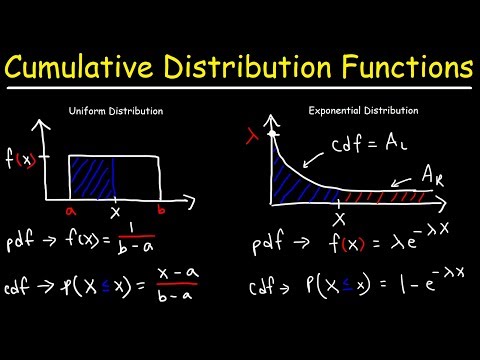 0:11:02
0:11:02
 0:08:44
0:08:44
 0:16:17
0:16:17
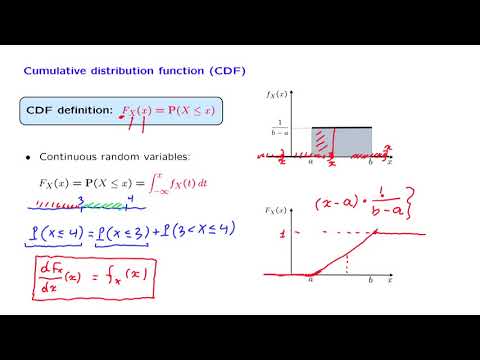 0:12:48
0:12:48
 0:11:29
0:11:29
 0:05:36
0:05:36
 0:12:34
0:12:34
 0:14:58
0:14:58
 2:30:20
2:30:20
 0:25:47
0:25:47
 0:05:26
0:05:26
 0:21:22
0:21:22
 0:12:34
0:12:34
 0:11:41
0:11:41
 0:06:44
0:06:44
 0:23:55
0:23:55
 0:05:18
0:05:18
 0:08:10
0:08:10
 0:18:32
0:18:32
 0:05:21
0:05:21
 0:09:22
0:09:22
 0:06:09
0:06:09
 0:06:22
0:06:22
 0:07:14
0:07:14