filmov
tv
70% candidates passed in English, 80% passed in mathematics, 10% failed in both. If 144 passed in
Показать описание
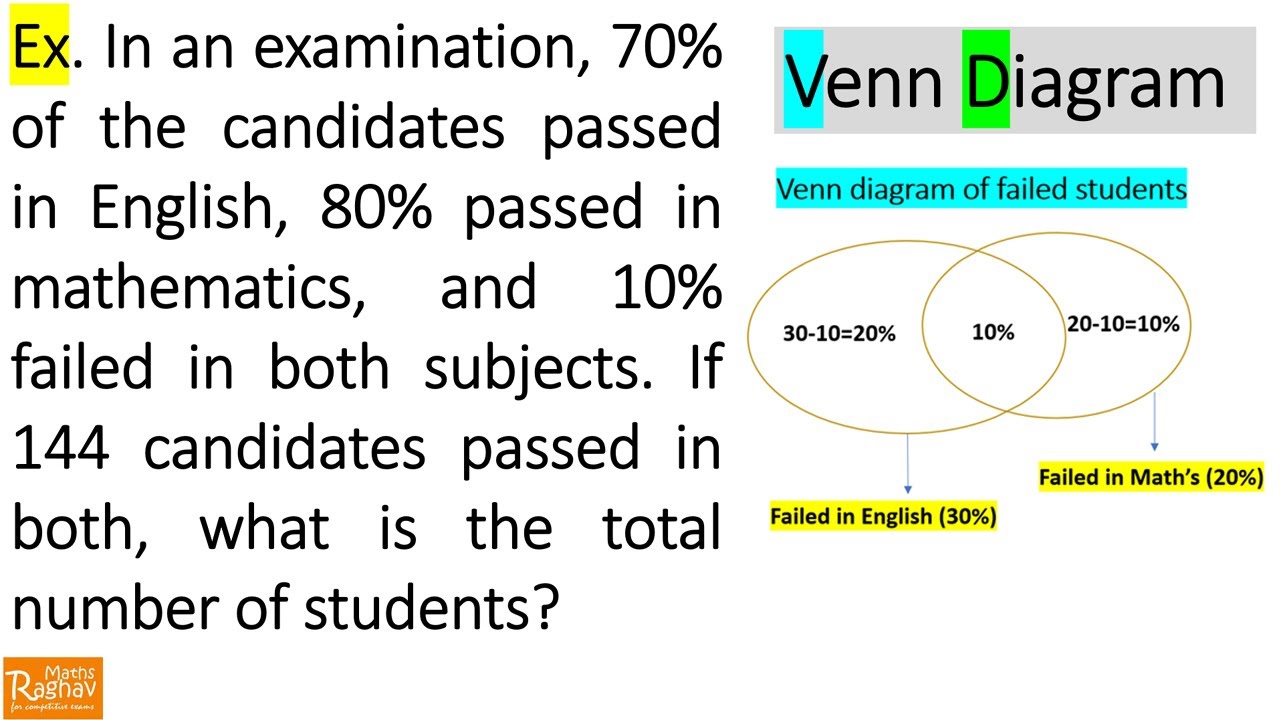
Ex. In an examination, 70% of the candidates passed in English, 80% passed in mathematics, and 10% failed in both subjects. If 144 candidates passed in both, what is the total number of students?
Ex. In an examination 60% of the candidates passed in Maths and 70% passed in English and 20% candidates failed in both the subjects. If 2500 candidates passed in both subjects, the total number of candidates appeared in examination was ?
Welcome to Raghav Maths, these are frequently asked Venn Diagram question in competitive exams, This video is related to Venn Diagram Reasoning, this complete Venn Diagram tutorial is in English. This Venn Diagram reasoning will be helpful for coming exams. I hope you will like this Venn Diagram concept and share in friends.
Keep watching Raghav Maths !
Best wishes !
Ex. In an examination 60% of the candidates passed in Maths and 70% passed in English and 20% candidates failed in both the subjects. If 2500 candidates passed in both subjects, the total number of candidates appeared in examination was ?
Welcome to Raghav Maths, these are frequently asked Venn Diagram question in competitive exams, This video is related to Venn Diagram Reasoning, this complete Venn Diagram tutorial is in English. This Venn Diagram reasoning will be helpful for coming exams. I hope you will like this Venn Diagram concept and share in friends.
Keep watching Raghav Maths !
Best wishes !
0:01:23
In an examination 70% of the candidates passed in English.80% passed in Mathematics
0:01:57
In an examination, 70% of the candidates passed in English, 80% passed in mathematics, and 10% fail
0:02:50
In an examination 70% of the candidate passed in English, 80% passed in Mathematics, 10% failed in
0:07:19
70% candidates passed in English, 80% passed in mathematics, 10% failed in both. If 144 passed in
0:01:38
In an examination, 60% of the candidates passed in English and70% of the candidates passed in
0:04:00
In an examination 70% of the candidates passed in English, 80% passed in Mathematics.
0:01:23
In an examination 80% candidates passed in English and 85%candidates passed in Mathematics.
0:02:20
In an examination, 80% of students passed in English,70% of students passed in Hindi | CSAT 2024 |
0:03:22
Out of 120 students 70% students passed in Math and 80% in English how many passed only English
0:08:46
In an examination 60% of the candidates passed in English and 70% of the candidates passed in math
0:14:10
In an examination 70% of the candidates passed in English
0:01:03
In an examination 60% of the students pass in English, 70%pass in Hindi and 40% pass in
0:01:19
In an examination 75% candidates passed in English and 60%passed in Mathematics. 25%
0:03:28
In an examination, 70% of the candidates passed in English, 80% passed in Mathematics and 109%
0:02:20
In an examination, 60% of the candidates passed in English and 70% of the candidates passed in
0:07:28
Problem 23 : In an exam 75% candidates pass in English, 65% candidates pass in Maths. 15% candidates
0:01:43
In an examination 73% of the candidates passed in quantitative aptitude test, 70% passed in
0:01:49
In an examination 80% of the boys passed in English and 85%passed in Mathematics, while
0:05:50
VENN Diagram: 80% passed in English, 85% Mathematics and 75% both. If 40 students failed
0:00:30
🔔Percentage || pass Fail percent || #youtubeshorts #mathtricks #ssc chsl #ssc cgl
0:03:45
If an exam 65% candidates passed in Biology & 70% passed in Maths while 20% failed in both|FPSC|...
0:01:38
Concept 31 | Percentage | 1EQ SSC Maths Complete Revision Book | SSC CGL CHSL CPO GD MTS 2024
0:01:44
In the final examination of class X, 70% of the candidates passed in Hindi, 55% passed in English,
0:01:13
In a class of 100 students, 55 students have passed in Mathematics and 67 students have passed in
Комментарии