Factoring Trinomials with the Box Method // Math Minute [#31] [ALGEBRA]

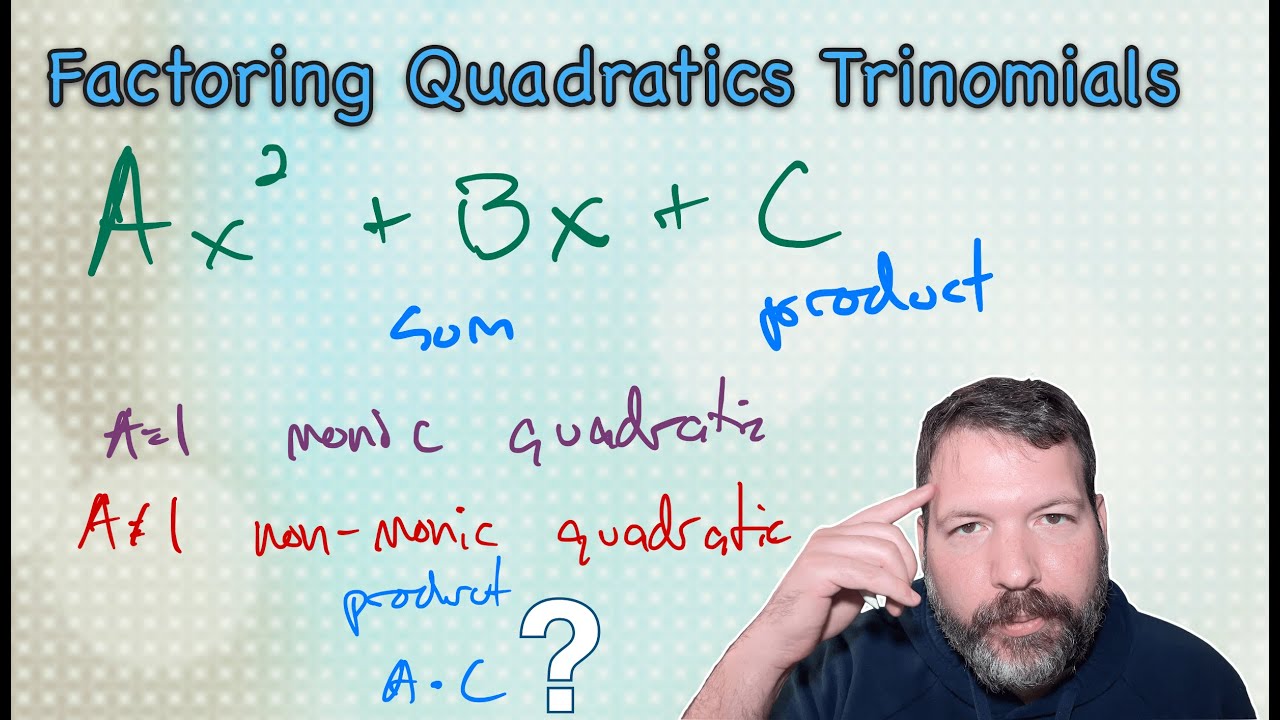
Second-degree trinomials can be split up into two groups for the purposes of factoring: those in which the coefficient of the x² term is equal to 1 (we call these monic quadratics) and those in which that coefficient is NOT equal to 1 (we call these non-monic quadratics). If a given trinomial is factorable, the monic quadratics tend to be quite straightforward. You simply want to find two numbers whose product matches the constant term of the trinomial (typically called C) and whose sum matches the coefficient of the middle term (typically, B). However, for the non-monic trinomials, it is sometimes a bit more difficult to see how the pieces fit together.

The key is to recognize that you're still looking for two numbers that have a certain product and a certain sum. In fact, the sum even still matches the same number we wanted it to before (the coefficient B). However, the product no longer needs to match not the constant term C. Instead we want to take another product A⋅C, where A is the coefficient of the squared term, and make our product match that.

Technically speaking, even for the monic trinomials, the product we want matches A⋅C, it's just that monic trinomials always have A=1, so it doesn't *look* like it makes a different to the product we look for.

From there, I personally recommend the area model for multiplication, also known as the box method, but any other factoring method will work as well.

Follow Tim Ricchuiti:

Watch more Math Videos: