filmov
tv
Laplace Applications

Показать описание
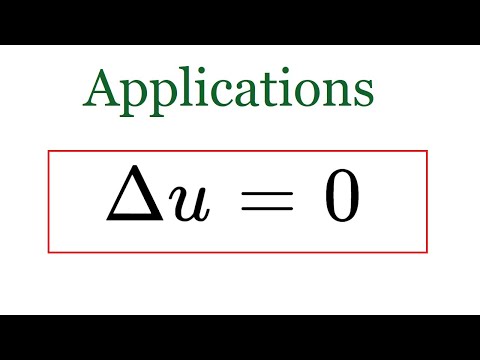
Laplace's Equation Applications
In this video, I give some very neat applications of Laplace's equation. In particular, I explain why harmonic functions are called harmonic, and I give a really cool probability application. Enjoy!
In this video, I give some very neat applications of Laplace's equation. In particular, I explain why harmonic functions are called harmonic, and I give a really cool probability application. Enjoy!
What does the Laplace Transform really tell us? A visual explanation (plus applications)
Laplace Applications
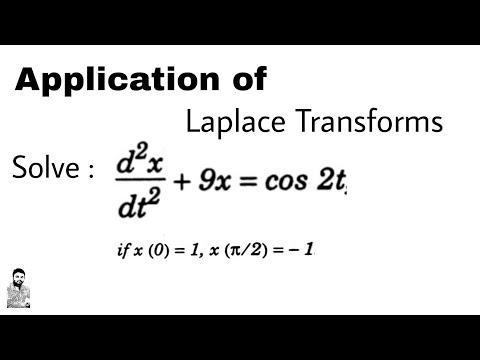
Using Laplace Transforms to solve Differential Equations ***full example***
Laplace Transform Explained and Visualized Intuitively
Why the Laplace Transform?
21. Application of Laplace Transforms | Most Important Problem#1
Intro to the Laplace Transform & Three Examples
Circuit Analysis using Laplace Transform
Laplace Transform Initial Value Problem (Example)
🔵33 - Solving Initial Value Problems using Laplace Transforms method
solve differential with laplace transform, sect 7.5#3
Applications of boundary value problems | Laplace Transforms
Application of Laplace Transform|| Zero Initial Conditions|| S domain|| Example 16.1||ENA 16.2(1)(E)
Circuit Analysis using Laplace Transform
33. Application of Laplace Transform | Complete Concept and Problem#1 | Most Important Problem
Differential Equations, Lecture 5.2: Properties & applications of the Laplace transform
Question#4 - Laplace Transform - Application to Ordinary Differential Equation.
Applications of laplace transform for circuit analysis.RLC circuit analysis by laplace transform.
Spherical and Conical capacitor || Applications of Laplace's equation for capacitance calculati...
The intuition behind Fourier and Laplace transforms I was never taught in school
22. Application of Laplace Transform | Most Important Problem#2
Applications of Laplace Transform || Example 16.1, 16.2 ,16.4 ||ENA 16.1(English)
Applications of Laplace Transform in Network Theory
mod04lec22 - Applications of Laplace transforms
Комментарии
 0:20:25
0:20:25
 0:14:00
0:14:00
 0:09:30
0:09:30
 0:19:55
0:19:55
 0:02:59
0:02:59
 0:16:29
0:16:29
 0:12:05
0:12:05
 0:08:34
0:08:34
 0:06:18
0:06:18
 0:21:44
0:21:44
 0:07:52
0:07:52
 0:02:30
0:02:30
 0:15:35
0:15:35
 0:04:24
0:04:24
 0:14:07
0:14:07
 0:57:53
0:57:53
 0:17:40
0:17:40
 0:17:59
0:17:59
 0:19:39
0:19:39
 0:18:00
0:18:00
 0:13:44
0:13:44
 0:18:49
0:18:49
 0:06:50
0:06:50
 0:33:12
0:33:12