filmov
tv
Multivariable Calculus: Distance from a point to a plane

Показать описание
In this exercise, we find the distance from the point (1, 2, 3) to the plane 3x - y + 5z = 2 using geometric methods. We start by identifying another point on the plane and connect it to our given point with a vector v. We then project v onto a line perpendicular to the plane by projecting it on the normal vector n derived from the plane's equation. The distance is calculated as the absolute value of the dot product of v and n divided by the magnitude of N. This approach emphasizes understanding vector projections for solving various distance problems in geometry without relying on specific formulas.
#mathematics #math #vectorcalculus #multivariablecalculus #linesandplanes #iitjammathematics #calculus3
#mathematics #math #vectorcalculus #multivariablecalculus #linesandplanes #iitjammathematics #calculus3
How To Find The Distance Between a Point and a Plane
Distance between two skew lines Vectors 2016 Test
Equations of Planes: Vector & Component Forms | Multivariable Calculus
Distance from Point to Plane | Vector Calculus #45
Multivariable calculus 1.3.1: Distance in Euclidean space
Distance between a point and a line (vectors) (KristaKingMath)
Calculating distance from a point to a vector equation of a line
How To Find The Distance Between 2 Points In 3D Space
Probability : L 1 G | Impossible Event and Compound Event | Class 12 Maths | NCERT CH 13 | JEE Main
Multivariate Calculus: distance to pts, lines and planes, calculus of paths intro, 1-25-17
Calculus 3: Vector Calculus in 3-D (16 of 35) Find the Distance Between a Point and a Line
distance from point to plane
[Multivariable Calculus] Equations of Planes
Distance Between a Point and a Line In 2D & 3D - Geometry
[Calculus] Vectors in the Plane Displacement and Distance Traveled
Multivariable Calculus Unit 4: Lines and Planes
Vector Calculus 22: The Distance Between a Point and a Curve
How to find the Distance Between a Point and Plane/Axis (12.1.12)
What Is The 'Normal Vector?' - Calculus 3
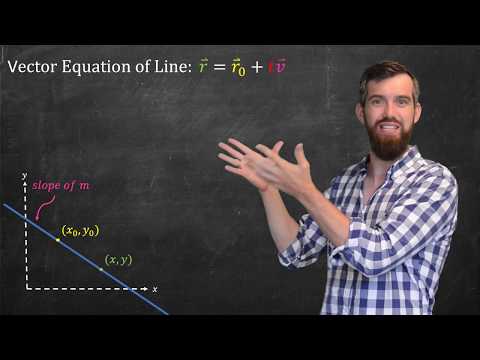
The Vector Equation of Lines | Multivariable Calculus
A Plane That Contains Three Points - Calculus 3
Projectile Motion: A Vector Calculus Problem
Multivariate Calculus: vectors, components, distance: 1-16-17
What are the big ideas of Multivariable Calculus?? Full Course Intro
Комментарии
 0:10:47
0:10:47
 0:05:52
0:05:52
 0:04:28
0:04:28
 0:13:28
0:13:28
 0:09:10
0:09:10
 0:08:52
0:08:52
 0:08:57
0:08:57
 0:10:58
0:10:58
 0:02:40
0:02:40
 0:51:57
0:51:57
 0:07:28
0:07:28
 0:07:41
0:07:41
![[Multivariable Calculus] Equations](https://i.ytimg.com/vi/Jdnh3QHsOb8/hqdefault.jpg) 0:10:49
0:10:49
 0:11:06
0:11:06
![[Calculus] Vectors in](https://i.ytimg.com/vi/ciawvSdyFEE/hqdefault.jpg) 0:08:39
0:08:39
 0:24:24
0:24:24
 0:13:12
0:13:12
 0:06:18
0:06:18
 0:00:59
0:00:59
 0:05:30
0:05:30
 0:01:00
0:01:00
 0:07:32
0:07:32
 0:51:43
0:51:43
 0:16:50
0:16:50