Circle chapter10 theorem 10.2/prove two tangents are drawn from exterior point are equal in length

hello dear
Priyanka Malik from Easy way to learn Maths

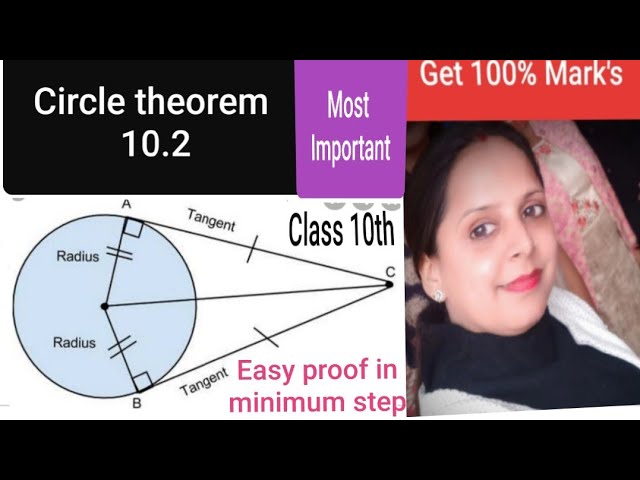
today we will prove if two tangents are drawn from an external point to a circle then the tangent are equal in length.

It is known that a tangent at any point of a circle is perpendicular to the radius through the point of contact. Thus, it is proved that the lengths of the two tangents drawn from an external point to a circle are equal. The length of tangents drawn from any external point are equal.

Let two tangent PT and QT are drawn to circle of centre O as shown in figure.
Both the given tangents PT and QT touch to the circle at P and Q respectively.

We have to proof : length of PT = length of QT
Construction :- draw a line segment ,from centre O to external point T { touching point of two tangents } .

Now ∆POT and ∆QOT
We know, tangent makes right angle with radius of circle.
Here, PO and QO are radii . So, ∠OPT = ∠OQT = 90°
Now, it is clear that both the triangles ∆POT and QOT are right angled triangle.
nd a common hypotenuse OT of these [ as shown in figure ]

Now, come to the concept ,
∆POT and ∆QOT
∠OPT = OQT = 90°
Common hypotenuse OT
And OP = OQ [ OP and OQ are radii]
So, R - H - S rule of similarity
∆POT ~ ∆QOT
Hence, OP/OQ = PT/QT = OT/OT
PT/QT = 1
PT = QT [ hence proved]