Finite element method. Heat transfer problem.

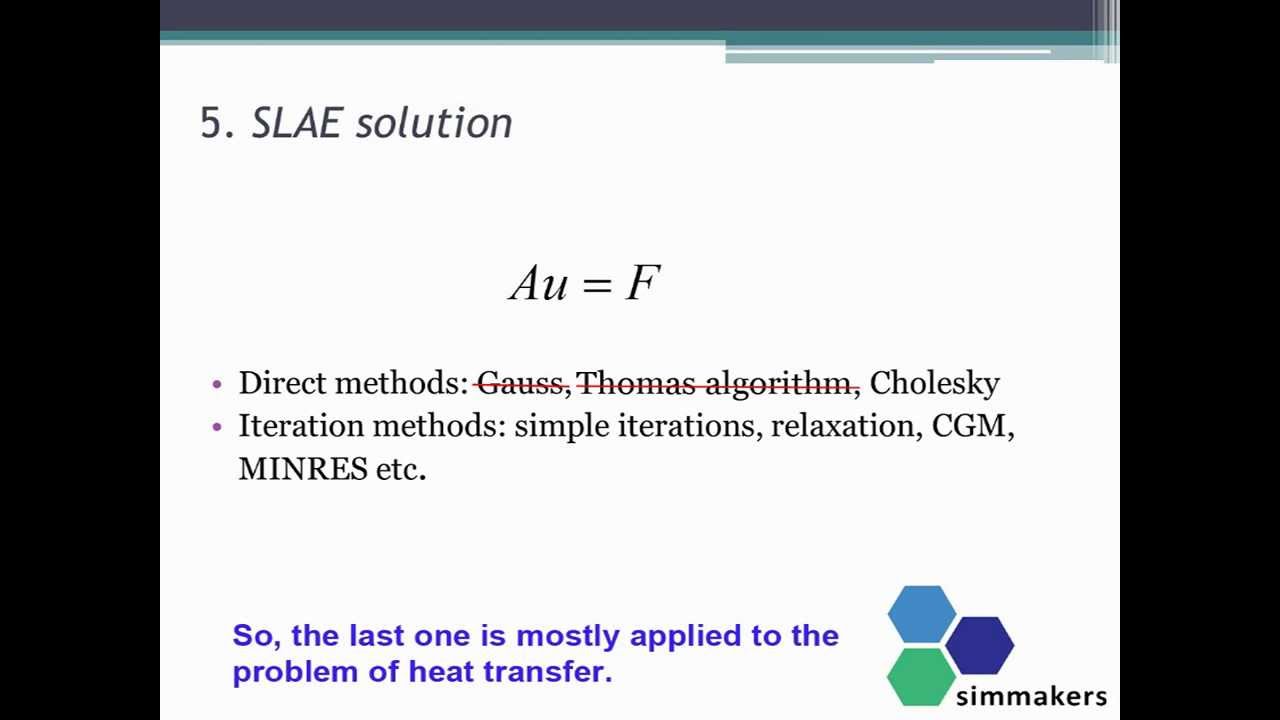
The main idea of finite element method is that we approximate a continuous function (even differentiable to a certain order), which is a solution of a problem, by means of linear combination of some basis functions. In our case Ni (x) - is the basis function. Each basis function corresponds to mesh node. The unknowns in this case are coefficients Ti, which are the value of the temperature or other physical parameter at mesh node. How to apply finite element method in order to find these unknowns? It is known that every differential problem can be reduced to algebraic. As a result we obtain as a system of algebraic equations with respect to the unknowns. How is it obtained in case of finite element method? First the area is meshed, then basis functions are selected, further we by means of some method (in case of finite element method - Galerkin method) pass on the discrete formulation of the problem and we obtain a system of equations and compute its coefficients. This system is solved by some methods, which we will describe later, and the solution is verified: is it proper or not, whether we can accept this or do we need to update the model or perhaps update the method.

[tedskandy]

any chance this could be translated into English?