Léonard Cadilhac (Sorbonne Université)

Title: Noncommutative maximal inequality and ergodic theorem for actions of amenable groups

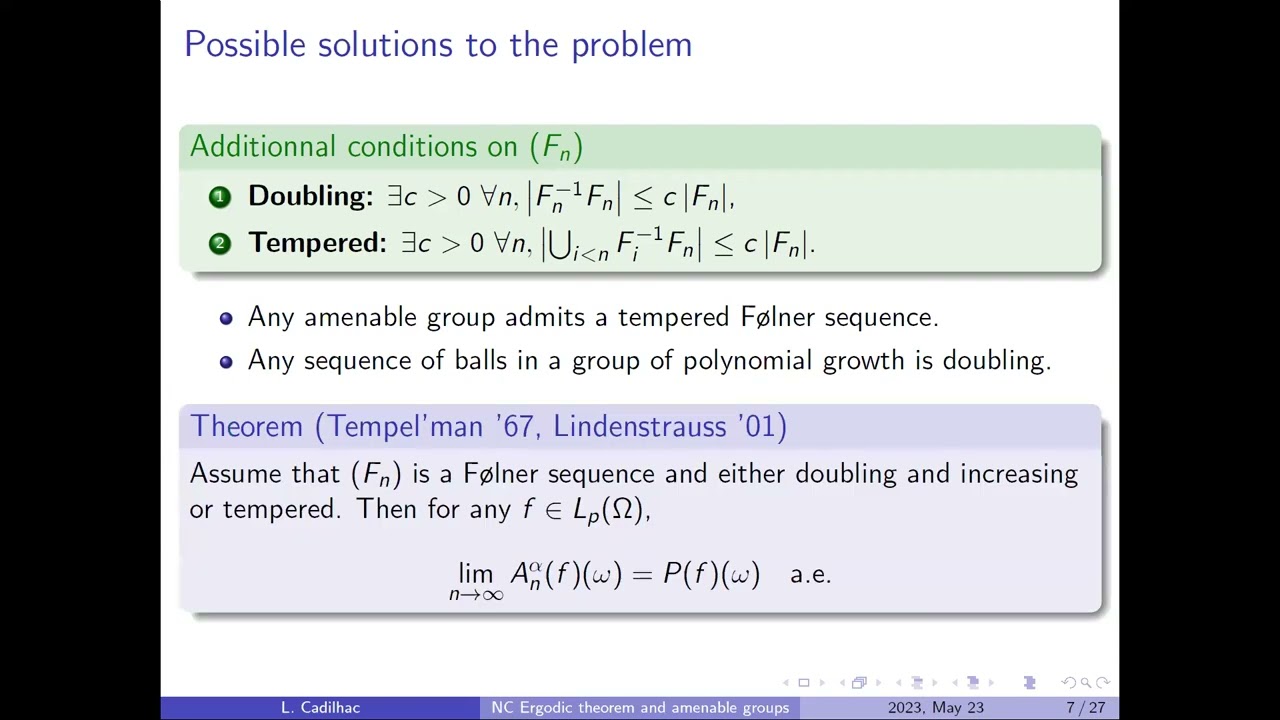
Abstract: The well-known pointwise theorem of Birkhoff establishes that ergodic averages associated with a measure preserving transformation converge almost everywhere. This original statement has been refined and generalized in many directions. In this talk, we will be interested in a pointwise theorem valid both for actions of amenable groups (rather than a single transformation) and in the noncommutative setting (actions on a noncommutative measure space). After a brief introduction of the literature leading up to this result, I will present the various techniques involved in its proof and how we adapt tools from Calderon-Zygmund theory to amenable groups. This work is a collaboration with Simeng Wang (Harbin).