Solutions to Questions on Linear Algebra in GATE 2024 for Data Science and Artificial Intelligence

Questions:

Consider the matrix 𝑴=[2−131].
Which ONE of the following statements is TRUE?

Consider the 3×3 matrix 𝑴=[123313436].
The determinant of (𝑴𝟐+12𝑴) is ______.

Select all choices that are subspaces of ℝ3.
Note: ℝ denotes the set of real numbers.
(A)
{𝐱=[𝑥1𝑥2𝑥3]∈ℝ3: 𝐱=𝛼[110]+𝛽[100],𝛼,𝛽∈ℝ}
(B)
{𝐱=[𝑥1𝑥2𝑥3]∈ℝ3: 𝐱=𝛼2[120]+𝛽2[101],𝛼,𝛽∈ℝ}
(C)
{𝐱=[𝑥1𝑥2𝑥3]∈ℝ3: 5𝑥1+2𝑥3=0,4𝑥1−2𝑥2+3𝑥3=0}
(D)
{𝐱=[𝑥1𝑥2𝑥3]∈ℝ3: 5𝑥1+2𝑥3+4=0}

Which of the following statements is/are TRUE?
Note: ℝ denotes the set of real numbers.
(A)
There exist 𝑴∈ℝ3×3,𝒑∈ℝ3,and 𝒒∈ℝ3 such that 𝑴𝐱=𝒑 has a unique solution and M𝐱=𝒒 has infinite solutions.
(B)
There exist 𝑴∈ℝ3×3,𝒑∈ℝ3,and 𝒒∈ℝ3 such that 𝑴𝐱=𝒑 has no solutions and M𝐱=𝒒 has infinite solutions.
(C)
There exist 𝑴∈ℝ2×3,𝒑∈ℝ2,and 𝒒∈ℝ2 such that 𝑴𝐱=𝒑 has a unique solution and M𝐱=𝒒 has infinite solutions.
(D)
There exist 𝑴∈ℝ3×2,𝒑∈ℝ3,and 𝒒∈ℝ3 such that 𝑴𝐱=𝒑 has a unique solution and M𝐱=𝒒 has no solutions

Let ℝ be the set of real numbers, 𝑈 be a subspace of ℝ3 and 𝑴∈ℝ3×3 be the matrix corresponding to the projection on to the subspace 𝑈.
Which of the following statements is/are TRUE?
(A)
If 𝑈 is a 1-dimensional subspace of ℝ3, then the null space of 𝑴 is a
1-dimensional subspace.
(B)
If 𝑈 is a 2-dimensional subspace of ℝ3, then the null space of 𝑴 is a
1-dimensional subspace.
(C)
𝑴2= 𝑴
(D)
𝑴3= 𝑴

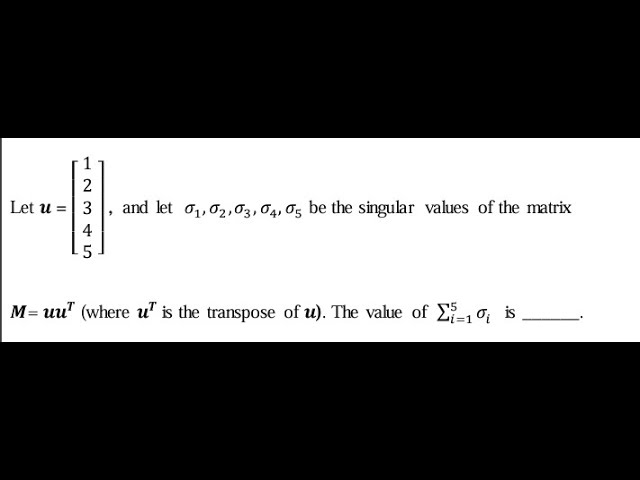
Let 𝒖 = [ 1 2345] , and let 𝜎1,𝜎2,𝜎3,𝜎4,𝜎5 be the singular values of the matrix
𝑴= 𝒖𝒖𝑻 (where 𝒖𝑻 is the transpose of 𝒖). The value of Σ𝜎𝑖 5
𝑖=1 is ______.

[SHIVAKUMAR-xbru]

good evening sir! In question no 61 you said that sum of singular values is equal to trace of M (M is symmetric and +ve semidefinite)and also we know that sum of eigen values is also trace M .how the eigen values and singular values are equal ?

[showkatahmad]

Good morning sir, after a long time with good topic