301.10H Applying the First Isomorphism Theorem

Показать описание

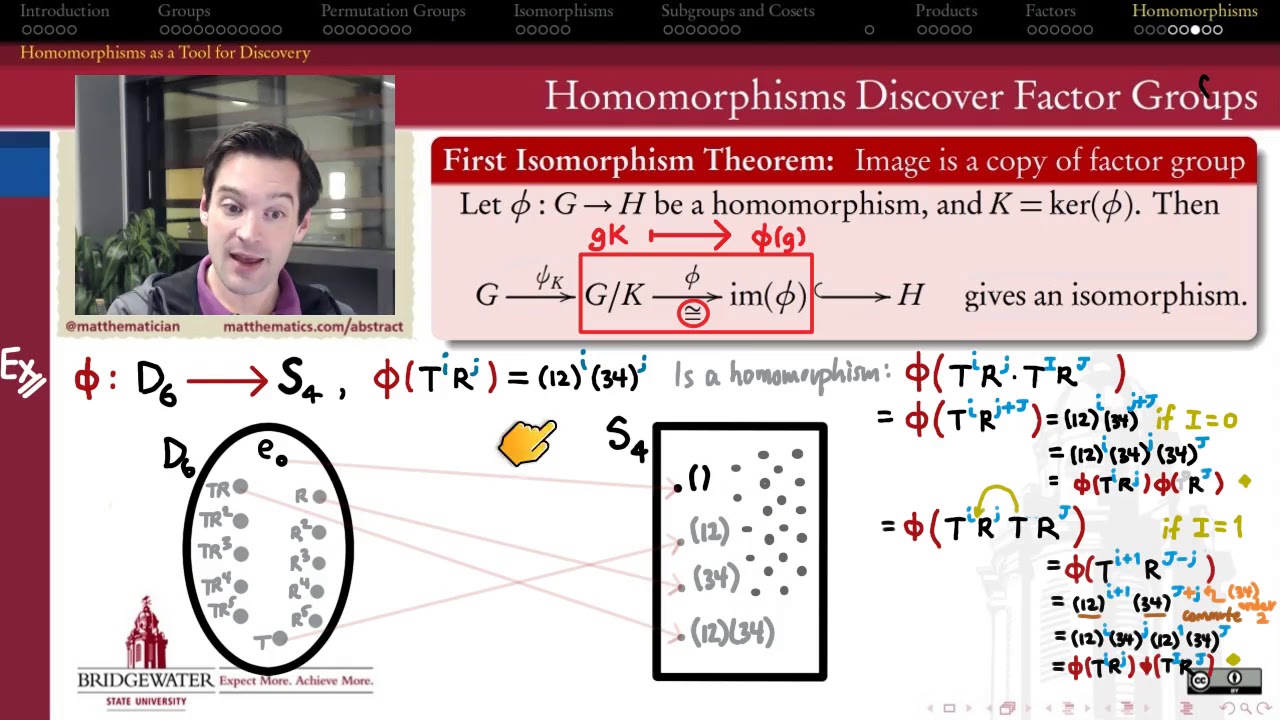
The first isomorphism theorem helps us "whittle down" a homomorphism to discover an isomorphism within. It does this by giving us a normal subgroup of G (the kernel of the homomorphism) and also telling us what the factor group by that normal subgroup is (it's isomorphic to the image of the homomorphism). Here's an example using dihedral and symmetric groups.

Рекомендации по теме

Комментарии

Applying the first? More like "Amazing videos that quench a thirst"...of knowledge! 👍

PunmasterSTP

How do we know to map to the transpositions (12) and (34). I kinda missed the point, how to determine to pick which elements in S4.

krumpy

The factor group is dual to the image -- the 1st isomorphism theorem.
Injective is dual to surjective synthesizes bijective or isomorphism.
Domains are dual to co-domains.
Same is dual to different -- removing the differences via projection/contraction synthesizes isomorphism or duality!

hyperduality

301.10H Applying the First Isomorphism Theorem

Advanced Calculus: contact geometry, Reeb field etc. 12-8-17, part 1

Constantin Teleman: Quantization commutes with reduction: The quantum GIT conjecture #ICBS2024

45 Sampling Methods (10.3-10.4)

301.3B Cyclic Groups and Subgroups

XI - Chemistry Reduced Syllabus (Complete Lectures in Description)

301.8B Defining, Motivating, and Modeling Direct Products

Isomerism || Different types of structural isomerism || chemistry

Video_103: Cut Set Matrix and Fundamental Cut Set Matrix of a Graph

probability Distributions | 4th sem m4 module 4 | vtu 4th sem model question paper solution

De-Broglie Relationship (Detailed Overview) | Atomic Structure | Lecture 5 | Z1 Tutorials

2016Waseda VC&QT 5/17, Don Zagier, Quantum invariants, q-series and Bloch groups

CS 302 Discrete Structure Introduction on Discrete Structure B.Tech III Sem, II Year (CS)