filmov
tv
How to use generating functions with integer partitions -- Number Theory 30

Показать описание
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
A brief introduction to generating functions
GENERATING FUNCTIONS - Discrete Mathematics
Olympiad level counting (Generating functions)
Generating Functions and Counting With Them - An Intro
Generating Functions - Functions - Discrete Mathematics
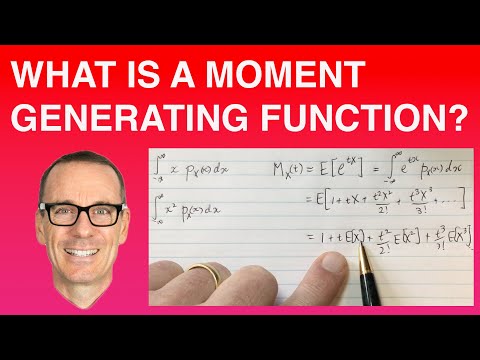
What is a Moment Generating Function (MGF)? ('Best explanation on YouTube')
How To Solve Recurrence Relations With Generating Functions
Generating function explained
Combinatorics 9.1 Generating Functions - Introductory Examples
RECURRENCE RELATIONS using GENERATING FUNCTIONS - DISCRETE MATHEMATICS
How to use generating functions with integer partitions -- Number Theory 30
The beauty of generating functions. Why this fraction gives Fibonacci sequence ?
Counting with Calculus: The Magic of Generating Functions
How you can solve dice puzzles with polynomials
Generating Functions -- Number Theory 29
Moment generating functions -- Example 1
Moment Generating Functions (Part 1)
Generating Functions | Part 1
Generating Functions: An Introduction - Part 1
Discrete Math II - 8.4.5 Solve Counting Problems with Generating Functions
Use generating functions to solve the recurrence relation with initial conditions
Discrete Math II - 8.4.6 The Explicit Form of a Generating Function
Finding Coefficient in an expansion| Generating Functions
Discrete Math II - 8.4.1 Readiness for Generating Functions: Model with Generating Functions
Комментарии
 0:07:01
0:07:01
 0:18:41
0:18:41
 0:34:36
0:34:36
 0:14:59
0:14:59
 0:11:41
0:11:41
 0:08:51
0:08:51
 0:07:42
0:07:42
 0:12:59
0:12:59
 0:13:54
0:13:54
 0:28:40
0:28:40
 0:29:45
0:29:45
 0:04:15
0:04:15
 0:21:52
0:21:52
 0:10:02
0:10:02
 0:24:41
0:24:41
 0:05:19
0:05:19
 0:08:25
0:08:25
 0:31:07
0:31:07
 0:07:01
0:07:01
 0:27:16
0:27:16
 0:09:53
0:09:53
 0:21:25
0:21:25
 0:11:33
0:11:33
 0:13:51
0:13:51