Introduction to analytic number theory 10: The functional equation for zeta function

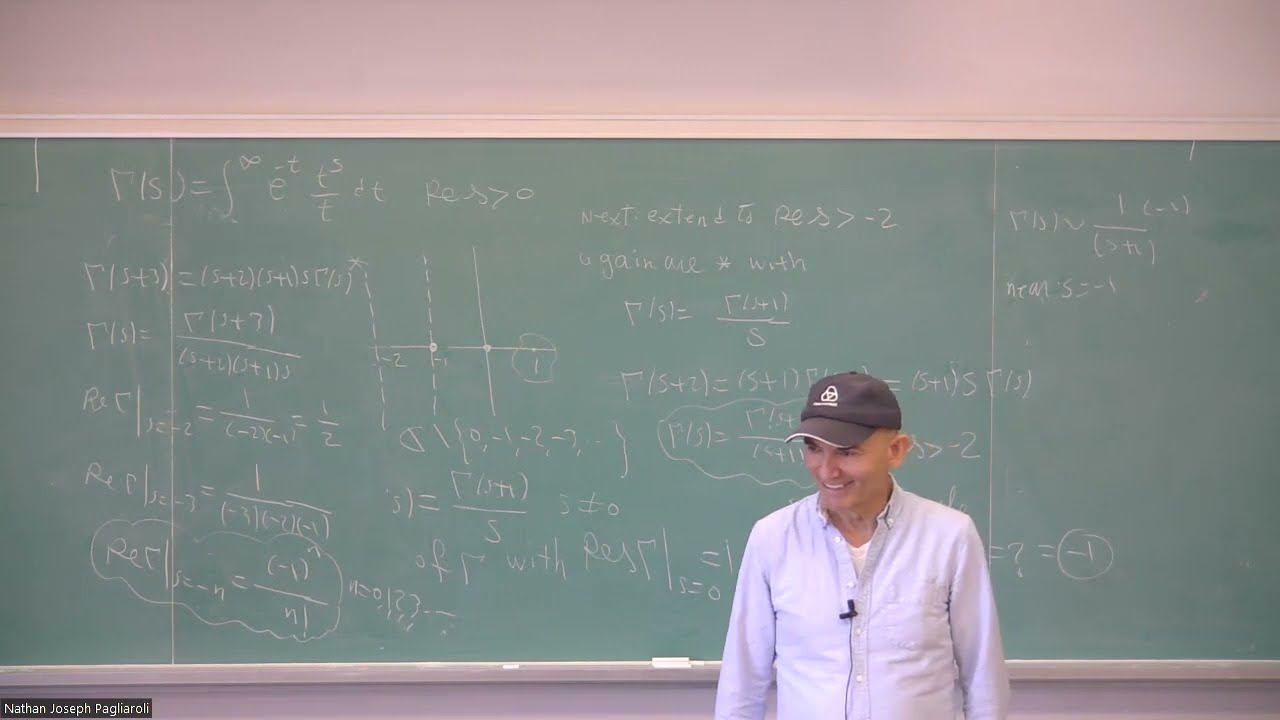
Показать описание

Lecture 10 of my graduate course, Introduction to analytic number theory, at the University of Western Ontario, May-June 2023. Analytic continuation and functional equation of the Riemann zeta function (Riemann's second proof): Mellin transform, theta function and modularity.
00:30 analytic continuation of Gamma function
10:00 poles and residues of Gamma function
37:00 completed zeta function
38:27 theta function, zeta via theta, Mellin transform
47:05 modularity of theta
49:00 analytic continuation and functional equation of zeta functions

Masoud Khalkhali

Рекомендации по теме

Комментарии

I love this video; it's very easy to understand and well-explained. Is there an easy way to demonstrate that the Gamma function cannot be zero?

RSLT

Introduction to analytic number theory 10: The functional equation for zeta function

Analytic Number Theory: Introduction to analytic number theory - 4th Year Student Lecture

Analytic Number Theory

Analytic number theory: introduction

Andrew Granville - 1/3 The pretentious approach to analytic number theory

Analytic Number Theory: Dirichlet series - Oxford Mathematics 4th Year Student Lecture

Analytic number theory

Introduction to Analytic Number Theory

Introduction to analytic number theory 8

Intro to Analytic Number Theory and PNT!

Introduction to analytic number theory 17: Class number formula examples

Introduction to analytic number theory 4: Dirichlet series

Introduction to Basic Analytic Number Theory by Aatman Supkar

Analytic Number Theory with Sage - Kamalakshya Mehatab

Introduction to analytic number theory 13: Riemann-von Mangoldt explicit formula

Introduction to analytic number theory 12: special values of zeta function

Introduction to analytic number theory 10: The functional equation for zeta function

Algebraic number theory - an illustrated guide | Is 5 a prime number?

Damaris Schindler: Interactions of analytic number theory and geometry - lecture 1

Introduction to analytic number theory 7

Introduction to analytic number theory 1: Euler, zeta function, and distribution of primes.

Lecture 1, Analytic Number Theory Rutgers Math 572 Prof. Kontorovich, 1/21/2022

Introduction to analytic number theory 14: A quick summary of Riemann's 1859 paper

Introduction to analytic number theory 19: Functional equation for Dirichlet L-functions

Analytic Number Theory Lecture 01