filmov
tv
Product & Quotient of Polar Complex Numbers
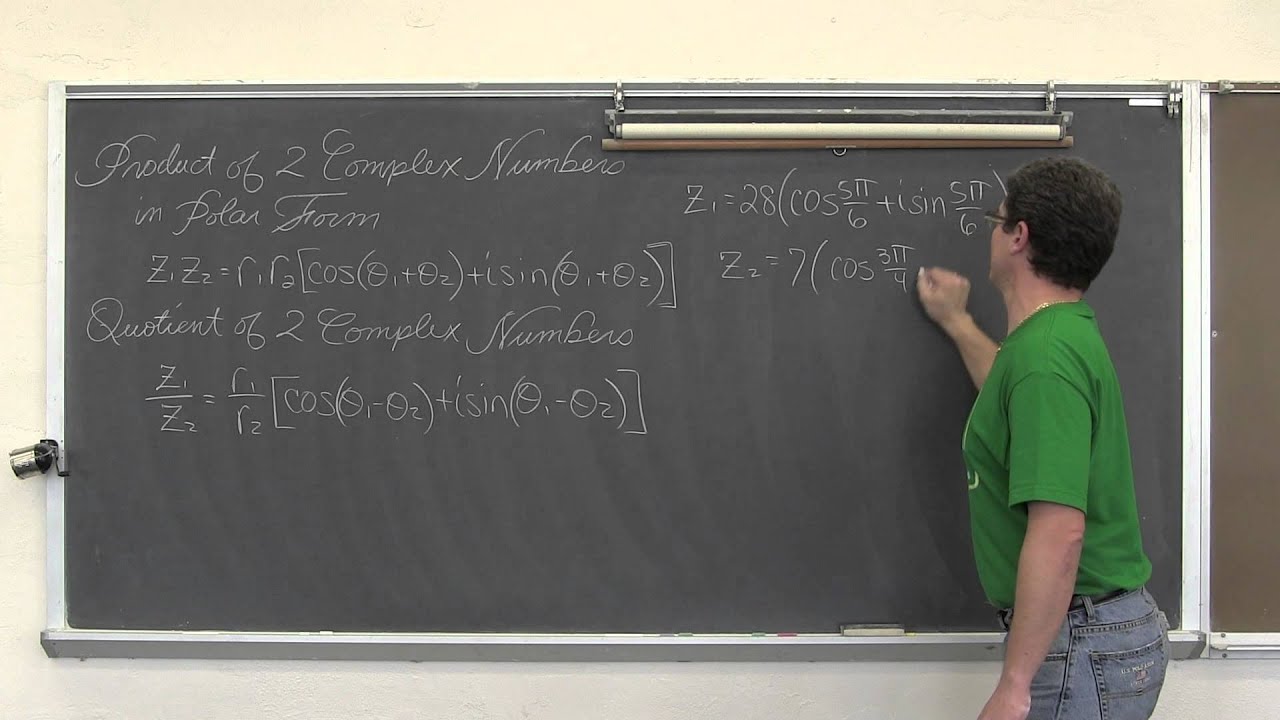
Показать описание
Product & Quotient of Polar Complex Numbers
Finding the Product and Quotient of Complex Numbers in Polar Form
Find the quotient of complex numbers in polar form
Product and Quotient Formulas of Complex Numbers in Polar Form
Trig: Product and quotient of two complex numbers in polar form
The Quotient Theorem of Polar Complex Numbers
Find the quotient of complex numbers in polar form
Complex Polar Product Quotient Power
Trig: Product and Quotient of complex numbers in polar form
Product Quotient Polar Form 1
Trig - 8.3 - The (Complex Polar) Product & Quotient Theorems - Examples
HSC Maths Ext2 - Complex Numbers - Product and Quotient in Modulus-Argument Form
PreCalculus - Polar Coordinates (30 of 35) Complex Numbers: Proof of the Product Rule
M98s0803 Product and Quotient of polar
408 polar product and quotient rule
Ch. 8.3 The Product and Quotient Theorems
Product and Quotient of Complex Numbers in Polar Form (111.8.5b)
Product and Quotient Proof for Complex numbers
Lecture 09 Product and Quotient Rule of Polar Form
Product Quotient Polar Form 2
HSC Maths Ext2 - Complex Numbers - Product and Quotient in Modulus-Argument Form Example
Product and Quotient Theorem for Complex Numbers
Given polar complex numbers, find product and quotient
Find the product and quotient of complex numbers
Комментарии
 0:07:20
0:07:20
 0:05:29
0:05:29
 0:01:25
0:01:25
 0:25:43
0:25:43
 0:03:54
0:03:54
 0:03:33
0:03:33
 0:01:42
0:01:42
 0:09:59
0:09:59
 0:02:51
0:02:51
 0:12:18
0:12:18
 0:17:08
0:17:08
 0:12:29
0:12:29
 0:04:40
0:04:40
 0:05:13
0:05:13
 0:10:43
0:10:43
 0:06:49
0:06:49
 0:04:22
0:04:22
 0:08:17
0:08:17
 0:07:33
0:07:33
 0:14:52
0:14:52
 0:04:32
0:04:32
 0:38:33
0:38:33
 0:03:04
0:03:04
 0:03:50
0:03:50