Let 6x6 Sudoku be Your Friend!

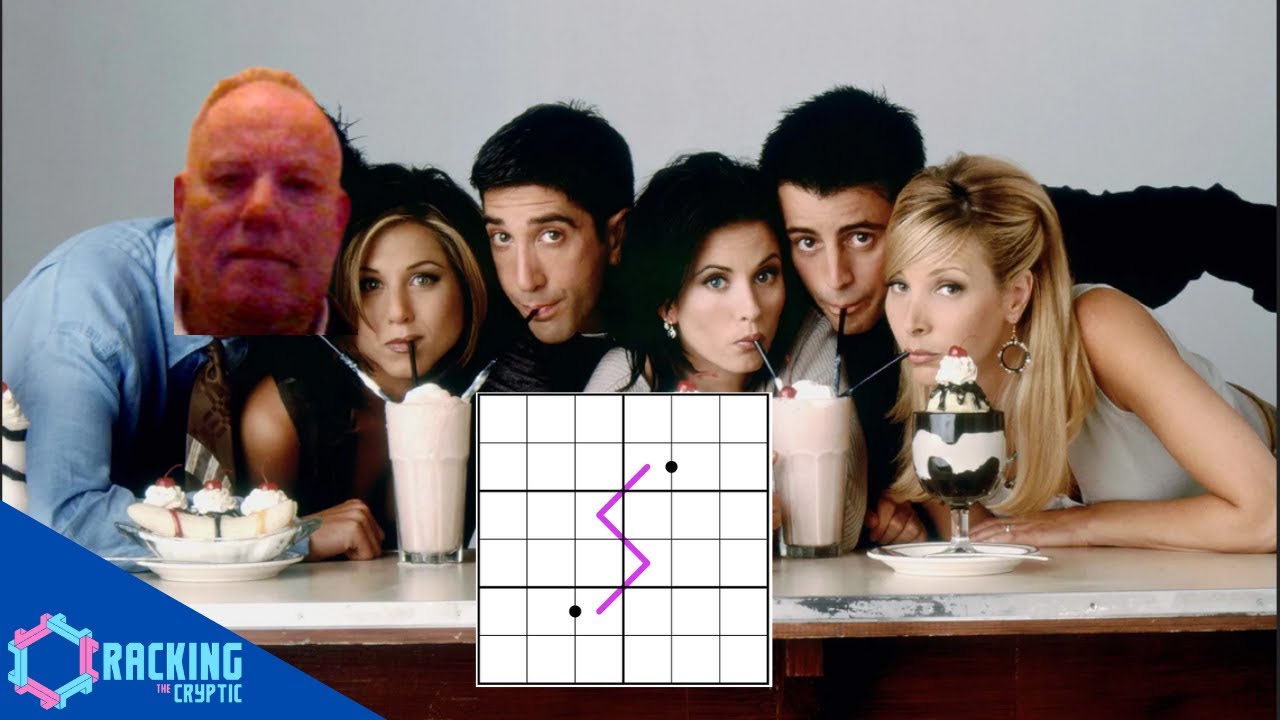
1-6 must be placed in each row, column and 3x2 box. A friendly cell contains a digit equal to its row, column, or 3x2 box number. Each box contains 3 friendly cells. The purple line contains a set of non-repeating consecutive digits in any order. Digits in cells connected by a black dot have a 1:2 ratio.

▶ SUDOKU PAD - Our New App ◀ NOW AVAILABLE - You can now input your own classic sudoku puzzles into our software using our new App! The app also comes with 12 handmade puzzles from us and it is NOW AVAILABLE at these links:
On Amazon: Search for “SudokuPad”

▶ OUR PATREON PAGE – JOIN THE COMMUNITY, SUPPORT US AND TRY OUR PUZZLE HUNTS◀

▶Our merchandise is all here: ◀

▶ CTC FAN DISCORD SERVER◀

▶ OUR BACK CATALOGUE – ALL CATEGORISED WITH LINKS!◀

▶ WHY NOT TRY OUR CLASSIC SUDOKU APP? ◀

▶ OUR KILLER SUDOKU IS ALSO AVAILABLE◀

▶ YOU CAN ALSO TRY OUR SANDWICH SUDOKU APP ◀

▶ TRY OUR CHESS SUDOKU APP◀

▶ HERE’S WHERE YOU CAN TRY OUR MIRACLE SUDOKU APP◀

▶ THERMO SUDOKU is available too!◀

▶ YOU CAN ALSO TRY OUR ARROW SUDOKU APP ◀ Here are the links:

▶SEND US PUZZLES TO SOLVE/CONTACT US◀
Snailmail: Mark Goodliffe, Box 102, 56 Gloucester Road, London SW7 4UB

▶FOLLOW US◀
Twitter: #crypticcracking
@crypticcracking

▶Music◀
Tim McCaskey (Guitar) or Lucy Audrin (Piano) or Riffclown plays Mozart's Sonata no 16 ("Sonata Facile")
▶SOFTWARE◀
Play the puzzle in the video by clicking the link under the video (above). Thanks to Sam Cappleman-Lynes and Sven Neumann for their work.
▶Logo Design◀
Melvyn Mainini

▶Opening Credits Design◀
Joel Blundell

▶ABOUT US◀
Hi! We're Simon Anthony and Mark Goodliffe, two of the UK's most enthusiastic puzzle solvers. We have both represented the UK at the World Sudoku Championships and the World Puzzle Championships. We're also "cryptic crossword" aficionados. Mark is the twelve-time winner of The Times championship and Simon is the former record holder for most consecutive correct solutions to The Listener crossword. We hope we can help your puzzle solving while also introducing you to some of the world's best puzzles.

Thank you for watching!
Simon and Mark

[thoughtbyte]

Thank you so much for featuring this one! It was a wonderful solve and followed the intended path to the tee. I'm so glad you featured it today after Phistomefel's 7x7, I think it showcases the beauty of minimal, smaller grids. Thank you, cheers!

[BroadwayGaming]

I don't do many puzzles because they're too hard but did this one on my own. Absolutely amazing logic, I love it.

[Playmaker]

I have to say, this is such a satisfying puzzle, and the way Mark delivered the logical thoughts throughout the solve here makes the puzzle even better :)

[G.Aaron.Fisher]

One of the very few instances where I've beaten Mark's time, and ironically it was because of all the pencilmarking. I straight away entered 123456 into every box, and took out the digits as I made deductions. Flew through the puzzle in 11 minutes.

[teliots]

I liked this ruleset. The restriction on overlapping friendly numbers is not obvious on the first read.

[srwapo]

14:38, took a few minutes to realize that the max number of friendly cells available in the puzzle equaled what the rules called for, therefore the friendly cell couldn't satisfy more than one requirement (eg, 1 can't be in r1c2 because it satisfy the row number and box number) or I'd run out of friendly cells. Neat negative constraint.

[richbaker]

11:26 for me. Nice little puzzle. The realization that all of the friendly cells had to be distinct was immediately clear, so that helped!

[kielblanton]

This ruleset was fantastic. Very different from any other puzzle I've played. I wish Mark had noticed early on that each friendly cell was also restricted by column (i.e. at 6:57 when he pencil marks 2s, r2c2 can't be a 2, and then r2c1 can't be a 1, etc). It uncovers the beauty of the logic a little faster, and forms a 34 pair in the middle rows, which I thought was a very clever result of the Renban logic.

[adrianhead]

Completed in 6m53s - it's simple when you realise that the box number is in the box by default AND cannot also appear in its row or column (that rules out four positions in each box)

[istvanmagi]

Let's construct an impossible statement!
"Today Simon is doing a Phistomefel construct, and Mark is doing a 6x6 puzzle. And Simon's one is much easier."
And it's true.

[Domancave]

That was a really fun puzzle. I don’t think I could have figured it out myself but I love how quickly you worked it out

[Coyotek]

10:29 ... I love puzzles like this. Friendship is Sudoku Magic! :)

Nice puzzle!

[amysteele]

Stunningly beautiful puzzle, with an almost deceptively simple rule set. Nicely solved

[inspiringsand]

Rules: 01:31
Let's Get Cracking: 04:11
Mark's time: 13m36s
Puzzle Solved: 17:47

And how about this video's Simarkisms?!
Symmetry: 8x (01:10, 01:16, 01:25, 03:59, 09:15, 16:28, 17:19, 17:40)
Hang On: 5x (02:50, 02:50, 06:51, 11:56, 13:12)
Clever: 3x (10:39, 12:57, 17:49)
Ah: 3x (07:27, 10:35, 15:12)
Sorry: 2x (02:26, 05:36)
Brilliant: 2x (10:44, 18:26)
By Sudoku: 2x (14:31, 16:39)
Fascinating: 1x (04:04)
Incredible: 1x (18:35)
I've Got It!: 1x (10:35)
Obviously: 1x (01:59)
Progress: 1x (08:30)
Wow: 1x (12:17)

Most popular number(>9), digit and colour this video:
Eighteen (2 mentions)
One (74 mentions)
Black (10 mentions)

Antithesis Battles:
Even (2) - Odd (0)
Black (10) - White (0)
Column (32) - Row (31)

FAQ:
Q1: You missed something!
A1: That could very well be the case! Human speech can be hard to understand for computers like me! Point out the ones that I missed and maybe I'll learn!
Q2: Can you do this for another channel?
A2: I've been thinking about that and wrote some code to make that possible. Let me know which channel you think would be a good fit!

[przemekmajewski]

This was VERY unexpected and VERY good quality! Nice 20 min mind game for me.

[SSGranor]

Early in the video, Mark talks about the idea that puzzles with symmetric clues need to have the symmetry broken in some way. While this is true of 9x9 sudoku (when talking about rotational symmetry, at least), it is not necessarily the case for 6x6. Although in this puzzle, the non-repeat rule for renbans does mean that the symmetry must be broken, it is possible to construct a totally rotationally symmetric 6x6 puzzle, because there are valid completed grids with rotational symmetry. (The problem in a 9x9 is that box 5 can't be rotationally symmetric without repeating digits; but, since 6x6 sudoku has no middle box, that's not a problem.)

[jwolfe]

14:14 "Well it can't be an overlapping 1, " but doesn't remove the 1 pencil mark.

I vaguely remembered from the last friendly cell puzzle on this channel that the friendliness couldn't overlap, but it took me a long time to remember how to prove it. It's not always true for friendly cell puzzles, just for ones with rule sets like this one.

[GuilhermeCarvalhoComposer]

Took me a hot minute to convince myself there couldn't indeed be overlaps. For some reason that was FAR from obvious to me. Great puzzle, though, I really enjoyed it and the solve. :)

[RD-nkos]

Another day where I solved both puzzles before they premiered on the channel! The break-in is tricky, but it’s smooth sailing once you figured out the break-in.

[LithmusEarth]

I just quickly pulled up the puzzle and put every friendly number in, so i labeled every row through their numbers, every column, and each box, and i found the first box and the last box are restricted by the 3 cell constraint. they have to contain 123 and 456 respectively, which meant column 3's 3 is in box 1 and and column 4's 4 is in box 6. which i'm sure he'll find, i'm going to give up on the puzzle, just wanted to test that out myself.
Also to your point at 5 minutes in, you can actually get 5 friendly numbers in box 2 if you wanted. 413 along top and 256 along the bottom, results in 4, 1, 2, 5, and 6 all being friendly numbers. although technically you could get that 2 moved around since it's box 2 i forgot. but oh well.
9 minutes in I get it. there are 6 rows, 6 columns and 6 boxes, and the puzzle requires 18 friendly cells distributed around 3 per box... so yeah you can't have any 1s in box 1 in a row 1 or column 1 giving them only 2 spots... maybe I should go back to the puzzle that's a crucial piece of logic.
Your 11 minute was another piece of logic i didn't see that i should be able to finish the logic, because of the lack of 3s and 4s on it in the middle i should have seen where that was going to lead.

31 Minutes.