Genetics. Law of probability: rules of multiplication and addition.

If A and B are events, the probability of obtaining either of them is:
P(A or B) = P(A) + P(B) - P(A and B)

If the events A and B are mutually exclusive( that is, if both events cannot occur simultaneously), the last term [P(A and B)] will be 0. Thus the addition rule with mutually exclusive events becomes:

P(A or B) = P(A) + P(B)

Example:

Suppose a high school consists of 25% juniors, 15% seniors, and the remaining 60% is students of other grades. The relative frequency of students who are either juniors and seniors is 40%. We can add the relative frequencies of juniors and seniors because no student can be both junior and senior.

P(J or S) = 0.25 + 0.15
which equals 0.40

The above formula can be expanded to consider more than two exclusive events:

P(A or B or C or D... or Z) = P(A) + P(B) + P(C) + ... + P(Z)

In the preceding section, we were concerned with determining the probability of obtaining one event or another based upon a single draw. Now we will learn how events A and B both occur simultaneously.
Example:

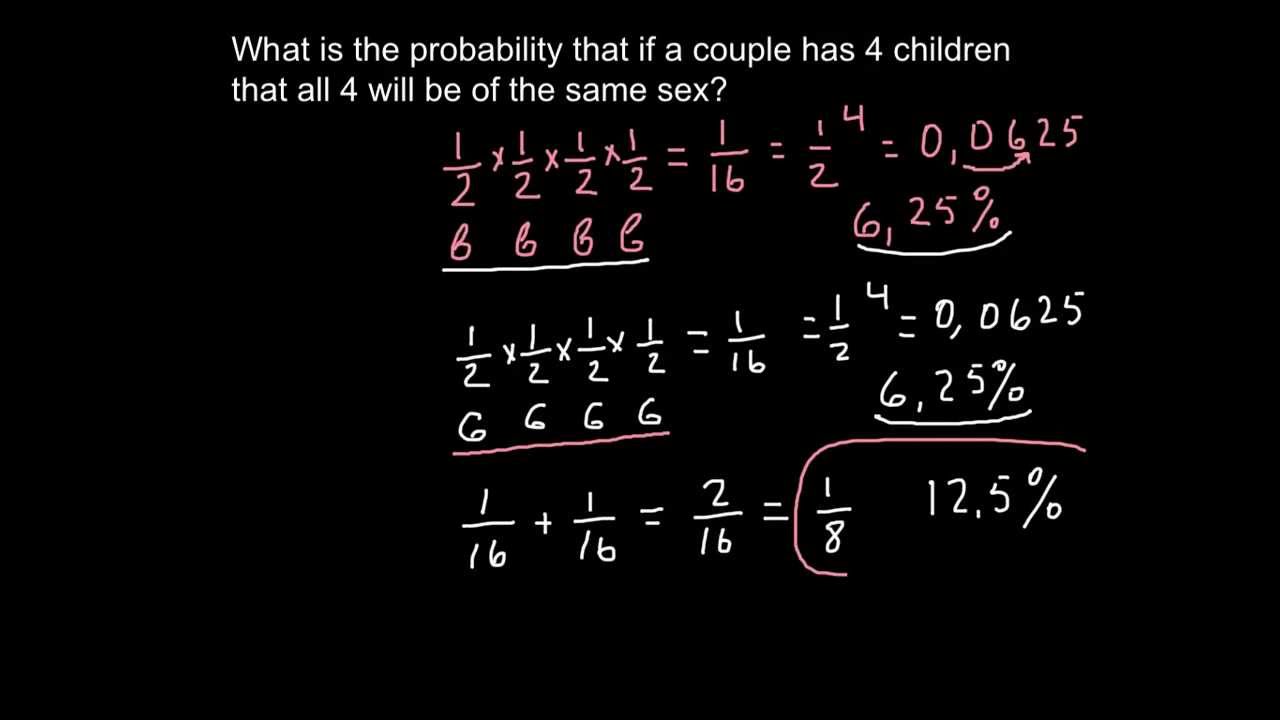
What is the probability that two tails occurs when two coins are tossed? Let A represent the occurrence of a tail on the first coin and B represent the occurrence of a tail on the second coin. In this example, the occurrence of A is not dependent upon the occurrence of B and vice versa. Events A and B are said to be Independent. That is, the outcome of the first toss has no effect on the outcome of the second toss. The probability of the simultaneous occurrences of two independent events is the product of the probabilities of each event:

P(A and B) = P(A) . P(B)
P(A) = 1/2
P(B) = 1/2
P(A and B) = 1/2 . 1/2 = 1/4

Example:

Suppose we have two dice. A is the event that 4 shows on the first die, and B is the event that 4 shows on the second die. If both dice are rolled at once, what is the probability that two 4s occur?

P(A) = 1/6
P(B) = 1/6
P(A and B) = P(A) . P(B) = 1/6 . 1/6 = 1/36

P(A and B) = P(A) . P(B)
The above formula can be expanded. If A , B , C , ... , Z are independent events, then:

P(A and B and C and ... and Z) = P(A) . P(B) . P(C) ... P(Z)

[priyankadube]

Wow sir your all video regarding genetics are awesome, Thank you so much for your kindness and help.

[poeticallyinclined]

In pea plants, yellow seeds
(G) are dominant to green seeds (g). A true-breeding plant with yellow seeds is
crossed to a true-breeding plant with green seeds. The F1 progeny
for this cross are bred with each other. You pick 4 random seeds and plant
them. What is the probability that all the first three seeds produce yellow
plants and they fourth one produces green seeds in that order?