Abstract Alg, Lec 21A: Internal Direct Products, Homomorphisms, Kernels, First Isomorphism Theorem

Abstract Algebra, Lecture 21A.

(0:00) Lecture plan.
(0:29) Exam 2 next class period.
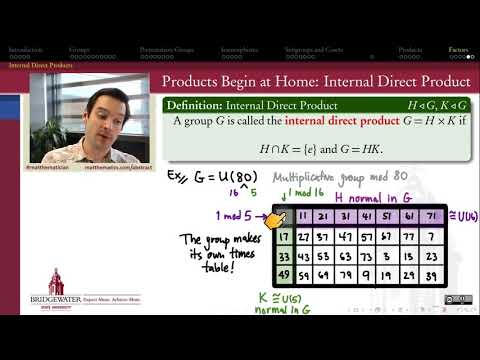
(1:35) Internal direct product of two normal subgroups and the proof that it is isomorphic to the corresponding external direct product.
(18:42) Definition of a group homomorphism and the kernel of a group homomorphism (with comments linear transformations and null spaces and about preimage (inverse image) notation).
(22:15) Kernels are normal subgroups and cosets of the kernel are preimages of individual elements in the image ("range"), and a verification.
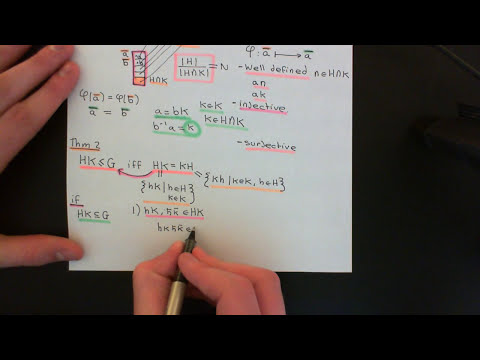
(29:19) First Isomorphism Theorem and the idea of its proof.
(35:51) Normal subgroups are kernels.

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.

[tylergonzales]

So helpful, the isomorphism theorems I found confusing at first, and I always find these lectures very helpful!

[saurabhdeep]

Sir i wanna ask what are those elements which have the order 10 in Z100+Z25

[ritesharora]

When will full course be uploaded? Will you upload graduate courses too ? Thanks