AP Precalculus Practice Test: Unit 1 Question #18 Understand a Limit as x Approaches Infinity

My AP Precalculus Practice Tests are carefully designed to help students build confidence for in-class assessments, support their work on AP Classroom assignments, and thoroughly prepare them for the AP Precalculus exam in May.

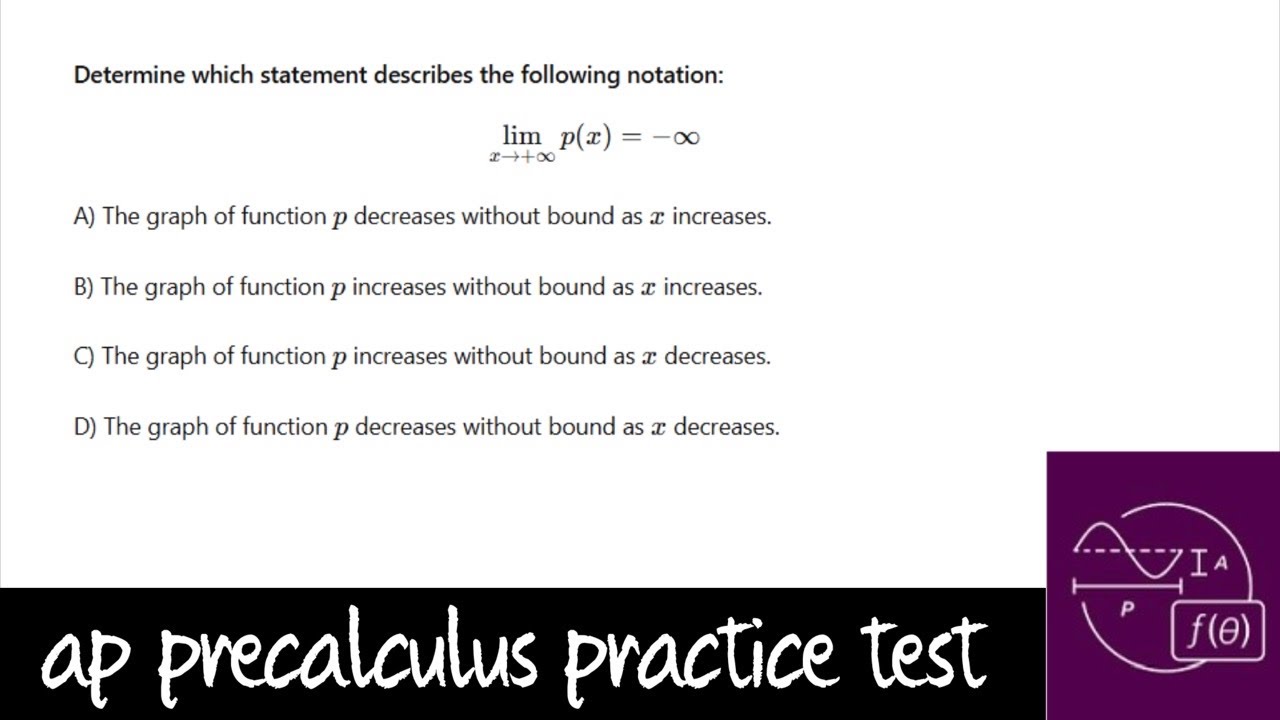
In AP Precalculus, Unit 1 introduces students to the concept of limits, particularly as \( x \) approaches infinity. Question #18 likely assesses students' understanding of how to determine the behavior of a function as \( x \) increases or decreases without bound.

Here’s a step-by-step approach to understanding limits as \( x \) approaches infinity:

1. **Identify the Function's Form**: Look at the given function, which might be a polynomial, rational function, exponential function, or another type. The behavior as \( x \to \infty \) often depends on the highest power of \( x \) in the function.

2. **Analyze Leading Terms**:
- **For Polynomial Functions**: The behavior as \( x \to \infty \) is governed by the leading term (the term with the highest power of \( x \)). For example, if \( f(x) = 3x^4 - 2x^2 + x \), as \( x \to \infty \), \( f(x) \approx 3x^4 \), so \( f(x) \to \infty \).
- **For Rational Functions**: Compare the degrees of the numerator and the denominator:
- If the degree of the numerator is higher, \( f(x) \to \infty \) or \( f(x) \to -\infty \).
- If the degrees are the same, the limit as \( x \to \infty \) is the ratio of the leading coefficients.
- If the degree of the denominator is higher, \( f(x) \to 0 \).
- **For Exponential Functions**: Exponentials like \( e^x \) or \( 2^x \) grow faster than polynomials, so if the exponent is positive, \( f(x) \to \infty \); if negative, \( f(x) \to 0 \).

3. **Determine the Limit**: Use the insights above to find the limit as \( x \to \infty \) based on the dominant terms.

### Example:
For \( f(x) = \frac{5x^2 + 3x}{2x^2 + x + 1} \):

- The leading terms in both the numerator and denominator are \( 5x^2 \) and \( 2x^2 \), respectively.
- As \( x \to \infty \), the limit is the ratio of these leading coefficients:
\[
\lim_{x \to \infty} f(x) = \frac{5}{2}.
\]

This approach helps students understand how to evaluate limits as \( x \) approaches infinity by focusing on the dominant terms of the function.

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths #math #shorts #funny #help #onlineclasses #onlinelearning #online #study