filmov
tv
Solve: dy/dx=(x+2y-3)/(2x+y-3)|| Homogeneous Differential Equation || Seperable ODE

Показать описание
In this video, we will solve the ODE dy/dx=(x+2y-3)/(2x+y-3) . This is a first-order linear differential equation. First we will make the substitution X=x-1, Y=y-1. After the substitution the given ode will become homogeneous differential equation. Then again we make a substitution Y=vX and convert the equation into a seperable differential equation. Here, we try to provide a step-by-step solution of the given problem.
If you find this video useful, then please like comment and subscribe. Thank you for watching.
Related Keywords:
#integratingfactor
#homogeneous differential equation
#homogeneousequation
#seperableode
#firstorderode
#ODE
#linearode
#differentialEquation
#maths
#physics
#appliedmaths
#linear
If you find this video useful, then please like comment and subscribe. Thank you for watching.
Related Keywords:
#integratingfactor
#homogeneous differential equation
#homogeneousequation
#seperableode
#firstorderode
#ODE
#linearode
#differentialEquation
#maths
#physics
#appliedmaths
#linear
Solve: dy/dx=(x+2y-3)/(2x+y-3)|| Homogeneous Differential Equation || Seperable ODE
Solve: dy/dx = x+2y-3/2x+y-3 | Reducible to homogeneous differential equations
Q136 Solve dy/dx = (x+2y-3)/(2x+y-3)
Solve: dy/dx=x^2y/(x^3+y^3)|| Homogeneous Differential equation ||Step-by-step solution || ODE
Solve dy/dx = (x+2y-3)/(2x+y-3).
2ndsem Solve(2x+y-3)dy=(x+2y-3)dxdifferentialEquations#unit 1questons
Q145 dy/dx = (2x-y+1)/(x+2y-3)
Q142 (New) dy/dx = (x+2y+3)/(2x+3y+4)
Solve the differential equation ` (dy)/(dx) = ( x + 2y + 3)/(2x + 3y + 4)`
(2x+2y+3)dy/dx=x+y+1 #NonHomogeneousDifferentialEquations L492 @MathsPulseChinnaiahKalpana
Solve `(dy)/(dx)=(2x-y+1)/(x+2y-3)`
'Solve `(dy)/(dx)=(2x-y+1)/(x+2y-3)`'
Solve `(dy)/(dx)=(x+2y+3)/(2x+3y+4)`
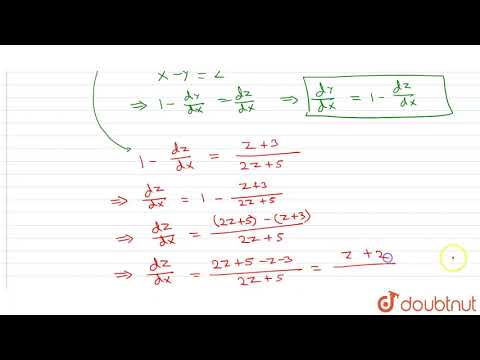
#13 Non Homogeneous differential Equation 1st order and 1st degree | solve dy/dx=(x-y+3)=(2x-2y+5)
Q137 Solve dy/dx + (x-y-2)/(x-2y-3) = 0
Differential Equations Practice #65: (2x + y - 4)dx + (x - 2y + 3)dy = 0
Differentiation vol-2 EXACT EQUATION by Srinivasa rao
(2x-2y+5)dy=(x-y+3)dx | 12 | DIFFERENTIAL EQUATIONS OF THE FIRST ORDER AND FIRST DEGREE | MATHS...
Homogeneous Differential Equation: dy/dx = (x^2 + y^2)/xy
dy/dx=(x-y-3)/(2x-2y+5)
Differential Equations Practice #52: (2x + y - 4)dx + (x - 2y + 3)dy = 0
(2x + y + 3) dx = (2y + x + 1) dy , Solve Non-Homogenous Differential Eq'n @EAG
Solving Non-Exact differential equations: Example 1/5
Solving Differential Equations: x(2y-3)dx+(x^2+1)dy=0
Комментарии
 0:06:29
0:06:29
 0:19:17
0:19:17
 0:21:23
0:21:23
 0:03:28
0:03:28
 0:08:34
0:08:34
 0:00:11
0:00:11
 0:19:20
0:19:20
 0:26:48
0:26:48
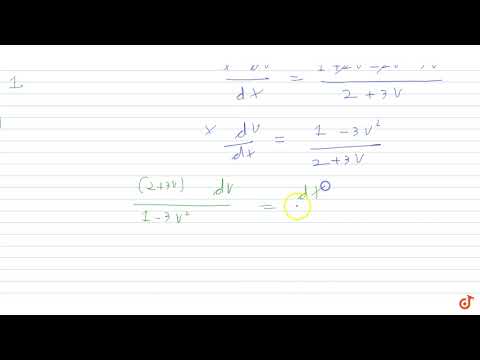 0:10:47
0:10:47
 0:22:24
0:22:24
 0:01:09
0:01:09
 0:02:40
0:02:40
 0:10:41
0:10:41
 0:22:59
0:22:59
 0:22:33
0:22:33
 0:03:16
0:03:16
 0:03:59
0:03:59
 0:07:06
0:07:06
 0:02:55
0:02:55
 0:04:16
0:04:16
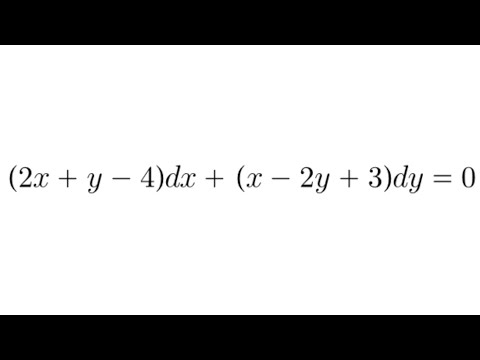 0:06:58
0:06:58
 0:29:52
0:29:52
 0:15:17
0:15:17
 0:01:01
0:01:01