filmov
tv
#07 Laplace's Equation in Two Dimensions | Two dimensional heat flow equation | 2 D heat equation
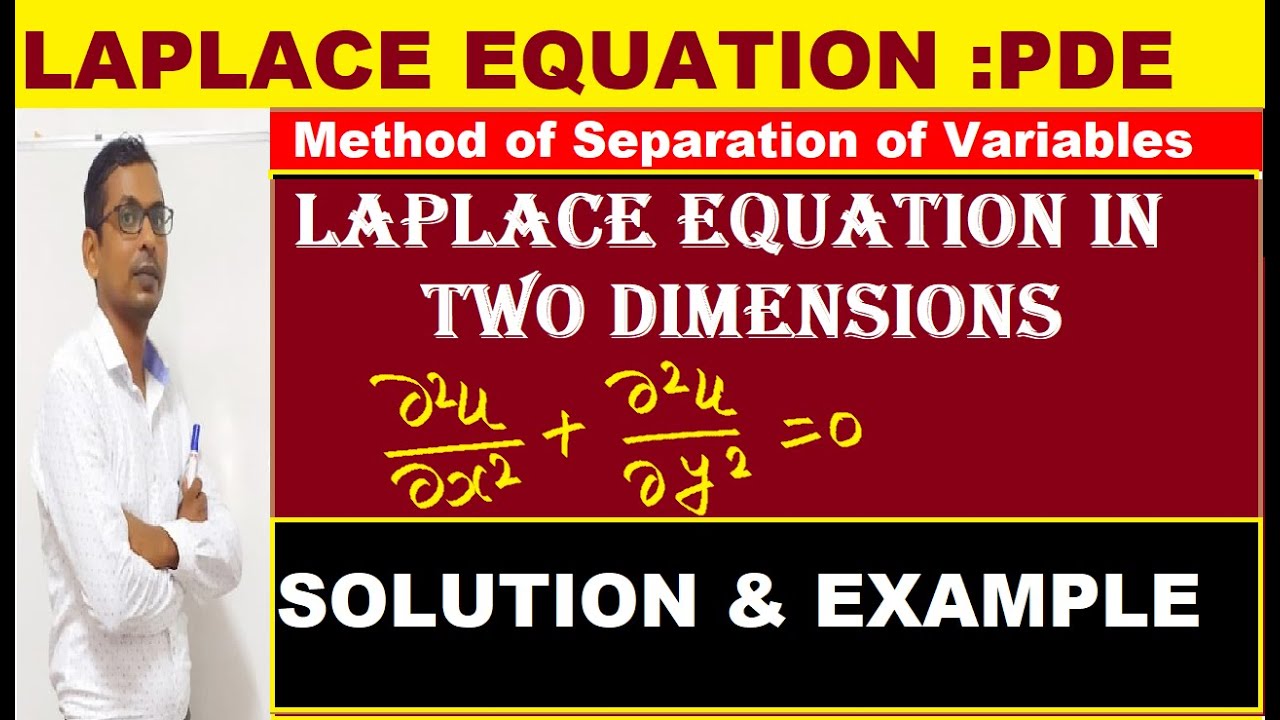
Показать описание
Thanks for watching
In this video we are discussed basic concept laplace equation in two dimensions*. this video helpful to CSIR NET | GATE | IIT JAM | TIFR students. this also helpful to B.Sc. and M.Sc. students. #ammathstutorials #laplaceequation2D #gbtutorials #csirnet_mathematics #gate_mathematics #iitjam_mathematics #abstractalgebra #abeliangroup #nonisomorphicabeliangroup
Follows My Social Network:
1.My web Page (for pdf notes)
2. Facebook Page
3.Twitter
4.Whatsapp channel
TWO DIMENSIONAL HEAT FLOW EQUATION IN HINDI
Laplace's Equation in Two Dimensions in Hindi
heat equation in hindi,
two dimensional heat equation in Hindi
suitable solution of two dimensional heat equation
most suitable solution of heat flow equation
two dimensional heat flow equation in hindi
solution of heat equation by separation of variables
solution of heat flow equation
Solution of two dimensional Heat flow equation in hindi
two dimensional heat equation
most suitable solution of heat equation
most suitable solution of two dimensional heat flow equation
trick to find solution of heat equation
separation of variables method of heat equation
separation of variables method of two dimensional heat equation
separation of variables method of heat flow equation
separation of variables method of two dimensional heat flow equation
Laplace's Equation in Two Dimensions in Hindi
suitable solution of Laplace's Equation in Two Dimensions
most suitable solution of Laplace's Equation in Two Dimensions
solution of Laplace's Equation in Two Dimensions by separation of variables
two dimensional heat equation
most suitable solution of Laplace's Equation in Two Dimensions
trick to find solution of Laplace's Equation in Two Dimensions
separation of variables method of Laplace's Equation
separation of variables method of Laplace's Equation
#ammathstutorials #partialdifferentialequation #onedimensionalheatequation
In this video we are discussed basic concept laplace equation in two dimensions*. this video helpful to CSIR NET | GATE | IIT JAM | TIFR students. this also helpful to B.Sc. and M.Sc. students. #ammathstutorials #laplaceequation2D #gbtutorials #csirnet_mathematics #gate_mathematics #iitjam_mathematics #abstractalgebra #abeliangroup #nonisomorphicabeliangroup
Follows My Social Network:
1.My web Page (for pdf notes)
2. Facebook Page
3.Twitter
4.Whatsapp channel
TWO DIMENSIONAL HEAT FLOW EQUATION IN HINDI
Laplace's Equation in Two Dimensions in Hindi
heat equation in hindi,
two dimensional heat equation in Hindi
suitable solution of two dimensional heat equation
most suitable solution of heat flow equation
two dimensional heat flow equation in hindi
solution of heat equation by separation of variables
solution of heat flow equation
Solution of two dimensional Heat flow equation in hindi
two dimensional heat equation
most suitable solution of heat equation
most suitable solution of two dimensional heat flow equation
trick to find solution of heat equation
separation of variables method of heat equation
separation of variables method of two dimensional heat equation
separation of variables method of heat flow equation
separation of variables method of two dimensional heat flow equation
Laplace's Equation in Two Dimensions in Hindi
suitable solution of Laplace's Equation in Two Dimensions
most suitable solution of Laplace's Equation in Two Dimensions
solution of Laplace's Equation in Two Dimensions by separation of variables
two dimensional heat equation
most suitable solution of Laplace's Equation in Two Dimensions
trick to find solution of Laplace's Equation in Two Dimensions
separation of variables method of Laplace's Equation
separation of variables method of Laplace's Equation
#ammathstutorials #partialdifferentialequation #onedimensionalheatequation
Комментарии
 0:26:09
0:26:09
 0:21:44
0:21:44
 0:07:52
0:07:52
 0:06:18
0:06:18
 0:06:27
0:06:27
 0:12:05
0:12:05
 0:02:48
0:02:48
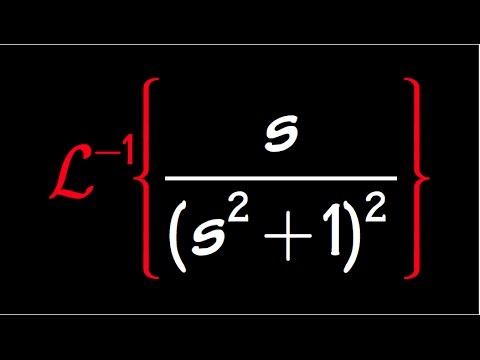 0:09:25
0:09:25
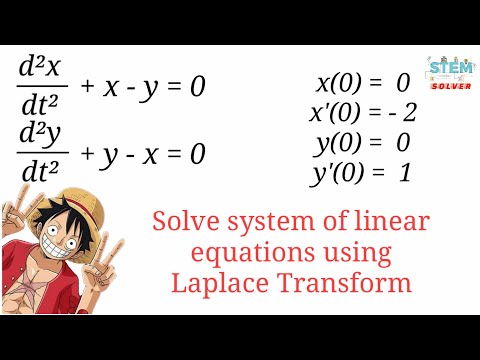 0:14:49
0:14:49
 0:04:06
0:04:06
 0:13:07
0:13:07
 0:00:35
0:00:35
 0:07:14
0:07:14
 0:12:42
0:12:42
 0:07:05
0:07:05
 0:05:31
0:05:31
 0:10:54
0:10:54
 0:27:51
0:27:51
 0:18:02
0:18:02
 0:06:26
0:06:26
 0:22:38
0:22:38
 0:09:59
0:09:59
 0:20:38
0:20:38
 0:11:56
0:11:56