Green's Function Solution to Wave Equation

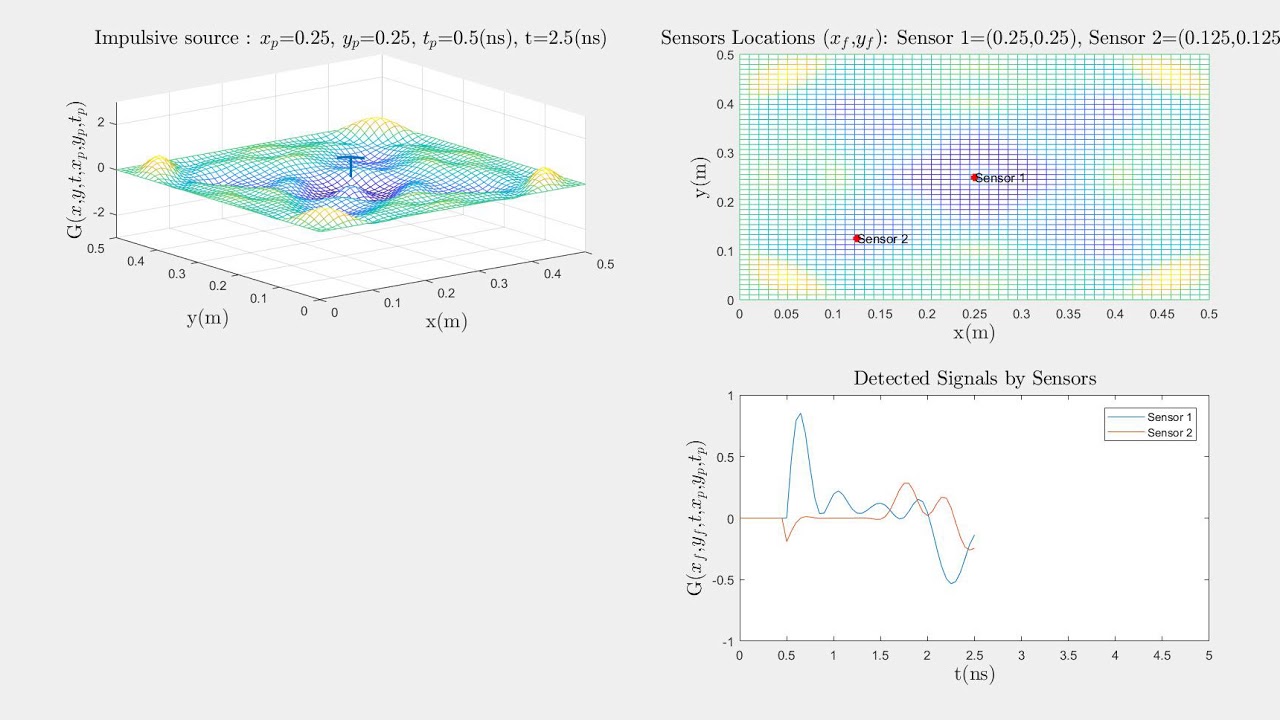
In this video the elementary solution G (known as Green's Function) to the inhomogenous scalar wave equation (∇"G+G"=δ(x-xp) δ(y-yp) δ(t-tp)) is shown:
-solution region: squared plane (0.5x0.5m);
-space-time impulsive source: δ(xp,yp,tp)=δ(0.25m,0.25m,0.5ns);
-homogenous Initial Conditions (ICs): G(x,y,t)=0 and G'(x,y,t)=0;
-homogenous Boundary Conditions (BCs): G(x,y,t)=0 on the boundary surface.

Source position and time delay can be easily set by code.

Solution approach consists of Separation of Variables, Duhamel Principle and Fourier Series concepts.

Useful References:
-Strauss, Walter A. Partial differential equations. Vol. 92. New York: Wiley, 1992.
-Zangwill, Andrew. Modern electrodynamics. Cambridge University Press, 2013.
-Jackson, John David. Classical electrodynamics. John Wiley & Sons, 2007.

Numerical Implementation is done in Matlab 2017a.