filmov
tv
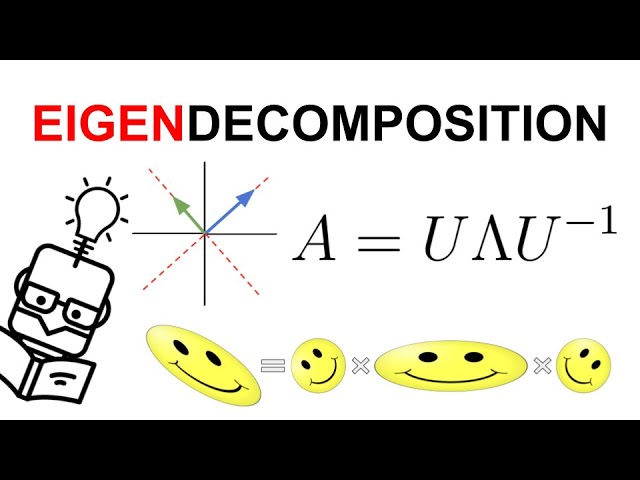
Eigendecomposition Explained
Показать описание
In this video, we explore how we can factorize a square matrix using the eigendecomposition and why this transformation can be useful when solving machine learning problems.
*References*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Related Videos*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Contents*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
00:00 - Intro
00:25 - Eigenvectors and eigenvalues
01:31 - Eigendecomposition equations
03:36 - Matrix raised to a power
05:50 - Eigendecomposition of a symmetric matrix
07:12 - Outro
*Follow Me*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Channel Support*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
The best way to support the channel is to share the content. ;)
If you'd like to also support the channel financially, donating the price of a coffee is always warmly welcomed! (completely optional and voluntary)
► Bitcoin (BTC): 3C6Pkzyb5CjAUYrJxmpCaaNPVRgRVxxyTq
► Ethereum (ETH): 0x9Ac4eB94386C3e02b96599C05B7a8C71773c9281
► Cardano (ADA): addr1v95rfxlslfzkvd8sr3exkh7st4qmgj4ywf5zcaxgqgdyunsj5juw5
► Tether (USDT): 0xeC261d9b2EE4B6997a6a424067af165BAA4afE1a
#eigendecomposition #eigenvectors #eigenvalues #linearalgebra
*References*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Related Videos*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Contents*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
00:00 - Intro
00:25 - Eigenvectors and eigenvalues
01:31 - Eigendecomposition equations
03:36 - Matrix raised to a power
05:50 - Eigendecomposition of a symmetric matrix
07:12 - Outro
*Follow Me*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
*Channel Support*
▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬
The best way to support the channel is to share the content. ;)
If you'd like to also support the channel financially, donating the price of a coffee is always warmly welcomed! (completely optional and voluntary)
► Bitcoin (BTC): 3C6Pkzyb5CjAUYrJxmpCaaNPVRgRVxxyTq
► Ethereum (ETH): 0x9Ac4eB94386C3e02b96599C05B7a8C71773c9281
► Cardano (ADA): addr1v95rfxlslfzkvd8sr3exkh7st4qmgj4ywf5zcaxgqgdyunsj5juw5
► Tether (USDT): 0xeC261d9b2EE4B6997a6a424067af165BAA4afE1a
#eigendecomposition #eigenvectors #eigenvalues #linearalgebra
0:08:51
Eigendecomposition : Data Science Basics
0:07:35
Eigendecomposition Explained
0:17:16
Eigenvectors and eigenvalues | Chapter 14, Essence of linear algebra
0:07:29
Eigendecomposition Explained !! | Tutorial 17 | Mathematics for MACHINE LEARNING
0:12:49
Eigendecomposition — Topic 33 of Machine Learning Foundations
0:01:00
Eigendecomposition Explained #machinelearning #maths #datascience #linearalgebra #eigenvectors
0:09:09
What eigenvalues and eigenvectors mean geometrically
0:02:55
Eigen values and Eigen vectors in 3 mins | Explained with an interesting analogy
0:05:29
Eigendecomposition
0:08:43
Diagonalization
0:09:46
Visualizing Diagonalization & Eigenbases
0:07:43
Introduction to eigenvalues and eigenvectors | Linear Algebra | Khan Academy
0:17:10
Finding Eigenvalues and Eigenvectors
0:04:48
Eigenvector and Eigenvalue and PCA overview
0:02:58
A 3x3 Symmetric Eigenvalue Decomposition in under 3 Minutes!
0:16:28
SVD Visualized, Singular Value Decomposition explained | SEE Matrix , Chapter 3 #SoME2
0:04:45
Singular Values vs. Eigenvalues : Data Science Basics
0:13:02
Eigenvector and Eigenvalue Applications — Topic 34 of Machine Learning Foundations
0:11:43
Eigenvalues, Eigenvectors And Eigendecomposition | Linear Algebra Explained | Simplilearn
0:11:58
Eigenvalues & Eigenvectors : Data Science Basics
0:15:02
Tutorial 24: Eigendecomposition of a matrix in Linear Algebra for Machine Learning
0:07:40
What is the Singular Value Decomposition?
0:06:44
Singular Value Decomposition (SVD): Overview
0:14:11
Singular Value Decomposition (the SVD)
Комментарии