filmov
tv
Quadratic curvature for algebraic curves (cont) | Differential Geometry 15 | NJ Wildberger
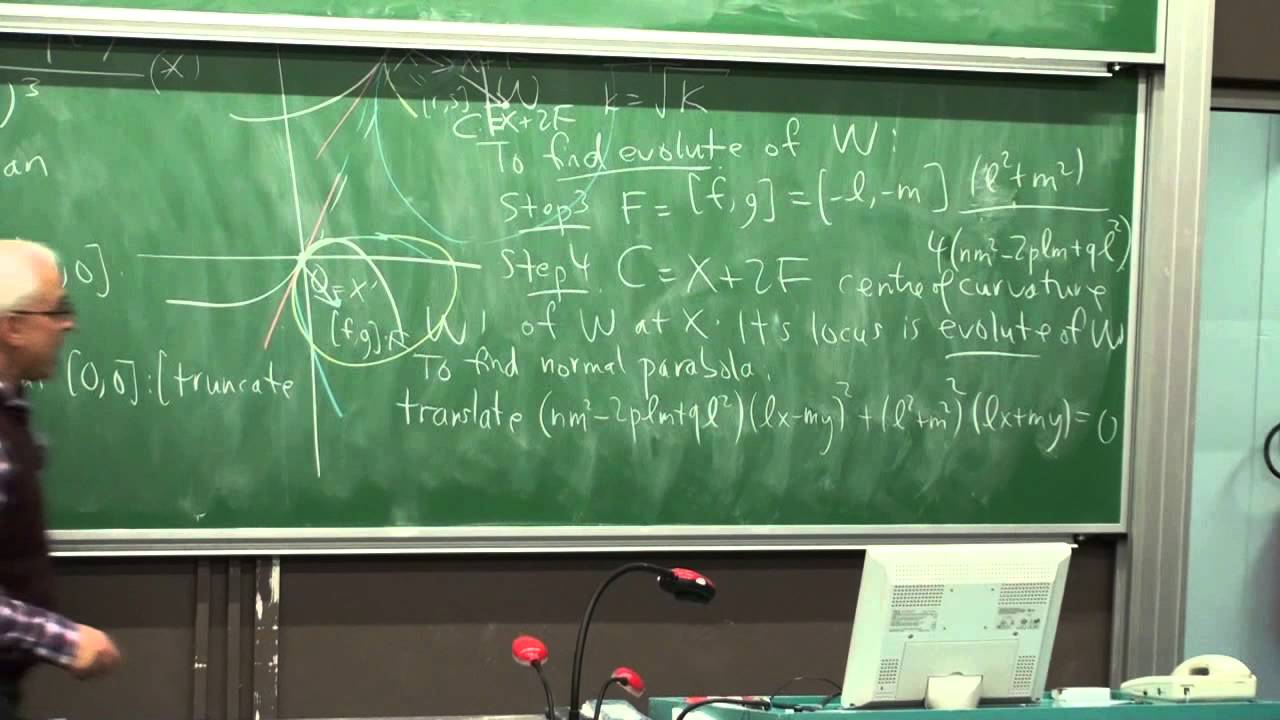
Показать описание
We continue in developing fundamental formulas that deal with curvature for surfaces in three dimensional space, given by algebraic equations. Our approach continues to be that the normal paraboloid to such a surface at a point is the key object that encodes the quadratic metrical information, including curvatures. However we want formulas that deal with the general situation, not only the simpler case when the tangent plane is horizontal.
[It appears this video has been unlisted lately, even though it is some years old! Better late than never to get it back out there ....]
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
[It appears this video has been unlisted lately, even though it is some years old! Better late than never to get it back out there ....]
************************
Here are the Insights into Mathematics Playlists:
Here are the Wild Egg Maths Playlists (some available only to Members!)
************************
Quadratic curvature for algebraic curves (cont) | Differential Geometry 15 | NJ Wildberger
Quadratic curvature for algebraic curves | Differential Geometry 14 | NJ Wildberger
AQA Core 1 3.08b Completely Sketching a Quadratic Curve
Putting Algebraic Curves in Perspective
AQA Core 1 3.08a Completely Sketching a Quadratic Curve
The Riemann-Roch theorem for algebraic curves (Part I) (6-23-2021)
The space of equations for an algebraic curve - Dhruv Ranganathan
MathLapse – Can Anybody See the Algebraic Curve’s Degree?
📊 Graphing Quadratic Equations | Intercept Method
Ye Tian: The arithmetic of quadratic twists of elliptic curves
Curve Tracing Part 4. Properties of Algebraic Curves_ Asymptotes and Holes (Functions Discontinuity)
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Parabola Concave Up Shift Up | Sketching Quadratic Graphs
How to find the Vertex of a quadratic/parabola
Quadratic Turning Point | GCSE Maths 2025
The Maths Prof: Line & Quadratic Curve Intersections
How to sketch a quadratic curve? Part 3/3
Transformation of Quadratic Graphs
Curve Tracing Part 2. Properties of Algebraic Curves_ Intercepts
CURVE SKETCHING, A QUADRATIC CURVE
How to find the equation of curve (Quadratic Chapter)
İzzet Coşkun 1 - Topics in Algebraic Curves
İzzet Coşkun 2 - Topics in Algebraic Curves
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Комментарии
 0:36:11
0:36:11
 0:33:08
0:33:08
 0:03:16
0:03:16
 0:21:39
0:21:39
 0:03:42
0:03:42
 1:39:58
1:39:58
 0:15:36
0:15:36
 0:03:31
0:03:31
 0:07:08
0:07:08
 0:45:35
0:45:35
 0:23:30
0:23:30
 0:00:15
0:00:15
 0:00:25
0:00:25
 0:00:21
0:00:21
 0:00:54
0:00:54
 0:18:28
0:18:28
 0:04:30
0:04:30
 0:00:16
0:00:16
 0:17:56
0:17:56
 0:08:43
0:08:43
 0:15:50
0:15:50
 1:28:17
1:28:17
 1:27:15
1:27:15
 0:00:38
0:00:38