filmov
tv
nested square roots

Показать описание
What is the value of sqrt(1+sqrt(1+sqrt(1+...)))? Watch this video to find out!
How to simplify nested square roots (shortcut & proof)
Nested square roots: why? when? how?
The SECRET Trick to Solving Nested Square Roots Revealed! #maths
A super fast math trick for nested square roots
nested square roots
Nested Square Roots
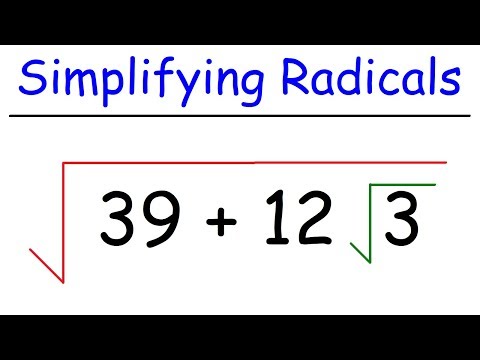
Math - Simplifying Radicals
Nested Square Root! No Calculators
How to solve radical expression with easiest method?
a nested square root!
Square roots which are nested || Solve this problem
A Nested Square Root Problem
A Special Quick and Easy Method to Simplify this Nested Square Roots| Olympiad Math
Special Nested Root (Part 1)
The Root of the Problem: Solving a Nested Square Root Equation by Applying Exponent Rules
Nested square root | √(1+2√(1+2√(1+...)))
How to Solve Nested Square Roots! #maths #shorts
Be careful with this nested square root equation
Approximate Square Roots Visually!
A very nested square root equation
An Infinite number of Square Roots!
Multiplying Square Root of Negative Numbers
NBT question on nested square roots
Nested square roots: why? when? how? USA Nice Root Simplification Radical Equation | find x
Комментарии
 0:04:54
0:04:54
 0:08:43
0:08:43
 0:00:33
0:00:33
 0:00:20
0:00:20
 0:00:27
0:00:27
 0:03:36
0:03:36
 0:10:14
0:10:14
 0:06:40
0:06:40
 0:02:58
0:02:58
 0:00:16
0:00:16
 0:01:23
0:01:23
 0:07:45
0:07:45
 0:07:40
0:07:40
 0:07:25
0:07:25
 0:03:37
0:03:37
 0:02:18
0:02:18
 0:00:32
0:00:32
 0:09:21
0:09:21
 0:01:00
0:01:00
 0:01:19
0:01:19
 0:00:54
0:00:54
 0:00:56
0:00:56
 0:02:44
0:02:44
 0:03:34
0:03:34