filmov
tv
When are F[x]-Modules Isomorphic? (Algebra 3: Lecture 2 Video 2)

Показать описание
Lecture 2: We started this lecture by recalling the process described at the end of the previous lecture that showed that a linear transformation T has a rational canonical form. We then proved that this rational canonical form is unique. We used this to answer a question about when different linear transformations S and T on the same vector space V give rise to isomorphic F[x]-modules. We showed how starting from an n x n matrix A with entries in F, we can define a linear transformation from it, which allows us to define the rational canonical form of a matrix. At the end of the lecture we discussed the situation where A is an n x n matrix with entries in a field F that is a subfield of a field K. We proved that the rational canonical form of A is the same whether we compute it over the smaller field F or over the larger field K.
Reading: In this lecture we followed part of Section 12.2, pages 475-478, very closely.
Reading: In this lecture we followed part of Section 12.2, pages 475-478, very closely.
 0:07:31
0:07:31
![When are F[x]-Modules](https://i.ytimg.com/vi/iVJob1FoRRg/hqdefault.jpg) 0:08:36
0:08:36
 0:05:04
0:05:04
 0:12:46
0:12:46
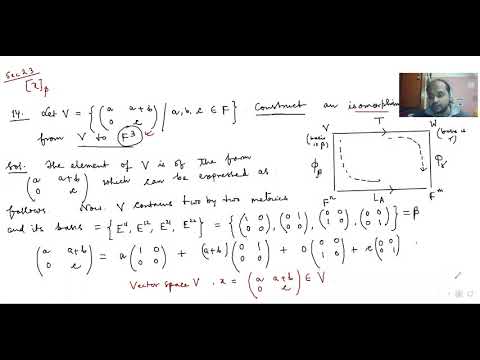 0:36:20
0:36:20
 0:14:36
0:14:36
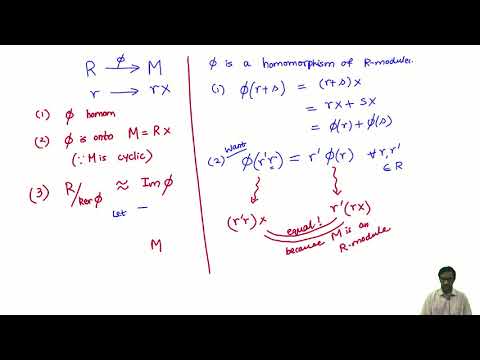 0:31:06
0:31:06
 0:07:43
0:07:43
 0:04:53
0:04:53
 0:04:45
0:04:45
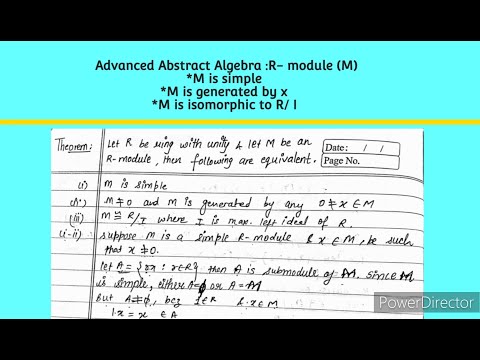 0:13:14
0:13:14
 0:15:27
0:15:27
 0:10:51
0:10:51
 0:10:04
0:10:04
 0:13:08
0:13:08
 0:15:01
0:15:01
 0:08:45
0:08:45
 0:40:10
0:40:10
 0:18:50
0:18:50
 0:22:06
0:22:06
 0:24:29
0:24:29
 0:12:30
0:12:30
 0:11:17
0:11:17
 0:07:53
0:07:53