Marissa Kawehi Loving - An intro to mapping class groups (Lecture 1)

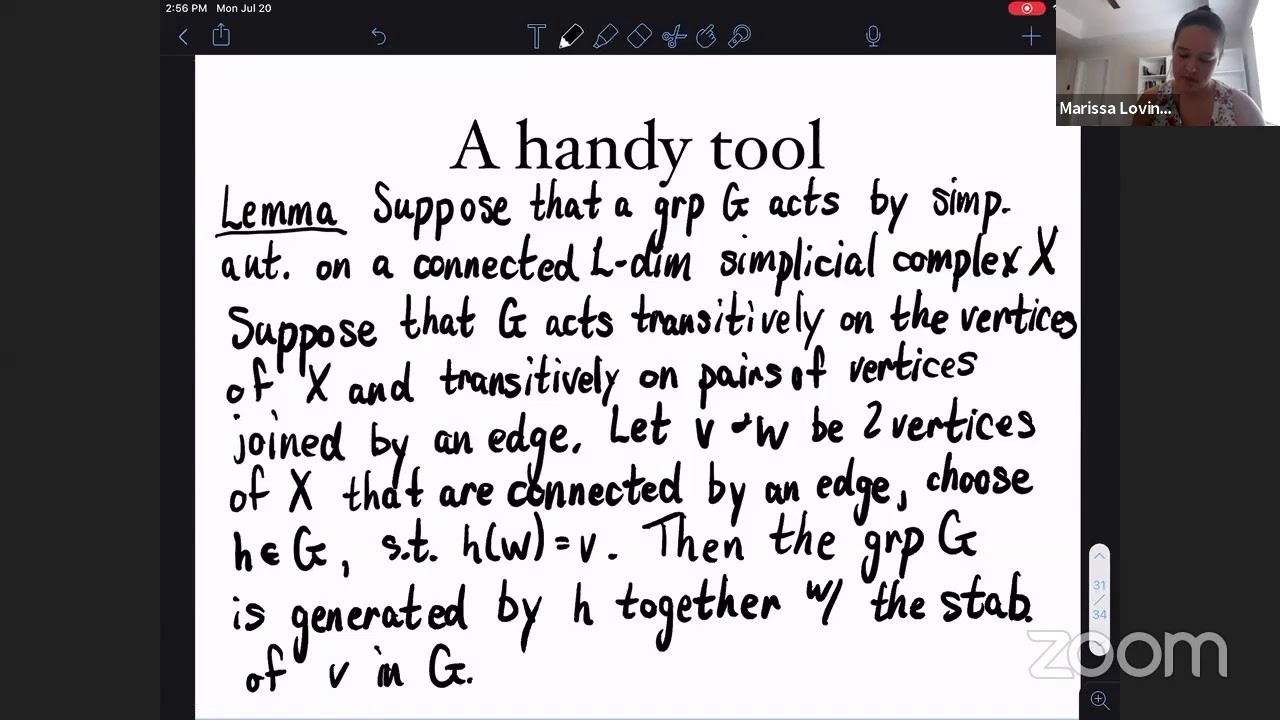
The mapping class group of a connected, oriented, finite-type surface S, denoted Mod(S), is the group of homotopy classes of orientation-preserving homeomorphisms of S. In this mini-course, we will cover some of the basics of Mod(S) such as building a generating set, producing actions of Mod(S) on various metric spaces (the curve complex, Teichmuller space, etc.), as well as building some (coarse) models for Mod(S).

In particular, we aim to build enough language and tools to give a brief overview of Masur-Minsky's hierarchy machinery by the end of the second lecture (this will hopefully give a good jumping off point for Davide's lectures on HHG's). Time allowing, in the final lecture, we will explore "big" mapping class groups of infinite-type surfaces, which is an area of increasing interest and is full of open questions and directions.