Legendre's Differential Equation Full Solution!

Hi! Today we are going to solve a really important and famous differential equation in physics and mathematics, Legendre's ODE.

Recommendation: Put the video at speed x2, it is a lot faster and easy to follo

As you see, the video is really long, but don't worry, the ODE is not too hard! The reason for the length of the video is that there are many details I wanted to explain in depth, but of course, as always, here you have the time stamps so you can navigate the video faster and go directly to what you need or want to see:

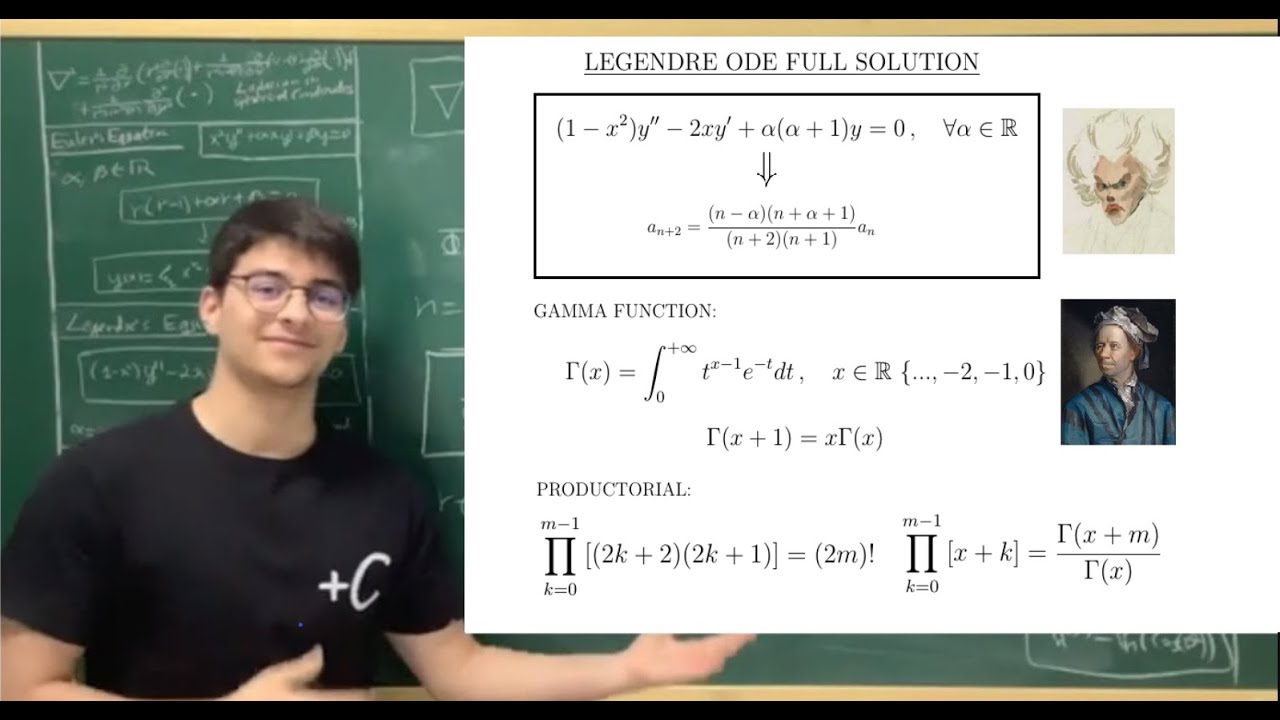
0:00 Introduction
0:40 Gamma Function and its Properties
2:36 Frobenius Method
3:36 Productorial Identities
3:56 We can start solving the equation
3:57 1. Ordinary Point x_0 = 0 and radius of convergence
6:56 2. Values of the parameter alpha
10:00 3. We propose a solution in a Series Expansion
24:44 4. Recurrence Relation/Difference Equation (Here is where the video really starts!)
29:30 5. Even terms n = 2m: a_2m
40:44 5.1. Restriction 1 on alpha
43:56 5.2. Restriction 2 on alpha
46:31 5.2. Explanation of the Restricitons: Alpha is not even or -1
51:28 5.3. Expression if alpha is even or -1
56:18 5.4. Recap: Solution if n is even: Expression for a_(2m)
57:34 6. Odd terms n = 2m + 1: a_(2m + 1)
1:01:50 6.1. Restriction 1 on alpha
1:04:30 6.2. Restriction 2 on alpha
1:05:30 6.3. Explanation of the Restrictions: Alpha is not odd
1:09:34 6.4. Expression if alpha is odd
1:11:00 7. We divide the general solution into a sum of even and odd terms: a_(2m) and a_(2m + 1)
1:19:45 8. We consider first the case in which alpha is not an integer
1:25:15 9. General solution if alpha is not an integer
1:29:44 10. General solution if alpha is an integer
1:30:33 11. Alpha is an integer: Many terms cancel out and we get finite polynomials
1:34:00 11.1. Alpha is even: The even degree polynomial is finite
1:35:30 11.2. Alpha is odd: The odd degree polynomial is finite
1:37:36 11.3. Recap: We get two finite polynomials if alpha is an integer
1:38:00 12. Final Result: General Solution of the Equation!
1:38:26 Summary of the problem
1:42:47 Legendre Polynomials

Another important aspect about this ODE, is that from it we can get the Legendre Polynomials, really important for both, physics and mathematics. These polynomials can also be generated in other ways different from this equation, and we will see that in other videos.

I hope you enjoyed the video and see you in the next one!

[tavochido]

you basically saved my life haha! There is no video on youtube that explains the solution to Legendre’s equation and goes all the way down to the gamma function part. I’m a sophomore at tsinghua university!

[tavochido]

do you have an email? And btw do you speak spanish haha