Marc Levine: Atiyah-Bott localization for Witt sheaf cohomology, with applications

30 September 2021

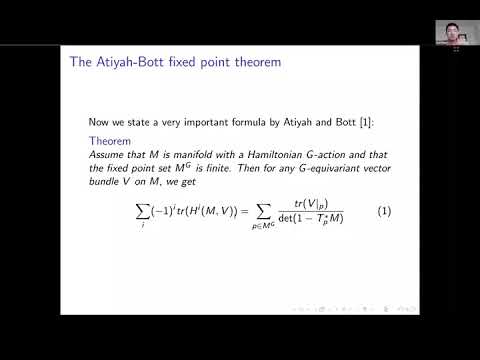
Abstract: Atiyah-Bott localization for singular cohomology of a space with a torus action has proven to be an effective tool in many areas, including enumerative geometry. We give here a parallel for cohomology with Witt-sheaf coeffcients, which is useful for computing quadratic renements of classical numerical invariants. Since the torus-equivariant Witt-sheaf cohomology is the same as the non-equivariant cohomology, there is no parallel theory for torus actions. We describe a version for schemes with an SLn2^n or N^n-action, where N is the normalizer of the torus in SL2. As an application, we compute (joint with Sabrina Pauli) a quadratic count of cubic rational curves on certain smooth hypersurfaces or complete intersections in a projective space; the first example being the case of a quintic hypersurface.

---------------------------------------------------------------------------------------------------------
Representation theory’s hidden motives
27 September – 1 October 2021