filmov
tv
A Nice Algebra Equation | Math Olympiad Problem
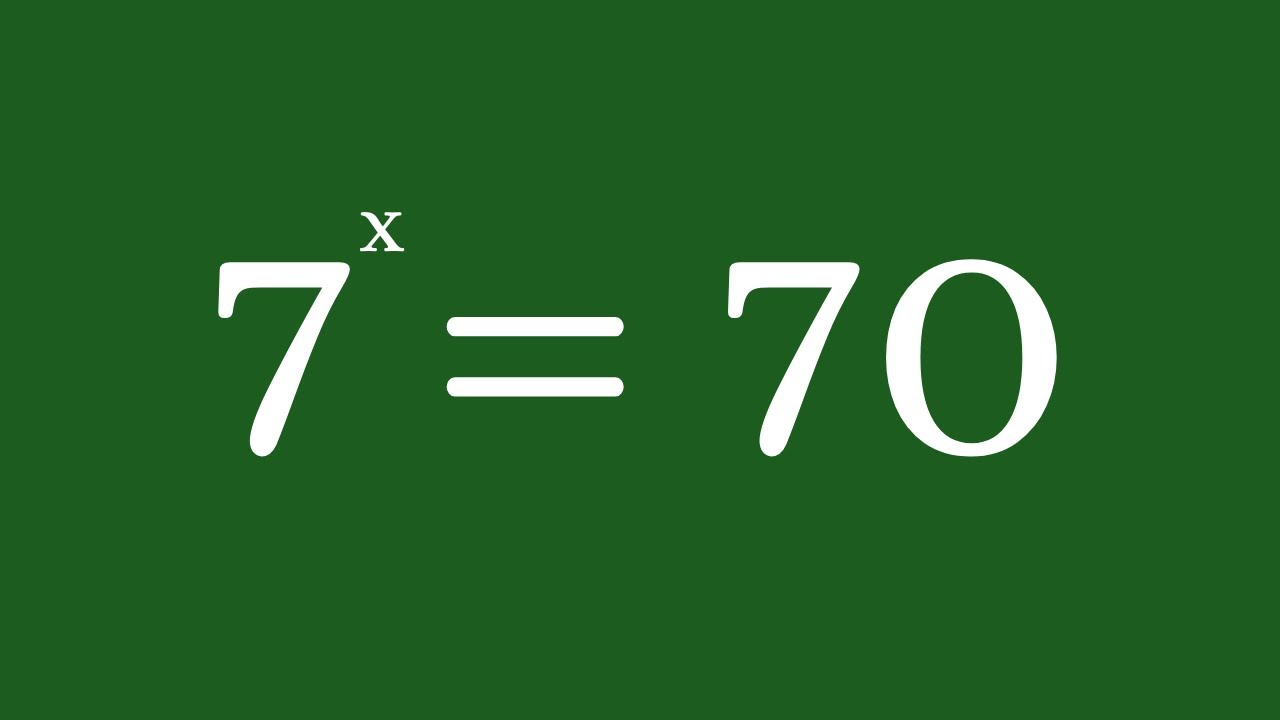
Показать описание
In todays video, I will be teaching you how to solve an interesting problem. Make sure to like, subscribe, and also comment any questions or video ideas you may have relating to math!
#maths #algebra #mathematics #mathematician #mathematicians #algebra #algebratricks #algebraformulas #algebrahelp #olimpiadas #olimpiadmath #algebraquestion #algebraquestions #geometry #easymaths #easymathematics #easymathematicsforyou #multiplication #division #fraction #product #laws #lawsofexponent #howtolearnmathsfromthebeginning #howtolearnmathsfrombasics #howtolearnmaths #simplemathstricks #simplemathematics #simplemath #algebrahelp #simplealgebra#relaxingmath#howtosolvepotentialequations#potentialequations#solveforx#mathssolving#exponents #equations #exponentialequation #exponentialequations #algebrahelp #algebraformulas #algebratrick #highermathematics #highermaths #highermath#programming #programmer#sinus#sinx#cosx#findthevalueof
#powerdivision#olympiadalgebra#olympiadclass8
#maths #algebra #mathematics #mathematician #mathematicians #algebra #algebratricks #algebraformulas #algebrahelp #olimpiadas #olimpiadmath #algebraquestion #algebraquestions #geometry #easymaths #easymathematics #easymathematicsforyou #multiplication #division #fraction #product #laws #lawsofexponent #howtolearnmathsfromthebeginning #howtolearnmathsfrombasics #howtolearnmaths #simplemathstricks #simplemathematics #simplemath #algebrahelp #simplealgebra#relaxingmath#howtosolvepotentialequations#potentialequations#solveforx#mathssolving#exponents #equations #exponentialequation #exponentialequations #algebrahelp #algebraformulas #algebratrick #highermathematics #highermaths #highermath#programming #programmer#sinus#sinx#cosx#findthevalueof
#powerdivision#olympiadalgebra#olympiadclass8
Germany| A Nice Exponential Equation. Can you Solve? #algebra
Japanese || A Nice Exponential Algebra Problem || Math Olympiad🖊️📘
Math Olympiad Question | Nice Algebra Equation | You should know this trick!!
Algebra Basics: Solving 2-Step Equations - Math Antics
Algebra - How To Solve Equations Quickly!
Algebra Basics: What Is Algebra? - Math Antics
A Nice Algebra Problem | Math Olympiad | Find all roots?
Algebra Basics: Solving Basic Equations Part 2 - Math Antics
Germany ┃Can you Solve This ? A Nice Algebra Problem┃ Math Olympiad ┃ Math Olympiad
Germany | Can You Solve This? | A Nice Olympiad Algebra Problem | Best Trick
Algebra Basics - Solving Basic Equations - Quick Review!
Math Olympiad Question | Nice Algebra Problem | You should Learn This Trick
A Nice Math Olympiad Algebra Problem | How to solve for 'b' ?
A Nice Algebra Problem | Math Olympiad | Solve for a , b ?
Algebra Basics: Solving Basic Equations Part 1 - Math Antics
Algebra: FOIL Method #Shorts #algebra #math #maths #mathematics #education #learn
Algebra Shortcut Trick - how to solve equations instantly
Poland | A Nice Algebra Math Simplification | Math Olympiad
Solving Two-Step Equations | Algebra Equations
A Nice Algebra Problem | Math Olympiad | How to solve for x?
France ┃A Nice Algebra Problem ┃ Math Olympiad ┃ Math problems
Algebra for Beginners | Basics of Algebra
A Nice Algebra Problem | Math Olympiad | Solve for all x value?
A Nice Algebra Math Simplification || Find the Value of K || #maths #education #algebra #mathematics
Комментарии
 0:11:55
0:11:55
 0:08:07
0:08:07
 0:07:26
0:07:26
 0:10:29
0:10:29
 0:25:05
0:25:05
 0:12:07
0:12:07
 0:12:50
0:12:50
 0:09:35
0:09:35
 0:05:02
0:05:02
 0:09:56
0:09:56
 0:11:24
0:11:24
 0:10:54
0:10:54
 0:09:33
0:09:33
 0:13:09
0:13:09
 0:11:08
0:11:08
 0:00:18
0:00:18
 0:10:14
0:10:14
 0:05:49
0:05:49
 0:09:13
0:09:13
 0:14:52
0:14:52
 0:06:31
0:06:31
 0:37:09
0:37:09
 0:14:31
0:14:31
 0:07:02
0:07:02