filmov
tv
Standard Error (of the sample mean) | Sampling | Confidence Intervals | Proportions

Показать описание
0:00 Introduction
1:20 Definition
6:40 Confidence Intervals
12:51 Proportions
17:16 Challenge Question
Series music by Purdy.
Song: 3 Friends to the Stars
Standard Error (of the sample mean) | Sampling | Confidence Intervals | Proportions
8. Sampling and Standard Error
What Is a Standard Error?
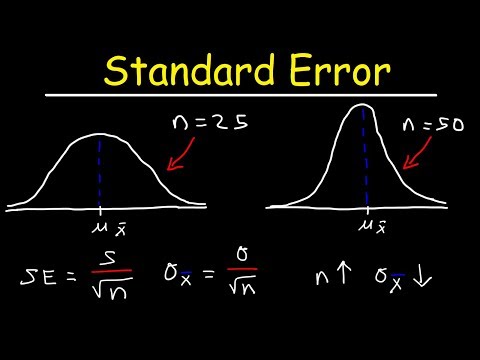
Standard Error of the Mean
Standard Error
Understanding Standard Error
Estimated Standard Error : One Sample
Standard Deviation Formula, Statistics, Variance, Sample and Population Mean
MAT 144 Topic 5 prob empirical rule and norm dist
Simplest Explanation of the Standard Errors of Regression Coefficients - Statistics Help
Sampling Distribution & Standard Error
standard error of proportion
Finding Sample Size, Given Standard Deviation and Standard error of the mean.
Sampling Distributions (7.2)
How To Calculate Sample Standard Deviation (Step By Step)
How to Use Z Score and Standard Error to Determine Sample Probability in Statistics
Chapter 7 Sample Size and Standard Error
Standard Error of the Mean in Excel
04 Effect of Sample Size on Standard Error
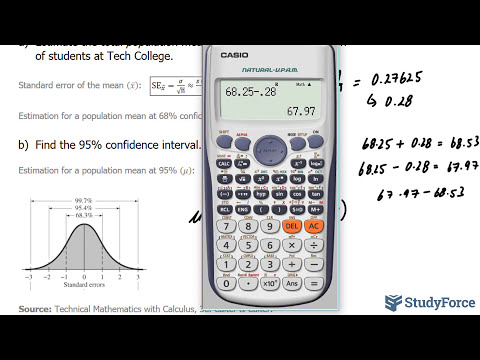
📚 How to find the standard error of a sample mean to estimate the population mean
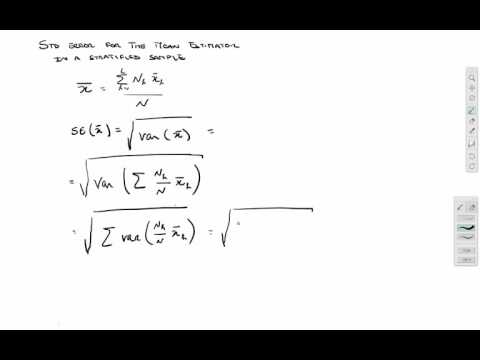
Standard Error for the mean of a stratified sample
What is sampling error?
Standard Error of the Mean: Concept and Formula | Statistics Tutorial #6 | MarinStatsLectures
Sample standard deviation and bias | Probability and Statistics | Khan Academy
Комментарии
 0:18:40
0:18:40
 0:46:45
0:46:45
 0:02:17
0:02:17
 0:10:45
0:10:45
 0:07:05
0:07:05
 0:05:01
0:05:01
 0:07:22
0:07:22
 0:10:21
0:10:21
 0:20:16
0:20:16
 0:04:07
0:04:07
 0:06:25
0:06:25
 0:03:38
0:03:38
 0:03:30
0:03:30
 0:11:06
0:11:06
 0:02:06
0:02:06
 0:06:24
0:06:24
 0:04:43
0:04:43
 0:03:09
0:03:09
 0:08:32
0:08:32
 0:05:06
0:05:06
 0:04:16
0:04:16
 0:02:49
0:02:49
 0:05:25
0:05:25
 0:09:32
0:09:32