filmov
tv
Discrete Math - 9.1.2 Properties of Relations

Показать описание
Exploring the properties of relations including reflexive, symmetric, anti-symmetric and transitive properties.
Video Chapters:
Introduction 0:00
Reflexive Relations 0:07
Symmetric Relations 1:56
Anti-Symmetric Relations 4:11
Transitive Relations 9:27
Relation Properties Practice 14:55
Up Next 21:35
Textbook: Rosen, Discrete Mathematics and Its Applications, 7e
Video Chapters:
Introduction 0:00
Reflexive Relations 0:07
Symmetric Relations 1:56
Anti-Symmetric Relations 4:11
Transitive Relations 9:27
Relation Properties Practice 14:55
Up Next 21:35
Textbook: Rosen, Discrete Mathematics and Its Applications, 7e
Discrete Math - 9.1.2 Properties of Relations
Discrete Math - 9.1.1 Introduction to Relations
Types of Relations (Part 1)
RELATIONS - DISCRETE MATHEMATICS
Properties of Relation - Relation- Discrete Mathematics
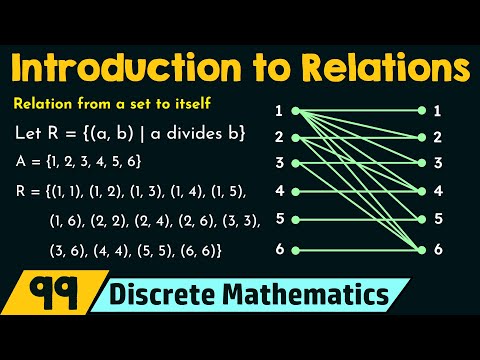
Introduction to Relations
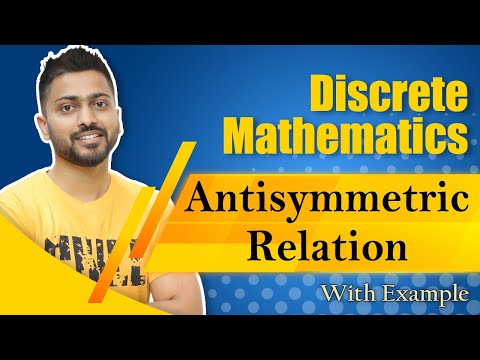
Antisymmetric Relation with examples | Discrete Maths
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
Conditional Statements: if p then q
Representation of Relations
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
Relations||How to check relation is reflexive, symmetric or transitive?
Equivalence Relation
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
L-2.2: Reflexive Relation with examples | Discrete Mathematics
Discrete Random Variables The Expected Value of X and VarX
Solving congruences, 3 introductory examples
Closure Properties Reflexive and Symmetric Closure - Relation - Discrete Mathematics
6÷2(1+2)=???
How to eat Roti #SSB #SSB Preparation #Defence #Army #Best Defence Academy #OLQ
truth values and tables in fuzzy logic | truth values and truth tables | class 12 in hindi |shortcut
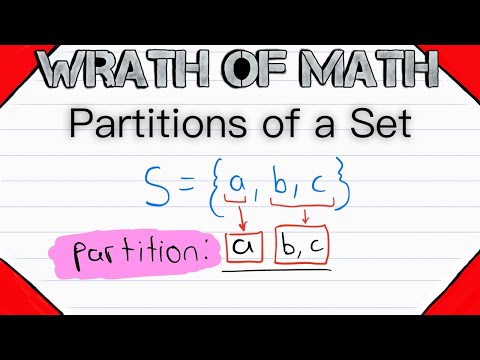
Partitions of a Set | Set Theory
Introduction to Partial Ordering
Cartesian Product
Комментарии
 0:21:40
0:21:40
 0:10:28
0:10:28
 0:06:39
0:06:39
 0:15:36
0:15:36
 0:22:13
0:22:13
 0:07:39
0:07:39
 0:06:54
0:06:54
 0:05:45
0:05:45
 0:07:09
0:07:09
 0:05:51
0:05:51
 0:00:16
0:00:16
 0:00:51
0:00:51
 0:06:29
0:06:29
 0:00:38
0:00:38
 0:11:41
0:11:41
 0:05:33
0:05:33
 0:03:51
0:03:51
 0:07:24
0:07:24
 0:01:21
0:01:21
 0:00:16
0:00:16
 0:03:31
0:03:31
 0:07:59
0:07:59
 0:15:34
0:15:34
 0:07:52
0:07:52